- #1
You are using an out of date browser. It may not display this or other websites correctly.
You should upgrade or use an alternative browser.
You should upgrade or use an alternative browser.
Struggling with Moduli in Complex Numbers?
- I
- Thread starter SamitC
- Start date
In summary, there is confusion about an example from the Brown-Churchill book, with a possible typo in the equation reference. The triangle inequality is applied to the equation and it is simplified to show it is less than 25. The conversation ends with a thank you for the clarification.
Physics news on Phys.org
- #2
- 4,133
- 1,734
I think you may have copied the wrong equation for (9). The triangle inequality says that ##|a+b|\leq|a|+|b|##. Applying that to your equation we get:
$$|z^3+3z^2-2z+1|\leq|z^3+3z^2-2z|+|1|\leq|z^3+3z^2|+|-2z|+|1|\leq|z^3|+|3z^2|+|-2z|+|1|$$
[applying the triangle inequality three times in succession]
$$=|z|^3+3|z|^2+|-2||z|+1$$
[applying (8) ]
$$=|z|^3+3|z|^2+2|z|+1<2^3+3\cdot 2^2+2\cdot 2+1=25$$
EDIT: Just saw Samy's post. I don't have the book but, based on that picture, it looks more likely a typo in that the ref to (9) should be to (10), rather than you miscopying it.
$$|z^3+3z^2-2z+1|\leq|z^3+3z^2-2z|+|1|\leq|z^3+3z^2|+|-2z|+|1|\leq|z^3|+|3z^2|+|-2z|+|1|$$
[applying the triangle inequality three times in succession]
$$=|z|^3+3|z|^2+|-2||z|+1$$
[applying (8) ]
$$=|z|^3+3|z|^2+2|z|+1<2^3+3\cdot 2^2+2\cdot 2+1=25$$
EDIT: Just saw Samy's post. I don't have the book but, based on that picture, it looks more likely a typo in that the ref to (9) should be to (10), rather than you miscopying it.
- #3
Samy_A
Science Advisor
Homework Helper
- 1,242
- 510
May well be a typo. He is referring to the following:
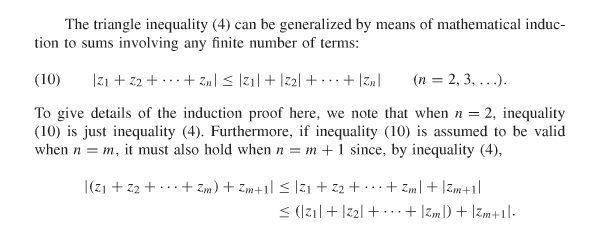
- #4
SamitC
- 36
- 0
Thank you very much.andrewkirk said:I think you may have copied the wrong equation for (9). The triangle inequality says that ##|a+b|\leq|a|+|b|##. Applying that to your equation we get:
$$|z^3+3z^2-2z+1|\leq|z^3+3z^2-2z|+|1|\leq|z^3+3z^2|+|-2z|+|1|\leq|z^3|+|3z^2|+|-2z|+|1|$$
[applying the triangle inequality three times in succession]
$$=|z|^3+3|z|^2+|-2||z|+1$$
[applying (8) ]
$$=|z|^3+3|z|^2+2|z|+1<2^3+3\cdot 2^2+2\cdot 2+1=25$$
EDIT: Just saw Samy's post. I don't have the book but, based on that picture, it looks more likely a typo in that the ref to (9) should be to (10), rather than you miscopying it.
- #5
SamitC
- 36
- 0
Thank you very much.Samy_A said:May well be a typo. He is referring to the following:
View attachment 99135
FAQ: Struggling with Moduli in Complex Numbers?
What are complex numbers moduli?
Complex numbers moduli are the absolute values or magnitudes of complex numbers. They represent the distance of a complex number from the origin on the complex plane.
How do you calculate the modulus of a complex number?
The modulus of a complex number is calculated by taking the square root of the sum of the squares of the real and imaginary parts of the number. In other words, it is the distance formula in the complex plane.
What is the significance of complex numbers moduli in mathematics?
Complex numbers moduli are important in many areas of mathematics, including calculus, geometry, and number theory. They allow us to work with complex numbers and perform operations such as addition, subtraction, multiplication, and division.
What is the relationship between a complex number's modulus and its conjugate?
The modulus of a complex number and its conjugate are equal. This means that the distance from the origin to a complex number and its conjugate on the complex plane is the same, even though they may have different real and imaginary parts.
Are there any real-world applications of complex numbers moduli?
Yes, complex numbers moduli have many real-world applications, including in electrical engineering, quantum mechanics, and signal processing. They are also used in computer graphics to represent rotations and transformations in 2D and 3D space.
Similar threads
- Replies
- 12
- Views
- 1K
- Replies
- 4
- Views
- 1K
- Replies
- 11
- Views
- 2K
- Replies
- 4
- Views
- 2K
- Replies
- 1
- Views
- 3K
- Replies
- 1
- Views
- 1K
- Replies
- 4
- Views
- 1K
- Replies
- 2
- Views
- 2K
- Replies
- 2
- Views
- 1K
- Replies
- 7
- Views
- 2K
Share:
