How Do I Solve a Complex Double Integral Problem?

[etf]

Here is my task and attempt for solution:

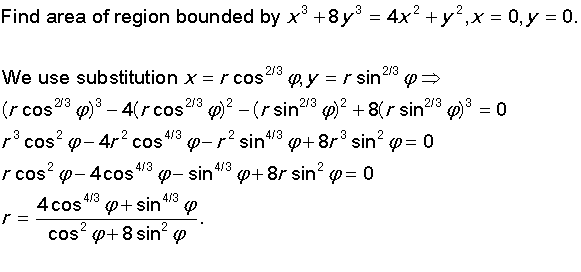
As you can see, I tried with substitutions but I have no idea what to do next.
Any suggestion?

 

[haruspex]

The equation you start with looks nasty. Are you sure it's not supposed to be x³+8y³=x²+4y², or somesuch?
Either way, I can't see the merit of that substitution. Why not just go to the usual polar coordinates? At least you can get as far as writing the area as a single integral. Still tough though.

 

[etf]

I'm sure it's how I wrote it... I tried usual polar coordinates too and I got that r=(4*cos(phi)^2+sin(phi)^2)/(cos(phi)^3+8*sin(phi)^3). I don't think that this substitution is more useful than previous. I tried with few other substitutions in order to make region easier but without success. I really have no idea what to do here :(

 

[haruspex]

I'm sure it's how I wrote it... I tried usual polar coordinates too and I got that r=(4*cos(phi)^2+sin(phi)^2)/(cos(phi)^3+8*sin(phi)^3). I don't think that this substitution is more useful than previous. I tried with few other substitutions in order to make region easier but without success. I really have no idea what to do here :(

I agree it's still nasty, but at least you can write out the integral for the area now . With your other substitution you'd have to calculate quite a messy Jacobian to get that far. (Did you compute the Jacobian? Maybe it simplifies magically, but I doubt it.)

 

[haruspex]

I've had maybe a better idea. Try u = x, v = y/x.

 

[etf]

Wow! This one is key to my problem :)
If I didn't make mistake somewhere, area should be:

 

[haruspex]

Looks good until you get to the last line. A = ∫∫dxdy = ∫∫Jdudv etc. I'm afraid it's going to get quite complicated, but it's all doable.

 

[etf]

I computed it using Matlab, I didn't try to do it by hand...
Thanks a lot!

 

[haruspex]

I computed it using Matlab, I didn't try to do it by hand...
Thanks a lot!

OK, I see - I misread your double integral as two separate integrals. (That's not the way I write them.)
The simplicity of the answer does suggest we've missed a trick somewhere.