- #1
Math Amateur
Gold Member
MHB
- 3,998
- 48
I am reading Houshang H. Sohrab's book: "Basic Real Analysis" (Second Edition).
I am focused on Chapter 2: Sequences and Series of Real Numbers ... ...
I need help with another issue/problem with Theorem 2.1.45 concerning the Supremum Property (AoC), the Archimedean Property, and the Nested Intervals Theorem ... ...
Theorem 2.1.45 reads as follows:
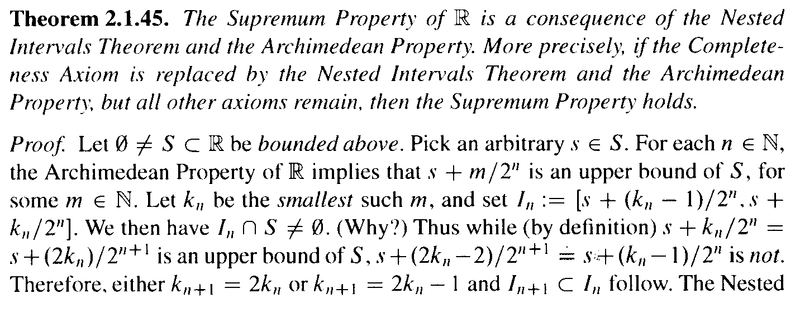
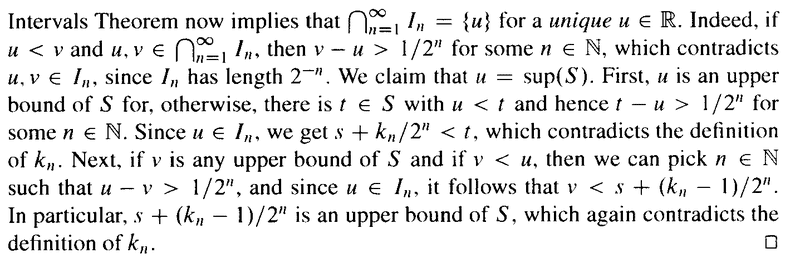 In the above proof by Sohrab, we read the following:
In the above proof by Sohrab, we read the following:
" ... ... Thus while (by definition) ##s + \frac{k_n}{2^n} = s + \frac{2 k_n}{ 2^{n + 1} }## is an upper bound of ##S, s + \frac{ 2 k_n - 2 }{ 2^{n + 1} } = s + \frac{k_n - 1 }{2^n}## is not. Therefore, either ##k_{ n+1 } = 2k_n## or ##k_{ n+1 } = 2k - 1## and ##I_{ n + 1 } \subset I_n## follow. ... ... "I am uncertain and somewhat confused by the logic of the above ...
I am not sure of the argument that either ##k_{ n+1 } = 2k_n## or ##k_{ n+1 } = 2k - 1## ... can someone please explain in simple terms why it is valid ... ..
I am also not sure exactly why ##I_{ n + 1 } \subset I_n## ... if anything it seems to me that ##I_{ n + 1 } = I_n## ... again, can someone please explain in simple terms why ##I_{ n + 1 } \subset I_n## ... that is that ##I_{ n + 1 }## is a proper subset of ##I_n## ... ...
[ ***EDIT*** Just checked and found that Sohrab is using \subset in the sense which includes equality ... so using ##\subset## as meaning ##\subseteq## ... ]Help will be appreciated ...
Peter
==========================================================================================The above theorem concerns the Supremum Property, the Archimedean Property and the Nested Intervals Theorem ... so to give readers the context and notation regarding the above post I am posting the basic information on these properties/theorems ...


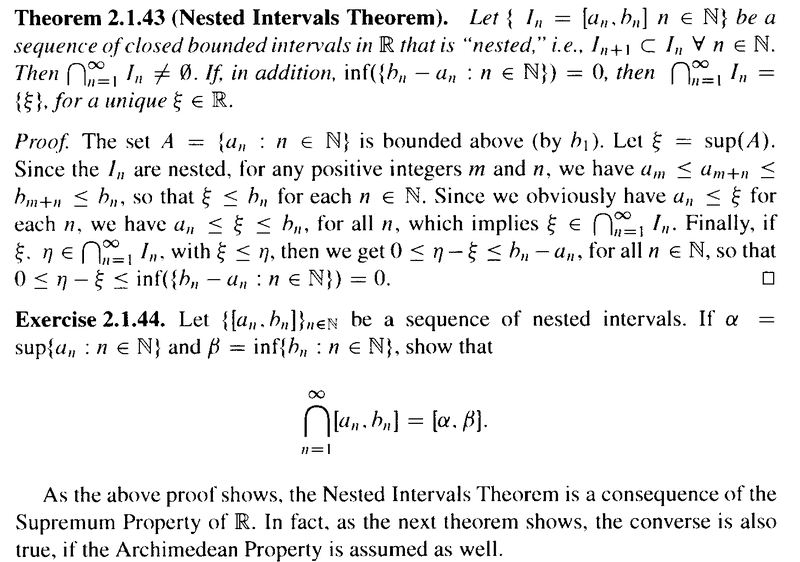
I am focused on Chapter 2: Sequences and Series of Real Numbers ... ...
I need help with another issue/problem with Theorem 2.1.45 concerning the Supremum Property (AoC), the Archimedean Property, and the Nested Intervals Theorem ... ...
Theorem 2.1.45 reads as follows:
" ... ... Thus while (by definition) ##s + \frac{k_n}{2^n} = s + \frac{2 k_n}{ 2^{n + 1} }## is an upper bound of ##S, s + \frac{ 2 k_n - 2 }{ 2^{n + 1} } = s + \frac{k_n - 1 }{2^n}## is not. Therefore, either ##k_{ n+1 } = 2k_n## or ##k_{ n+1 } = 2k - 1## and ##I_{ n + 1 } \subset I_n## follow. ... ... "I am uncertain and somewhat confused by the logic of the above ...
I am not sure of the argument that either ##k_{ n+1 } = 2k_n## or ##k_{ n+1 } = 2k - 1## ... can someone please explain in simple terms why it is valid ... ..
I am also not sure exactly why ##I_{ n + 1 } \subset I_n## ... if anything it seems to me that ##I_{ n + 1 } = I_n## ... again, can someone please explain in simple terms why ##I_{ n + 1 } \subset I_n## ... that is that ##I_{ n + 1 }## is a proper subset of ##I_n## ... ...
[ ***EDIT*** Just checked and found that Sohrab is using \subset in the sense which includes equality ... so using ##\subset## as meaning ##\subseteq## ... ]Help will be appreciated ...
Peter
==========================================================================================The above theorem concerns the Supremum Property, the Archimedean Property and the Nested Intervals Theorem ... so to give readers the context and notation regarding the above post I am posting the basic information on these properties/theorems ...
Attachments
-
 Sohrab - 1 - Theorem 2.1.45 ... - PART 1 ... ....png37.9 KB · Views: 538
Sohrab - 1 - Theorem 2.1.45 ... - PART 1 ... ....png37.9 KB · Views: 538 -
 Sohrab - 2 - Theorem 2.1.45 ... - PART 2 ... ....png29.9 KB · Views: 496
Sohrab - 2 - Theorem 2.1.45 ... - PART 2 ... ....png29.9 KB · Views: 496 -
 Sohrab - Axiom of Completeness ... Supremum Property ....png31.8 KB · Views: 551
Sohrab - Axiom of Completeness ... Supremum Property ....png31.8 KB · Views: 551 -
 Sohrab - Theorem 2.1.31 - Archimedean Property ... ....png28.3 KB · Views: 732
Sohrab - Theorem 2.1.31 - Archimedean Property ... ....png28.3 KB · Views: 732 -
 Sohrab - Theorem 2.1.43 ... Nested Intervals Theorem ....png48 KB · Views: 503
Sohrab - Theorem 2.1.43 ... Nested Intervals Theorem ....png48 KB · Views: 503
Last edited: