- #1
chetzread
- 801
- 1
in part b , we can find mass by density x area ?
is it because of the thin plate, so, the thickness of plate can be ignored?
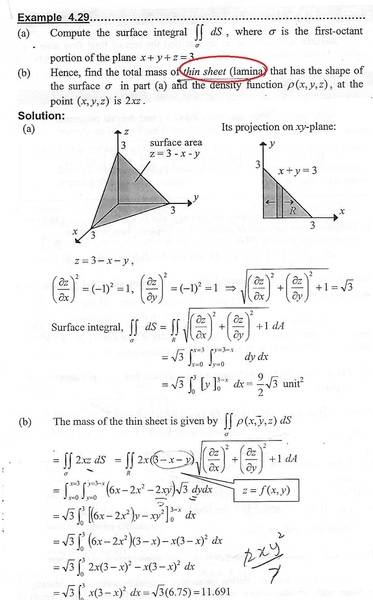
is it because of the thin plate, so, the thickness of plate can be ignored?