Symmetrical component example: can't understand solution

[ffp]

Ok, now I'm studying symmetrical components. Im using Stevenson's Power System Analysis book and example 11.9 asks for the zero sequence circuit of the example 6.1 in the same book.

Here's the example and the solution:

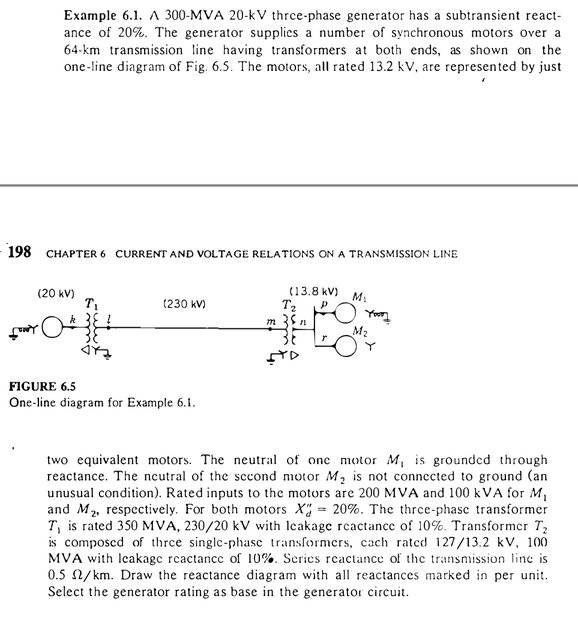
Here's example 6.1, so we can see what is the circuit:

Here's the beggining of the solution, where I'm stuck...

I believe de X of T1 (0.0857 pu) is found by changing bases (Xpu=OLDpu x OLDbase/NEWbase). So 0.1 is the pu of T1, 300 is the Sbase (MVA) of the generator (and hence, system) and 350 is the Sbase (MVA) of T1. Is that right?

If so, why is X of T2 calculated differently, I believe by reflecting impedances? If that's really what he's doing, why is he using 13.2 and 13.8?

I'm really lost here with this example.

 

[cnh1995]

I believe de X of T1 (0.0857 pu) is found by changing bases (Xpu=OLDpu x OLDbase/NEWbase). So 0.1 is the pu of T1, 300 is the Sbase (MVA) of the generator (and hence, system) and 350 is the Sbase (MVA) of T1. Is that right?

Yes. Both T1 and the generator have the same voltage rating(and are at the same voltage level in the line diagram). This is why only the ratio of MVA ratings is used to find the new pu reactance.

If so, why is X of T2 calculated differently

The MVA rating of T2 is same as the base MVA (300MVA). The 0.1 pu reactance of T2 is w.r.t its own ratings (300MVA, 13.2kV). To convert it properly according to the motor citcuit voltage level, it is divided by the motor circuit voltage level (and then squared).