Symmetries of particle interacting with external fields (Ballentine)

[EE18]

I am following along with Ballentine's (in his *Quantum Mechanics: A Modern Development*) construction/identification of symmetry generators as operators representing the standard observables (observables here being used in the sense of a physical concept which have operators representing them) and I find myself a bit lost in the argument (I have attached the full argument at the end here in case .

My confusion is as follows. In the two cases involving isolated particles before this step, Ballentine uses that an isolated particle is invariant under the full set of Galilean symmetries. This invariance under the full set of Galilean symmetries implied some specific commutators which were then used in the construction of the operators ($\textbf{J},\textbf{G}, \textbf{P}, H$) representing key observables. Ballentine then goes on to say that in the case of a particle interacting with external fields we can no longer keep the commutators involving $H$. My question has to do with this last statement:
1) Where does it sneak that the particle is isolated in developing the commutators between $\textbf{J},\textbf{G}, \textbf{P}, H$? It seems like these commutators simply emerge from observing that there is a correspondence (homomorphism) between Galilean transformations on spacetime and on $\mathcal{H}$ which we enforce and thus arrive at the commutators? Nowhere I don't think do we say that the physics is invariant under these transformations? As far as I can tell we only enforce that when we talk about how the position operator $\textbf{Q}$ transforms. (This part of the question is not related to what's pictured. Hopefully I've given a sufficiently self-contained explanation of my issue though.)
2) Why can't we keep the commutators involving $H$ when the interactions are time-independent?

I think both of these questions may have something to do with Ballentine's paragraph beginning "One may ask..." but I'm not entirely sure I follow that.

 

[strangerep]

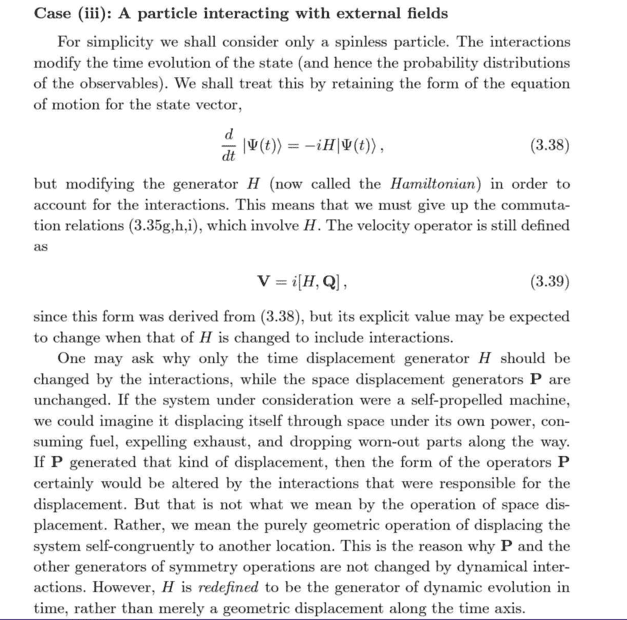
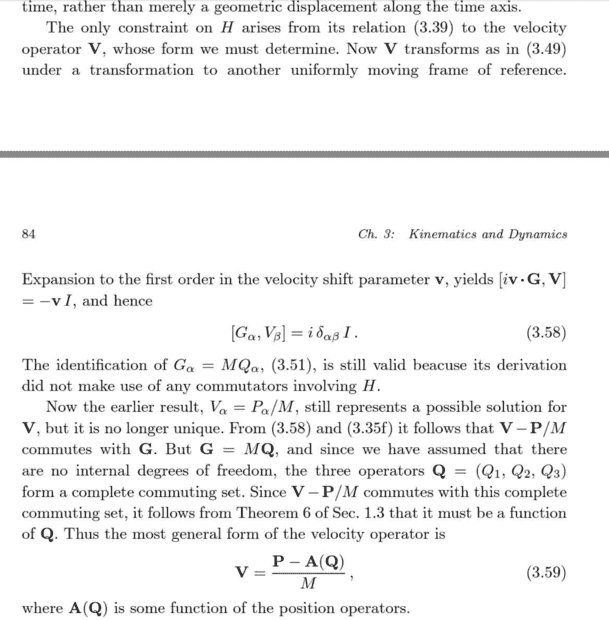
My confusion is as follows. In the two cases involving isolated particles before this step, Ballentine uses that an isolated particle is invariant under the full set of Galilean symmetries. This invariance under the full set of Galilean symmetries implied some specific commutators which were then used in the construction of the operators ($\textbf{J},\textbf{G}, \textbf{P}, H$) representing key observables. Ballentine then goes on to say that in the case of a particle interacting with external fields we can no longer keep the commutators involving $H$. My question has to do with this last statement:
1) Where does it sneak that the particle is isolated in developing the commutators between $\textbf{J},\textbf{G}, \textbf{P}, H$? It seems like these commutators simply emerge from observing that there is a correspondence (homomorphism) between Galilean transformations on spacetime and on $\mathcal{H}$ which we enforce and thus arrive at the commutators? Nowhere I don't think do we say that the physics is invariant under these transformations?

Well, we do say that the equations of physics are invariant under these transformations. This usually means we must represent physical quantities by mathematical entities that transform covariantly under those transformations.

As far as I can tell we only enforce that when we talk about how the position operator $\textbf{Q}$ transforms.

No, it sneaks in near the start of section 3.2 when Ballentine says: "The symmetries of space–time include rotations, displacements, and transformations between uniformly moving frames of reference." I've emboldened the word "uniformly" because it really implies transformations between inertial (unaccelerated) frames of reference.

In classical Hamiltonian mechanics, one works with a phase space, i.e., a position space, a momentum space, a time variable, and a Poisson bracket (type of commutator). One works with the Galilei transformations, as well as a Poisson bracket of the form $\{p_i\,,q_j\} = \delta_{ij}$. The latter becomes the usual canonical commutation relation when we construct a quantum version of a classical theory.

As to the "One may ask..." question: this is actually rather deep. Dirac wrote a seminal paper on this subject called "Forms of Dynamics", in which he explored the consequences arising from putting interaction terms together with the Hamiltonian (time translation), or with spatial translation, or another kind involving lightlike paths (IIRC - I skip over this here because it's relativistic stuff).

I'm not sure how to explain the choice to put interaction with the Hamiltonian any better than Ballentine already does. It's do with what we really mean by "physical action" and "dynamics as time-evolution". It boils down to Newton's 2nd law.

When we think of physical action and dynamics we're not talking exclusively about unaccelerated frames of reference any more. But the overall system must still obey Galilei transformations when viewed from the outside as a whole (at rest).

Hence the combination of entities inside a system and their interactions must somehow still result in the whole system obeying Galilei. Ballentine's treatment is one way of approaching that. A more general approach is to take the Action for the system and find its dynamical symmetry group (i.e., the group of transformations that map solutions of the equations of motion among themselves). If one cannot find, within the dynamical symmetry group, a subgroup with the same Lie algebra (and suitable interpretation) as Galilei-acting-on-spacetime, then something is probably wrong with the chosen Action.

HTH.

 

[EE18]

No, it sneaks in near the start of section 3.2 when Ballentine says: "The symmetries of space–time include rotations, displacements, and transformations between uniformly moving frames of reference." I've emboldened the word "uniformly" because it really implies transformations between inertial (unaccelerated) frames of reference.

Thank you for your very detailed response. I will only reply to the bit that I follow as the rest will require quite some thinking methinks.

In particular, you say what I've attached above. I agree that Ballentine says that, but where does he actually enforce it (mathematically)? In particular, it seems to me that (3.7), which is the only input to the governing equation (3.13), is just a statement about the representation of the Galilei group being a homomorphism? I don't see anywhere (until the dicussion about how $\textbf{Q}$ transforms) that the equations which actually enforce Galilean invariance (namely those equations at the top of page 67 or, equivalently, as introduced in (a) and (b) on page 63) are used to develop the commutators between the symmetry generators?

Also, would you be able to comment on (the second question in the OP) regarding why the system isn't Galilean invariant when there is an external field which is time-independent?

 

[strangerep]

In particular, you say what I've attached above. I agree that Ballentine says that, but where does he actually enforce it (mathematically)? In particular, it seems to me that (3.7), which is the only input to the governing equation (3.13), is just a statement about the representation of the Galilei group being a homomorphism?

(3.7) is statement that time translations for a 1-parameter (Lie) group, up to a phase (technically known as a centrally-extended group because of the extra generator $I$).

Similarly, the early discussion in sect 3.3 is just about how Lie groups can be built from generators via the exponential map. The discussion up to eq(3.12) is just a quick sketch of standard textbook stuff about how the generators of group necessarily satisfy a commutator algebra (the "Lie algebra").

So far, this is all standard Lie group theory, following from the assumption that the coordinate transformations of spacetime form a group -- the Galilei group in this nonrelativistic case. The only (tedious, tricky) bit in the quantum case is that the group multiplication law need only be satisfied up to a phase, which is the origin of the extra multiples of identity in the commutators. Ballentine shows which ones can be determined by consistency conditions, those which can removed without physical effect, and those which can't.

I don't see anywhere (until the dicussion about how $\textbf{Q}$ transforms) that the equations which actually enforce Galilean invariance (namely those equations at the top of page 67 or, equivalently, as introduced in (a) and (b) on page 63) are used to develop the commutators between the symmetry generators?

A little bit of this is near the bottom of p72. I suppose Ballentine is assuming the reader is already familiar with the classical Galilei group, so he doesn't give a full treatment beyond the leadup to (3.6) -- which is the multiplication law for arbitrary Galilei transformations in spacetime -- but moves quickly to the interesting quantum features. He deduces features of the commutation relation relations by examining individual rules for how parameters combine when transformations are composed -- e.g., pp70-71. I would have just derived the classical commutation relations from the coordinate formulas, and then noted that multiples of identity can be added to each commutator because of the phase freedom. But I daresay Prof Ballentine knows best.

Also, would you be able to comment on (the second question in the OP) regarding why the system isn't Galilean invariant when there is an external field which is time-independent?

It still IS Galilean invariant (meaning the Galilean Lie algebra is still satisfied) -- provided we use a different representation of the Galilei generators. That's what the "interacting form of dynamics" is all about. In this case, the "external field" induces this different representation -- the quantum particle no longer moves freely on a background of empty spacetime anymore, but rather with the external field as "background".

 

[EE18]

(3.7) is statement that time translations for a 1-parameter (Lie) group, up to a phase (technically known as a centrally-extended group because of the extra generator $I$).

Similarly, the early discussion in sect 3.3 is just about how Lie groups can be built from generators via the exponential map. The discussion up to eq(3.12) is just a quick sketch of standard textbook stuff about how the generators of group necessarily satisfy a commutator algebra (the "Lie algebra").

So far, this is all standard Lie group theory, following from the assumption that the coordinate transformations of spacetime form a group -- the Galilei group in this nonrelativistic case. The only (tedious, tricky) bit in the quantum case is that the group multiplication law need only be satisfied up to a phase, which is the origin of the extra multiples of identity in the commutators. Ballentine shows which ones can be determined by consistency conditions, those which can removed without physical effect, and those which can't.A little bit of this is near the bottom of p72. I suppose Ballentine is assuming the reader is already familiar with the classical Galilei group, so he doesn't give a full treatment beyond the leadup to (3.6) -- which is the multiplication law for arbitrary Galilei transformations in spacetime -- but moves quickly to the interesting quantum features. He deduces features of the commutation relation relations by examining individual rules for how parameters combine when transformations are composed -- e.g., pp70-71. I would have just derived the classical commutation relations from the coordinate formulas, and then noted that multiples of identity can be added to each commutator because of the phase freedom. But I daresay Prof Ballentine knows best. It still IS Galilean invariant (meaning the Galilean Lie algebra is still satisfied) -- provided we use a different representation of the Galilei generators. That's what the "interacting form of dynamics" is all about. In this case, the "external field" induces this different representation -- the quantum particle no longer moves freely on a background of empty spacetime anymore, but rather with the external field as "background".

I am unfortunately lost, I'm not sure I got any of that :( perhaps I need a bit more background in group theory for physics. I will keep reading Ballentine but maybe one day I'll return when I know as much as you and understand it!

 

[strangerep]

perhaps I need a bit more background in group theory for physics.

Maybe try "Quantum Mechanics -- Symmetries", by Muller & Greiner. That contains a more pedestrian introduction to the group theory applicable in QM.