Symmetry factor via Wick's theorem

[CAF123]

Consider the lagrangian of the real scalar field given by $$\mathcal L = \frac{1}{2} (\partial \phi)^2 - \frac{1}{2} m^2 \phi^2 - \frac{\lambda}{4!} \phi^4$$

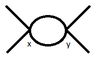
Disregarding snail contributions, the only diagram contributing to $\langle p_4 p_3 | T (\phi(y)^4 \phi(x)^4) | p_1 p_2 \rangle$ at one loop order is the so called dinosaur (see attached)

To argue the symmetry factor $S$ of this diagram, I say that there are 4 choice for a $\phi_y$ field to be contracted with one of the final states and then 3 choices for another $\phi_y$ field to be contracted with the remaining final state. Same arguments for the $\phi_x$ fields and their contractions with the initial states. This leaves 2! permutations of the propagators between x and y. Two vertices => have factor (1/4!)² and such a diagram would be generated at second order in the Dyson expansion => have factor 1/2. Putting this all together I get
$$S^{-1} = \frac{4 \cdot 3 \cdot 4 \cdot 3 \cdot 2!}{4! \cdot 4! \cdot 2} = \frac{1}{4}$$ I think the answer should be 1/2 so can someone help in seeing where I lost a factor of 2?

I could also evaluate $$\langle p_4 p_3 | T (\phi(y)^4 \phi(x)^4) | p_1 p_2 \rangle = \langle p_4 p_3 | : \phi(y)^4 \phi(x)^4 : | p_1 p_2 \rangle + \dots + (\text{contract}(\phi(x) \phi(y)))^2 \langle p_4 p_3 | : \phi(y)^2 \phi(x)^2 :| p_1 p_2 \rangle + \dots $$ where dots indicate diagrams generated via this correlator that do not contribute at one loop. (I don't know the latex for the Wick contraction symbol so I just write contract). Is there a way to find out the symmetry factor from computing the term $(\text{contract}(\phi(x) \phi(y)))^2 \langle p_4 p_3 | : \phi(y)^2 \phi(x)^2: | p_1 p_2 \rangle?$

Thanks!

 

[vanhees71]

You lost the factor of 2 in the very first step. Whether you connect a $\phi(y)$ with an initial or final state doesn't play any role and thus for the contraction of one of the $\phi(y)$ fields you have 8 (not only 4) possibilities. But now say, you've connected (counting the possibilities as 8 as discussed) this $\phi(y)$ with a final state, then you have only 3 possibilities left to connect the other final state, since due to the topology of the diagram now your final state is connected with the $\phi(y)$ and not the $\phi(x)$ fields. To connect one of the initial states you have 4 possibilities (either of the $\phi(x)$) and for the other initial state you have 3. To connect one of the remaining $\phi(x)$ with a $\phi(y)$ (dictated again by the topology of your diagram) you have 2 possibilities, and then there's only 1 possibility left to connect the other $\phi(x)$ with the one remaining $\phi(y)$. So you symmetry factor is all together
$8*4*3*4*3*2*1/((4!)^2*2!)=1/2$ as you expected.

 

[CAF123]

Thanks! It makes sense - is it possible to extract this symmetry factor using the Wick theorem as I have begun in the last part of my OP? --

I could also evaluate $$\langle p_4 p_3 | T (\phi(y)^4 \phi(x)^4) | p_1 p_2 \rangle = \langle p_4 p_3 | : \phi(y)^4 \phi(x)^4 : | p_1 p_2 \rangle + \dots + (\text{contract}(\phi(x) \phi(y)))^2 \langle p_4 p_3 | : \phi(y)^2 \phi(x)^2 :| p_1 p_2 \rangle + \dots $$ where dots indicate diagrams generated via this correlator that do not contribute at one loop. (I don't know the latex for the Wick contraction symbol so I just write contract). Is there a way to find out the symmetry factor from computing the term $(\text{contract}(\phi(x) \phi(y)))^2 \langle p_4 p_3 | : \phi(y)^2 \phi(x)^2: | p_1 p_2 \rangle?$

 

[nrqed]

Thanks! It makes sense - is it possible to extract this symmetry factor using the Wick theorem as I have begun in the last part of my OP? --

Yes, it goes through exactly as what you and Vanhees71 just figured out. What you must do is to contract four of the phi's (two of the $\phi(x)$ with the incoming states and two of the $\phi(y)$ with the outgoing states or vice versa, as you did in your counting) and then the two remaining $\phi(x)$ with the two remaining $\phi(y)$.

 

[CAF123]

Ok thanks - what I meant, however, was how to extract the correct coordinate space representation of the process using Wick's theorem with the result that the net numerical factor in front gives rise to the symmetry factor. I'll illustrate what I mean by the simplest example in $\phi^4$ theory, that of the local interaction vertex. This is produced (amongst other diagrams) in $\langle p_3 p_4 | T( \phi(x)^4) |p_1 p_2 \rangle$. The term of interest is the one with no contractions that is $\langle p_3 p_4 | : \phi(x)^4 :|p_1 p_2 \rangle$. Computing this should generate terms of the form $e^{-ix(p_1 + p_2 - p_3 - p_4)}$ so that it is equal to $e^{-ix(p_1 + p_2 - p_3 - p_4)} + \dots$. In the Dyson expansion I have therefore $$-\frac{i\lambda}{4!} \int \text{d}^4 x ( e^{-ix(p_1 + p_2 - p_3 - p_4)} + \dots) $$ Now, under the integration over $x$ the terms included in $\dots$ should give rise to terms such that the prefactor of 1/4! is cancelled. But what do these terms look like?

Thanks!

Edit: The result for $$p_3 p_4 | : \phi(x)^2 \phi(y)^2 : | p_1 p_2 \rangle = 4(e^{-i(p_1+p_2)y} e^{i(p_3+p_4)x} + e^{i(p_4-p_1)y} e^{i(p_3-p_2)x} + e^{i(p_4-p_2)y} e^{i(p_3-p_1)x} + (x \leftrightarrow y))$$ as in the OP. Specialising this to the case of $x=y$ as we have here this reduces to $4! \, e^{-ix(p_1 + p_2 - p_3 - p_4)}$ so that indeed the factor of 1/4! is cancelled. And the combinatoric argument without all this tedious calculation is that in the correlator we can contract four $\phi(x)$ fields with $p_1$, leaving three for $p_2$, two for $p_3$ and one for $p_4$. This gives 4! permutations of the prongs, again cancelling the 1/4!.

 

[nrqed]

Ok thanks - what I meant, however, was how to extract the correct coordinate space representation of the process using Wick's theorem with the result that the net numerical factor in front gives rise to the symmetry factor. I'll illustrate what I mean by the simplest example in $\phi^4$ theory, that of the local interaction vertex. This is produced (amongst other diagrams) in $\langle p_3 p_4 | T( \phi(x)^4) |p_1 p_2 \rangle$. The term of interest is the one with no contractions that is $\langle p_3 p_4 | : \phi(x)^4 :|p_1 p_2 \rangle$. Computing this should generate terms of the form $e^{-ix(p_1 + p_2 - p_3 - p_4)}$ so that it is equal to $e^{-ix(p_1 + p_2 - p_3 - p_4)} + \dots$. In the Dyson expansion I have therefore $$-\frac{i\lambda}{4!} \int \text{d}^4 x ( e^{-ix(p_1 + p_2 - p_3 - p_4)} + \dots) $$ Now, under the integration over $x$ the terms included in $\dots$ should give rise to terms such that the prefactor of 1/4! is cancelled. But what do these terms look like?
.

I am not sure what you mean by the dot dot dot. All the contributions are of the form of the term you wrote, there are simply 4! of them because of all the possible pairings.