- #1
Math Amateur
Gold Member
MHB
- 3,998
- 48
I am reading Bruce N. Coopersteins book: Advanced Linear Algebra (Second Edition) ... ...
I am focused on Section 10.1 Introduction to Tensor Products ... ...
I need help in order to get a basic understanding of Theorem 10.2 regarding the basis of a tensor product ... ...
My apologies if my previous questions on this matter are similar to earlier questions ... but I have been puzzling over this theorem for some time ... and still do not have a full understanding of it ...Theorem 10.2 reads as follows:

My questions are as follows:
1. What is the nature/form of the elements of ##X'## and ##X## and how are they related to each other ...
2. What are the nature/form of the elements of ##Z## and ##Z'## and how are they related to each other ... and further, what is the form of the non-basis elements of ##Z## and ##Z'## ... ...
Apologies ... ... I know I have not formulated the question very precisely ... ...
 ... ... but nonetheless I hope someone is able to help ...
... ... but nonetheless I hope someone is able to help ...
Peter============================================================
*** EDIT ***
I have been reflecting on my own questions above ... here are my thoughts ...
Elements of ##X'## would be of the form
##x = (v_1, v_2, \ ... \ ... \ , v_m)## with ##v_i \in \mathcal{B}_i##Elements of ##X = V_1 \times V_2 \times \ ... \ ... \ \times V_m## would be of the form
##x = (v_1, v_2, \ ... \ ... \ , v_m)## with ##v_i \in V_i##and ... ...
... since I imagine ##\mathcal{B}_i \subseteq V_i ## ... then we have ##X' \subseteq X## ... ... (Now ... can we say any more about the form of the elements of X' and X?
Is the above all we can say? )
Now, before outlining the form of the elements of ##Z'## ... we just note that we are asked to identify each element ##x = (v_1, v_2, \ ... \ ... \ , v_m) \in X' ## with ##\chi_x \in Z'## ... ...Now, ##Z'## is a vector space over the field ##\mathbb{F}##, so there will be an operation of addition of elements of ##Z'## and a scalar multiplication ... ...
So ... if ##x_1 = (v_{11}, v_{21}, \ ... \ ... \ , v_{m1}) \in X'## and if ##c_1 \in \mathbb{F}## ... ...
... then ##c_1 \chi_{x_1} \in Z'##Similarly ##c_2 \chi_{x_2} \in Z'## and so on ...
So, by operations of addition we can form elements of the form
##c_1 \chi_{x_1} + c_2 \chi_{x_2} + \ ... \ ... \ + c_n \chi_{x_n}## ... ... ... ... ... (1)
... and (1) above is the general form of elements in ##Z'## ... ...If we then identify ##c_i \chi_{x_i}## with ##x_i## we can view the elements of ##Z'## as ##c_1 (v_{11}, v_{21}, \ ... \ ... \ , v_{m1}) + c_2 (v_{12}, v_{22}, \ ... \ ... \ , v_{m2}) + \ ... \ ... \
+ c_n (v_{1n}, v_{2n}, \ ... \ ... \ , v_{mn})##
BUT ... THEN ... what form do the elements of ##Z## have ... especially those that are in ##Z##] but not in ##Z'## ... ... ?Can someone please critique my analysis ... and comment on the elements of ##Z## ... especially those not in ##Z'##... ...
========================================================
========================================================NOTE:
The early pages of Cooperstein's Section 10.1 give the notation and approach to understanding tensor products and hence to understanding the notation and concepts used in Theorem 10.2 ... ... hence I am providing the first few pages of Section 10.1 as follows:
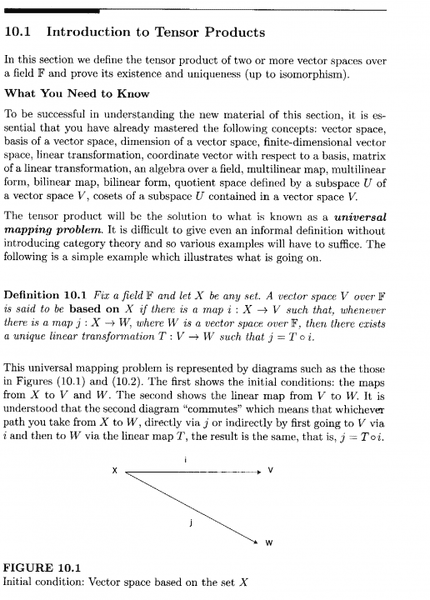



I am focused on Section 10.1 Introduction to Tensor Products ... ...
I need help in order to get a basic understanding of Theorem 10.2 regarding the basis of a tensor product ... ...
My apologies if my previous questions on this matter are similar to earlier questions ... but I have been puzzling over this theorem for some time ... and still do not have a full understanding of it ...Theorem 10.2 reads as follows:
My questions are as follows:
1. What is the nature/form of the elements of ##X'## and ##X## and how are they related to each other ...
2. What are the nature/form of the elements of ##Z## and ##Z'## and how are they related to each other ... and further, what is the form of the non-basis elements of ##Z## and ##Z'## ... ...
Apologies ... ... I know I have not formulated the question very precisely ... ...
Peter============================================================
*** EDIT ***
I have been reflecting on my own questions above ... here are my thoughts ...
Elements of ##X'## would be of the form
##x = (v_1, v_2, \ ... \ ... \ , v_m)## with ##v_i \in \mathcal{B}_i##Elements of ##X = V_1 \times V_2 \times \ ... \ ... \ \times V_m## would be of the form
##x = (v_1, v_2, \ ... \ ... \ , v_m)## with ##v_i \in V_i##and ... ...
... since I imagine ##\mathcal{B}_i \subseteq V_i ## ... then we have ##X' \subseteq X## ... ... (Now ... can we say any more about the form of the elements of X' and X?
Is the above all we can say? )
Now, before outlining the form of the elements of ##Z'## ... we just note that we are asked to identify each element ##x = (v_1, v_2, \ ... \ ... \ , v_m) \in X' ## with ##\chi_x \in Z'## ... ...Now, ##Z'## is a vector space over the field ##\mathbb{F}##, so there will be an operation of addition of elements of ##Z'## and a scalar multiplication ... ...
So ... if ##x_1 = (v_{11}, v_{21}, \ ... \ ... \ , v_{m1}) \in X'## and if ##c_1 \in \mathbb{F}## ... ...
... then ##c_1 \chi_{x_1} \in Z'##Similarly ##c_2 \chi_{x_2} \in Z'## and so on ...
So, by operations of addition we can form elements of the form
##c_1 \chi_{x_1} + c_2 \chi_{x_2} + \ ... \ ... \ + c_n \chi_{x_n}## ... ... ... ... ... (1)
... and (1) above is the general form of elements in ##Z'## ... ...If we then identify ##c_i \chi_{x_i}## with ##x_i## we can view the elements of ##Z'## as ##c_1 (v_{11}, v_{21}, \ ... \ ... \ , v_{m1}) + c_2 (v_{12}, v_{22}, \ ... \ ... \ , v_{m2}) + \ ... \ ... \
+ c_n (v_{1n}, v_{2n}, \ ... \ ... \ , v_{mn})##
BUT ... THEN ... what form do the elements of ##Z## have ... especially those that are in ##Z##] but not in ##Z'## ... ... ?Can someone please critique my analysis ... and comment on the elements of ##Z## ... especially those not in ##Z'##... ...
========================================================
========================================================NOTE:
The early pages of Cooperstein's Section 10.1 give the notation and approach to understanding tensor products and hence to understanding the notation and concepts used in Theorem 10.2 ... ... hence I am providing the first few pages of Section 10.1 as follows:
Attachments
-
 Cooperstein - 3 - Theorem 10.2 .... ....png57.5 KB · Views: 696
Cooperstein - 3 - Theorem 10.2 .... ....png57.5 KB · Views: 696 -
 Cooperstein - 1 - Section 10.1 - PART 1 ....png70.8 KB · Views: 609
Cooperstein - 1 - Section 10.1 - PART 1 ....png70.8 KB · Views: 609 -
 Cooperstein - 2 - Section 10.1 - PART 2 ....png38.4 KB · Views: 593
Cooperstein - 2 - Section 10.1 - PART 2 ....png38.4 KB · Views: 593 -
 Cooperstein - 3 - Section 10.1 - PART 3 ....png35.3 KB · Views: 596
Cooperstein - 3 - Section 10.1 - PART 3 ....png35.3 KB · Views: 596 -
 Cooperstein - 4 - Section 10.1 - PART 4 ....png33.1 KB · Views: 627
Cooperstein - 4 - Section 10.1 - PART 4 ....png33.1 KB · Views: 627