Test whether the diets are different from one another at ##α=5\%##

[chwala]

Homework Statement

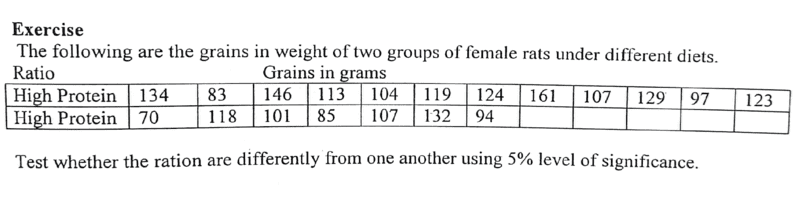
See attached

Relevant Equations

stats

Looking at stats today,

In my working i have;

Let

$H_0 = μ_1=μ_2$

v/s

$H_1 = μ_1-μ_2≠ 0$

then,

$\bar x = \dfrac{134+83+...+123}{12}=120$

$\bar y = \dfrac{70+118...+94}{7}=101$

$t=\dfrac{\bar x- \bar y}{S_p ⋅\sqrt {\dfrac{1}{n_1}+\dfrac{1}{n_2}}}$

$t=\dfrac{120-101}{21.21 \sqrt {\dfrac{1}{12}+\dfrac{1}{7}}}$

$t=1.89$

and,

$t_{[17, 0.05]} =2.11$

since $t_{calculated} < t_{[17, 0.05]}$ that is $1.89 < 2.11$ we accept the null hypothesis that the ration are not different from one another and reject the alternative hypothesis that the ration are different from one another.

your insight is welcome...cheers.

 

[Mark44]

$t_{[17, 0.05]} =2.11$

Did you use a two-tailed test or a one-tailed test?

As a side note, whatever statistics textbook this is, the publisher didn't spend much money on copy edits.
"The following are the grains in weight..." -- gains in weight?
"Test whether the ration are differently ..."
The table headings are confusing, with both rows headed by "High Protein." The table rows could be more easily understood if the rows showed the two groups of mice.

 

[chwala]

Did you use a two-tailed test or a one-tailed test?

As a side note, whatever statistics textbook this is, the publisher didn't spend much money on copy edits.
"The following are the grains in weight..." -- gains in weight?
"Test whether the ration are differently ..."
The table headings are confusing, with both rows headed by "High Protein." The table rows could be more easily understood if the rows showed the two groups of mice.

Two tailed test.

 

[Mark44]

The formulas you show seem to be the right ones, but to verify your result I would need to do a fair amount of calculations that you don't show, such as the two sample means, the two sample variations (and standard deviations), and would need to check that you came up with the right value that corresponds to the t value you found.

 

[chwala]

The formulas you show seem to be the right ones, but to verify your result I would need to do a fair amount of calculations that you don't show, such as the two sample means, the two sample variations (and standard deviations), and would need to check that you came up with the right value that corresponds to the t value you found.

That's the whole essence of insight, you're a mathematician...the sample mean is shown ...I know that you can calculate the pooled variance too... Verification is an exercise on your part...

Your part (insight ); would be to tell whether my substitutions are correctly done...and whether there are better alternatives...

...I am pretty sure you that you can check from the t distribution table... degree of freedom vs the given alpha level where I got the $2.11$ value. Cheers mate.

 

[Mark44]

That's the whole essence of insight, you're a mathematician...the sample mean is shown

I know how to do this, and have done it many times in the past. I just don't feel like going through the motions to do so. What you could do is to double-check your calculations.

I know that you can calculate the pooled variance too... Verification is an exercise on your part...

My idea about the insights is to confirm that you have used the right formulas, but not re-do all your calculations.

Your part probably would be to tell whether my substitutions are correct.

...I am pretty sure you also can check from the t distribution table... degree of freedom vs the given alpha level where I got the 2.11#. cheers mate.

Same as above.