The angle at which a skier will leave the sphere

[simphys]

Homework Statement

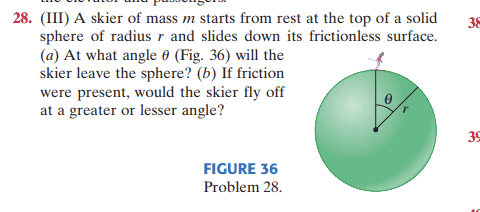
III) A skier of mass m starts from rest at the top of a solid
sphere of radius $r$ and slides down its frictionless surface.
(a) At what angle $/theta$ (Fig. 36) will the
skier leave the sphere? (b) If friction
were present, would the skier fly off
at a greater or lesser angle?

Relevant Equations

conserfation of mechanical energy concept(only translational KE considered)

Back here again.. but I am sorry guys, only the parameters are on my paper so wouldn't be of much help to show. I basically have no clue on how to start solving this problem at all.
I stopped yesterday and let it sit overnight but still have no clue on how to approach the problem basically. Because what in the world would indicate and thus tell me when it will leave that spehere.
It's a 3* problem as well.

So what I am asking is.. Could I get some tips on how to start this problems please?
Thanks in advance.

What do I know?
So grav force is the only one that's doing work aka energy stored. Normal force does no work for that's about it (a).
for (b) friction(NC force) is involved as well.

problem in picture format:

 

[simphys]

does it have to do something with where from $a_n = v^2/r$ $r$ becomes bigger or something and perhaps relate that to conservation of energy?

 

[simphys]

ONE SECOND I MIGHT BE ONTO SOMETHING... $a_n$ ACTUALLY BECOMES ZERO AT THE END ZO VELOCITY AT THE POINT WHERE THE SKIER WILL LEAVE THE SPHERE IS ZERO.

 

[Orodruin]

ONE SECOND I MIGHT BE ONTO SOMETHING... $a_n$ ACTUALLY BECOMES ZERO AT THE END ZO VELOCITY AT THE POINT WHERE THE SKIER WILL LEAVE THE SPHERE IS ZERO.

No. What you call $a_n$ is the centripetal acceleration required to keep the skier on the circular path. You need to think about what provides this centripetal acceleration and whether that is possible or not.

 

[simphys]

No. What you call $a_n$ is the centripetal acceleration required to keep the skier on the circular path. You need to think about what provides this centripetal acceleration and whether that is possible or not.

No. What you call $a_n$ is the centripetal acceleration required to keep the skier on the circular path. You need to think about what provides this centripetal acceleration and whether that is possible or not.

yep i came at pi/2 but now that I read it again it doesn't make sense that V is zero lol...
i will use NT's law to equate the force of gravity providing the centripetal acceleration to $v^2/r$ then?

Damn I will real close... pff

 

[Orodruin]

By the way. The (b) part is one of those fundamentally misguided problem statements where the problem stater has not really thought things through.

 

[simphys]

By the way. The (b) part is one of those fundamentally misguided problem statements where the problem stater has not really thought things through.

I will skip that part then if that's okay.
one question though I am in a dilemma here...
for (a) is there a normal force or not? because if we assume no friction N = 0 so to say? or I can't assume that equation at all? edit: $N = \mu_0*F_{fr}$

Because otherwise I get $mg*cos(\theta) - N = m*a_n$ where I have no way to remove N

 

[Orodruin]

I will skip that part then if that's okay.
one question though I am in a dilemma here...
for (a) is there a normal force or not? because if we assume no friction N = 0 so to say? or I can't assume that equation at all? edit: $N = \mu_0*F_{fr}$

Because otherwise I get $mg*cos(\theta) - N = m*a_n$ where I have no way to remove N

The normal force will be whatever it needs to be to keep the skier from falling into the sphere. What values can $N$ take? What is the limit when the skier is about to fall off?

 

[simphys]

The normal force will be whatever it needs to be to keep the skier from falling into the sphere. What values can $N$ take? What is the limit when the skier is about to fall off?

right! it'll be zero because it's just about to lift off. Same as weightnessless where it's normal force becomes zero.

 

[simphys]

it's basically what I did here but taking centripetal acceleration into account. as the force that causes it.

 

[Orodruin]

sorry this was a wrong

No, it wasn’t.

it's basically what I did here but taking centripetal acceleration into account. as the force that causes it.
View attachment 304157

It is essentially impossible to tell what you have been trying to do here. Please type out your arguments and your math and don’t simply post pictures of scribbles assuming that we will understand what you mean.

 

[simphys]

No, it wasn’t.

It is essentially impossible to tell what you have been trying to do here. Please type out your arguments and your math and don’t simply post pictures of scribbles assuming that we will understand what you mean.

yep I am doing that right now indeed

 

[simphys]

Need to find the velocity at which it leaves, what do I use?
Nt's law for centripetal acceleration:
$mgcos(\theta) = ma_n --> g = v_2^2/r$
it becomes: $v_2 = sqrt{gr}$

Then we know that conservation of ME applies:
$mgy_1 + 1/2*mv_1^2 = 1/2*mv_2^2 + mgy_2$
y_2 is derived from the figure as $y_2 = rcos(\theta)$
This givese a relation for finding $\theta$ so, we get:
$mgr + 0 = 1/2*m*(sqrt{gr})^2 + mgrcos\theta$
removing m and g and we get:
$1 = 1/2 + cos\theta$
$cos\theta = 1/2$
$\theta = 60 degrees$

however it's $48$ degrees as answer

 

[Orodruin]

Nt's law for centripetal acceleration:
$mg = ma_n --> g = v_2^2/r$
it becomes: $v_2 = sqrt(gr)$

Not quite. In which direction does the gravitational force act? How much of the gravitational force can provide centripetal acceleration?

 

[simphys]

Not quite. In which direction does the gravitational force act? How much of the gravitational force can provide centripetal acceleration?

oh my god... I've written the angle in my fbd but forgot to include it

This changes the game completely ofcourse...

 

[simphys]

Not quite. In which direction does the gravitational force act? How much of the gravitational force can provide centripetal acceleration?

got it, thanks a lot @Orodruin ! as you indicated, I won't bother with (b) tough.

This one was a little tougher for the beginning but I guess solving problems like these just takes practice to recognize what concepts are to be used.

 

[Orodruin]

as you indicated, I won't bother with (b) tough.

I did not say don’t think about it. Rather, think about why it is badly posed.

 

[simphys]

tbh.. at 1st I wanted to just skip the exercise but now that I am thinking about it.. from now on I think I am never going to skip the 3*(/more difficult) problems because that's where you learn the most even if it takes way longer..

the 1*-2* ones is mostly just applying the learned concept and not too tough.

 

[simphys]

I did not say don’t think about it. Rather, think about why it is badly posed.

because of the normal force from which we can't derive a numerical solution?
never mind.. because friction is tangential

 

[simphys]

is it because then the more general law of conservation of energy needs to be applied?
the terms to be added $W_{f_n} = \mu_k*N*d$
Where $d$ is not findable it's $d = r*s$, $s$ being the arclength

 

[Delta2]

is it because then the more general law of conservation of energy needs to be applied?
the terms to be added $W_{f_n} = \mu_k*N*d$
Where $d$ is not findable it's $d = r*s$, $s$ being the arclength

In that formula for the work of friction the problem is that the normal force $N$ varies along the trajectory...So you have to make it an integral instead of a simple multiplication. And this complicates things. The problem would be modeled by an integral equation for $\theta(t)$ which probably can't be solved by analytical means...

 

[simphys]

In that formula for the work of friction the problem is that the normal force $N$ varies along the trajectory...So you have to make it an integral instead of a simple multiplication. And this complicates things. The problem would be modeled by an integral equation for $\theta(t)$ which probably can't be solved by analytical means...

damn it right of course... thank you.
yeah that's basically integration that to get the work done by friction after knowing what you just described it becomes simple indeed.

 

[kuruman]

In that formula for the work of friction the problem is that the normal force $N$ varies along the trajectory...So you have to make it an integral instead of a simple multiplication. And this complicates things. The problem would be modeled by an integral equation for $\theta(t)$ which probably can't be solved by analytical means...

I agree with all that. However, I disagree that part (b) is mathematically complicated or badly stated. In part (b) the problem is not asking for the angle at which the skier is losing contact when friction is present. It is asking "If friction were present, would the skier fly off at a greater of lesser angle?"

The question can be answered without having to calculate the actual angle. With friction present, just consider that, at the critical angle where the skier flies off in part (a), the speed of the skier will be less. The condition for losing contact is obtained from the FBD at the critical angle and is the same with or without friction: $$g\cos\!\theta_c=\frac{v^2}{R}.$$ What happens to $\theta_c$ when $v$ decreases?

 

[Lnewqban]

$mgr + 0 = 1/2*m*(sqrt{gr})^2 + mgrcos\theta$
removing m and g and we get:
$1 = 1/2 + cos\theta$
$cos\theta = 1/2$
$\theta = 60 degrees$

however it's $48$ degrees as answer

The distribution of the different forces acting on the skier will change in your FBD as the angle increases.

With a lot of friction present, the final tangential velocity could not be too high; therefore, the take-off angle will get closer to 90 degrees (where N and any horizontal forces become very small).

On the other hand, with plenty of initial kinetic energy (the skier being pushed forward) and no friction, the take-off angle would get closer to zero, as the tangential velocity would be greater.

 

[SammyS]

I have observed that when @Orodruin makes a point it's prudent to give thoughtful consideration to what he says.

I don't pretend to know precisely what he has in mind here:

By the way. The (b) part is one of those fundamentally misguided problem statements where the problem stater has not really thought things through.

I note the following: At the starting position, the skier is at a point of equilibrium - unstable equilibrium.

The problem states that the skier starts from rest. Well, we know that actually the skier must be given a very small nudge or must start just slightly displaced from the very top of the sphere - in either case just a small enough amount so as not to affect the result in a significant way for part (a) of the problem.

For part (b), with friction, a small displacement from the top will result in no motion. a small enough nudge and the skier quickly stops.

I don't know if this is what @Orodruin had in mind, but that's what came to mind for me.

 

[Orodruin]

For part (b), with friction, a small displacement from the top will result in no motion. a small enough nudge and the skier quickly stops.

I don't know if this is what @Orodruin had in mind, but that's what came to mind for me.

Yes, this was indeed my objection. The skier will never start moving with just a small nudge if there is friction. You will need a nudge that is at least large enough to get to where the tangential component of the weight overcomes friction in order not to stop.

The problem stater likely had in mind something like subtracting work done by friction when computing the speed, but since the required starting velocity cannot be arbitrarily close to zero, it requires different initial conditions.

 

[Orodruin]

I agree with all that. However, I disagree that part (b) is mathematically complicated or badly stated. In part (b) the problem is not asking for the angle at which the skier is losing contact when friction is present. It is asking "If friction were present, would the skier fly off at a greater of lesser angle?"

I disagree. See above.

With the stated initial conditions, the skier simply won’t move.

 

[malawi_glenn]

@Orodruin will the skier move when placed on top of the sphere even with friction absent? Consider point-like skier. Some intial velocity is needed also in that case.

Sure one can argue that such configuration is not stable and any small movement of the skiers CM (if not point like skier) will cause the skier to move down along the sphere.

 

[Orodruin]

@Orodruin will the skier move when placed on top of the sphere even with friction absent? Consider point-like skier.

No, but the equilibrium is unstable as was mentioned in #25. This means that even the tiniest of deviations from the equilibrium (either spatial or in velocity) will make the skier go down the slope. This is typically what is intended by this type of problem and is more or less ok since you can consider the ”start on top” as a limiting case that also is a good approximation to ”starts just next to the top”. This is no longer the case when you introduce friction. There will then be a finite angle that the skier must be displaced to to have the same sort of situation.

 

[hmmm27]

There will then be a finite angle that the skier must be displaced to to have the same sort of situation.

Or, "some friction" can be interpreted as "non-uniform" (and also "not enough at any given point to stop the skier").

 

[Orodruin]

Or, "some friction" can be interpreted as "non-uniform" (and also "not enough at any given point to stop the skier").

You can, but that still requires making additional constraining assumptions on the problem that are not part of the problem statement.

 

[kuruman]

You can, but that still requires making additional constraining assumptions on the problem that are not part of the problem statement.

It does indeed. There are additional unmentioned assumptions, such as "Assume that the skis and the skier is a point mass; however, although a point mass has no air resistance, assume that the force it is distributed over a finite area so that the skier does not sink in $~dots~$" and the list goes on. I am not being facetious. I am asking where, in an introductory physics problem statement, one draws the inclusion line of assumptions that are absolutely to necessary to answer the question. Too many listed assumptions detract, especially beginners who are not experienced enough to decide for themselves what is important. Students are unlikely to become engaged with introductory physics problems that read like legal documents

I draw a line at the point where the statement of the problem is not sufficient to result in an answer unless physically unreasonable assumptions are made. However, what's physically unreasonable, in my opinion, needs to be tempered by the pedagogical utility of the question. This particular problem requires the combination of mechanical energy conservation with dynamics and circular motion. Its utility is that it shows to students that the concepts they have seen in separate textbook chapters are integral parts of the same underlying reality. That, in my mind, is sufficient reason to overlook the fuzziness of the assumptions and the lack of specific initial conditions. I am OK with the idea of a point skier merrily sliding on a frictionless sphere on point skis having started from rest by being a bit to the side of the top or by being gently pushed. That takes care of part (a). In part (b) I am still OK with the idea that this skier started a bit off to the side at an angle that was already larger than the angle of repose.

I don't see any reason for drawing a line between parts (a) and (b). I think that the utility of part (b) is to reinforce the connection between energy conservation and dynamics made in part (a). Others may disagree with me and draw the line elsewhere. I am hoping that we can agree to disagree.

 

[Orodruin]

I don't see any reason for drawing a line between parts (a) and (b). I think that the utility of part (b) is to reinforce the connection between energy conservation and dynamics made in part (a). Others may disagree with me and draw the line elsewhere. I am hoping that we can agree to disagree.

We can of course agree to disagree. To me, the introduction of friction destroys the entire (idealised) model and the argument behind the solution.