The Colors of The Moon (Doppler Effect)

[dom_quixote]

Hey guys!

I will pass an illustrated problem, below.

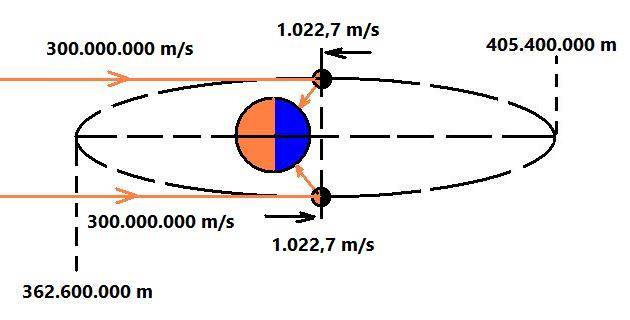
- We know that the solar rays that reach the Moon and Earth are practically parallel;

- We know that the lunar orbit with respect to Earth lasts 27.322 days or 2,360,621 seconds;

- We know that the lunar orbit with respect to the Earth is elliptical;

- We know that 27.322 days the distance between the Moon and Earth varies between 362,600 km and 405,400 km.

In order to simplify our calculations, let us consider an average circular orbit:

[405,400,000 m + 362,600,000 m] / 2 = 384,000,000 m

The perimeter of the average lunar orbit is equal to:

2 * pi * 384,000,000 m = 2,412,743,158 m

The speed of the Moon relative to its average orbit is:

2,412,743,158 m / 2,360,621 s = 1,022.7 m/s

We ask:

Do we have enough technology to detect the Doppler effect of sunlight reflected by the Moon from the Earth's surface?

 

[anuttarasammyak]

From your numbers I calculate v/c = 3.4E-6. Balmer spectre of n=3 has wave length of 656 nm would undertake the wave length difference of 2.2 pico meter. I am not good at relevant technologies at all but suppose the effect is too tiny to detect.

 

[Keith_McClary]

There are some narrow lines:

NIST

 

[dom_quixote]

Thank you, anuttarasammyak!
Thanks, Keith_McClary!

I consulted about the Doopler effect on low-orbiting satellites.
The frequency deviation of the electromagnetic communication signals seems to me very considerable.

Graphic
https://en.wikipedia.org/wiki/Doppler_effect#/media/File:SatDoppler.png

Source
https://en.wikipedia.org/wiki/Doppler_effect