The Definition of Torque - a proof

[Shreya]

Homework Statement

Please refer the image below.

Relevant Equations

Newton's Laws

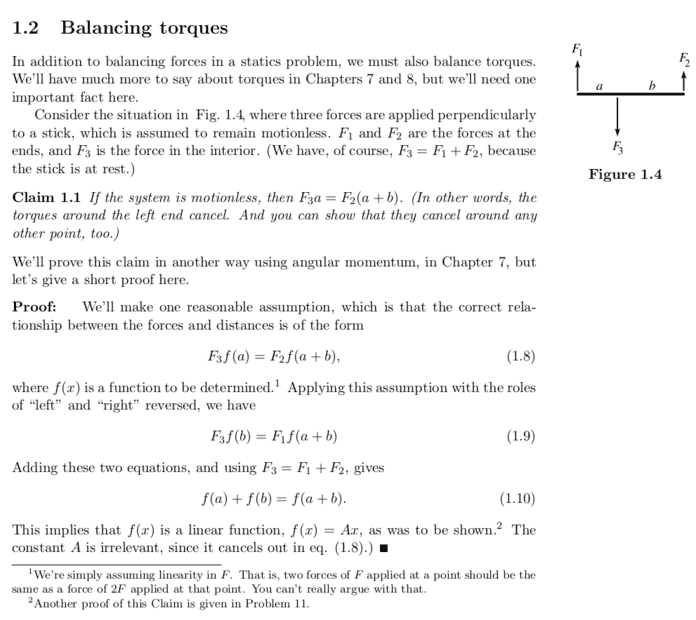
I have been trying to understand this proof from the book 'Introduction to classical mechanics' by David Morin. This proof comes up in the first chapter of statics and is a proof for the definition of torque.
I don't understand why the assumption taken in the beginning of the proof is reasonable. A note given at the end tries to give some clarification, but I can't relate these 2 points.
Please be kind to help. :)

 

[haruspex]

How about this:
The "effectivity" of a force of magnitude F applied in a specified direction at x from the axis is $g(F,x)$. Applying some multiple of F, $\lambda F$, in the same direction and at the same point should have effectivity $\lambda g(F,x)$, i.e. $\lambda g(F,x)=g(\lambda F,x)$. Hence $\frac{\partial g(F,x)}{\partial F}=\frac{\partial g(\lambda F, x)}{\partial F}$. Since every force in the specified direction can be represented by a suitable choice of $\lambda$, this implies $\frac{\partial g}{\partial F}$ is a function of x only. Integrating, $g=F f(x)+c$ for some function $f$.
Adding that a zero force should have zero effect leads to the result.

 

[Shreya]

Sorry, but I don't quite understand the partial differential equation, @haruspex . Should'nt there be a $\lambda$ on the left.

 

[haruspex]

Sorry, but I don't quite understand the partial differential equation, @haruspex . Should'nt there be a $\lambda$ on the left.

Sorry, I didn't write the algebra correctly.
$\lambda g(F,x)=g(\lambda F,x)$. Hence $\lambda\frac{\partial g}{\partial F}\vert_{F,x}=\lambda\frac{\partial g}{\partial F}\vert_{\lambda F, x}$.
Cancelling,
$\frac{\partial g}{\partial F}\vert_{F,x}=\frac{\partial g}{\partial F}\vert_{\lambda F, x}$.
Since every force in the specified direction can be represented by a suitable choice of $\lambda$, this implies $\frac{\partial g}{\partial F}$ is a function of x only. Integrating, $g=F f(x)+c$ for some function $f$.

 

[Shreya]

Yes sir, I understood it now. Just to check if I got it properly, I'll try to summarise here. The 'effectiveness of rotation' of a force, taken as $g(F,x)$ is a function of the force F and the distance from the origin x. If the force be scaled by $\lambda$, the effectiveness $g(F,x)$ must also be scaled by that factor. This implies that the change in effectiveness per change in F just depends on x (and not on the particular value of F). Therefore, effectiveness itself is a linearly dependent on f and some function of x. I might have lost some rigorousness here due to departure from mathematical notation, but I hope I have understood the idea properly.

I also wanted to correlate the assumption with the note 1 given at the end. From your explanation, $\lambda g (F,x) = g (\lambda F, x)$ means the same as the note, right?

 

[haruspex]

I hope I have understood the idea properly.

Yes, you get the idea.

From your explanation, $\lambda g (F,x) = g (\lambda F, x)$ means the same as the note, right?

My explanation is an attempt to use the hint to arrive at the answer. Whether that's what the author had in mind I cannot be sure.

 

[Shreya]

Thank you so much @haruspex for helping me out again. I had tried many resources to understand this question and all that had failed. You are doing a great help to all students in the world