The radius of convergence of a series

[Amaelle]

Homework Statement

look at the image

Relevant Equations

r=an+1/an

Greetings!
I have a problem with the solution of that exercice

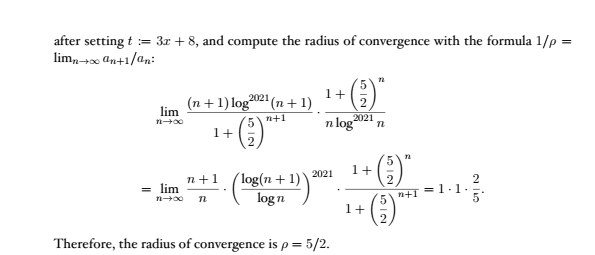
I don´t agree with it because if i choose to factorise with 6^n instead of 2^n will get 5/6 instead thank you!

 

[BvU]

if i choose to factorise with 6^n instead of 2^n will get 5/6

Can you show us how you do that ?

$\$

 

[Amaelle]

thank you
here it is

 

[BvU]

So your $t = x+{16\over 6}$. What is your $a_n$ ? It looks as if the $2^n$ in the denominator has disappeared ...

$\$

 

[Amaelle]

So your $t = x+{16\over 6}$. What is your $a_n$ ? It looks as if the $2^n$ in the denominator has disappeared ...

$\$

of course according to the asymptotic aprroximation 5^n >2^n so I got rid of it

 

[BvU]

So if you factorize with, say, 24, you get $\displaystyle{5\over 24}$ ?

$\$

 

[Amaelle]

thank you!
First could you confirm that my assymptotic approximation is correct?
second my original approch was to put y=6x+16 which gives me a raduis of 5/6 I just don´t understand this book approach

 

[Mark44]

$2^n(3x + 8)^n = 6^n(x + \frac 8 3)^n$. Of course $x + 8/3$ is the same as $x + 16/6$, but why leave it in this unsimplified form?

In your work using the Ratio Test, you have omitted the $6^n(x + \frac 8 3)^n$ part, which is a mistake.

 

[Amaelle]

thank you but
the ration test is an+1/an and (x+8/3)^n is not part of it's part of t^n

 

[Mark44]

the ration test is an+1/an

The Ratio Test for a power series definitely includes the part with the x + 8/3 factor. It's how you determine the radius of convergence.
For example, for the power series $\displaystyle \sum_{i = 0}^\infty a_n(x - c)^n$, the Ratio Test would be to evaluate this limit:
$\displaystyle \lim_{n \to \infty} \frac {a_{n+1}(x - c)^{n+1}}{a_n(x - c)^n}$.

BTW, when you write "an+1/an" this would be interpreted by most as $a_n + \frac 1 {a_n}$. If you don't use LaTeX, write this expression as (an + 1)/(an).

 

[BvU]

thank you but
the ration test is an+1/an and (x+8/3)^n is not part of it's part of t^n

That is why I asked you to post your $a_n$. What I see in #3 is wrong.

$\$

 

[Amaelle]

what´s wrong with it? I posted all the elements I have and done, I would be grateful if you could point out the problem clearely.

 

[BvU]

what´s wrong with it? I posted all the elements I have and done, I would be grateful if you could point out the problem clearely.

Excuse me ? Your first $=$ sign is wrong, the second $=$ sign is wrong. And you can not just 'get rid of' the 2^n.

What is your $a_n$ ?

$\$

 

[Amaelle]

And you can not just 'get rid of' the 2^n.
$\$

is not 5^n+2^n ~ 5^n assymptotically?

 

[Mark44]

what´s wrong with it? I posted all the elements I have and done, I would be grateful if you could point out the problem clearely.

The general term of you series is this:
$$\frac{n \log^{2021}(n)}{2^n + 5 ^n}6^n(x + 16/6)^n$$
In the second line of your work in post #3 you have this, I think -- your work is hard to read in places:
$$\frac{ (n + 1) \log^{2022}(n) 6^{n+1} 5^n} {5^{n + 1} n \log^{2021}(n) 6^n } $$
This is wrong on several counts.
1. The log function doesn't get bumped up another power. It should be $\log^{2021}(n + 1)$.
2. As BvU said, you can't just throw away the $2^n$ term. Instead, factor it out to get $2^n(1 + (5/2)^n)$.
3. You should also not throw away the x + 8/3 factor.

And you can not just 'get rid of' the 2^n.

Yes

 

[BvU]

is not 5^n+2^n ~ 5^n assymptotically?

No. The difference gets bigger (by a factor of 2) with every step

$\$

 

[BvU]

3. You should also not throw away the x + 8/3 factor.

We look at the series $\sum a_n \, t^n$. Apparently there is a theorem that the radius of convergence is equal to $1/\lim {a_{n+1}/a_n}$.

$\$

 

[Amaelle]

We look at the series $\sum a_n \, t^n$. Apparently there is a theorem that the radius of convergence is equal to $1/\lim {a_{n+1}/a_n}$.

$\$

yes

 

[vela]

We look at the series $\sum a_n \, t^n$. Apparently there is a theorem that the radius of convergence is equal to $1/\lim {a_{n+1}/a_n}$.

For convergence, the ratio test requires
$$\lim_{n \to \infty} \left\lvert \frac{a_{n+1}t^{n+1}}{a_n t^n} \right\rvert = \lvert t \rvert \lim_{n \to \infty} \left\lvert \frac{a_{n+1}}{a_n} \right\rvert < 1,$$ so we have
$$\lvert t \rvert < \lim_{n \to \infty} \left\lvert \frac{a_{n}}{a_{n+1}} \right\rvert.$$

In the solution, the value of the limit is claimed to be the radius of convergence, but presumably, when we talk about the radius of convergence, we're talking about the size of the interval in terms of $x$, not $t$. It seems to me you still need to rescale the value of the limit depending on how $t$ is defined in terms of $x$.

 

[Amaelle]

For convergence, the ratio test requires
$$\lim_{n \to \infty} \left\lvert \frac{a_{n+1}t^{n+1}}{a_n t^n} \right\rvert = \lvert t \rvert \lim_{n \to \infty} \left\lvert \frac{a_{n+1}}{a_n} \right\rvert < 1,$$ so we have
$$\lvert t \rvert < \lim_{n \to \infty} \left\lvert \frac{a_{n}}{a_{n+1}} \right\rvert.$$

In the solution, the value of the limit is claimed to be the radius of convergence, but presumably, when we talk about the radius of convergence, we're talking about the size of the interval in terms of $x$, not $t$. It seems to me you still need to rescale the value of the limit depending on how $t$ is defined in terms of $x$.

Thanks a million vela,
that was my question and my concern!
indeed after rescaling it we find 5/6.

 

[Amaelle]

Thanks @BvU @Mark44 for taking time to answer me !

 

[BvU]

Thanks a million vela,
that was my question and my concern!
indeed after rescaling it we find 5/6.

Same exercise with $\displaystyle 1\over 4^n + 5^n$ instead of $\displaystyle 1\over 2^n + 5^n\$: do you get the same answer or a different one ?

@vela: yes, the book answer leaves unmentioned that the $\rho$ found applies to $3x+8$.

$\$

 

[Mark44]

book answer leaves unmentioned that the ρ found applies to 3x+8.

Which is why I kept saying that we need the 3x + 8 term (or x + 8/3) to find the interval of convergence.

 

[Amaelle]

Same exercise with $\displaystyle 1\over 4^n + 5^n$ instead of $\displaystyle 1\over 2^n + 5^n\$: do you get the same answer or a different one ?

@vela: yes, the book answer leaves unmentioned that the $\rho$ found applies to $3x+8$.

$\$

Yes I got the same result (without doing the problematic assymptotic approximation you pointed out)