The Set of Borel Sets .... Axler Pages 28-29 .... ....

[Math Amateur]

I am reading Sheldon Axler's book: Measure, Integration & Real Analysis ... and I am focused on Chapter 2: Measures ...

I need help in order to fully understand the set of Borel sets ... ...

The relevant text reads as follows:

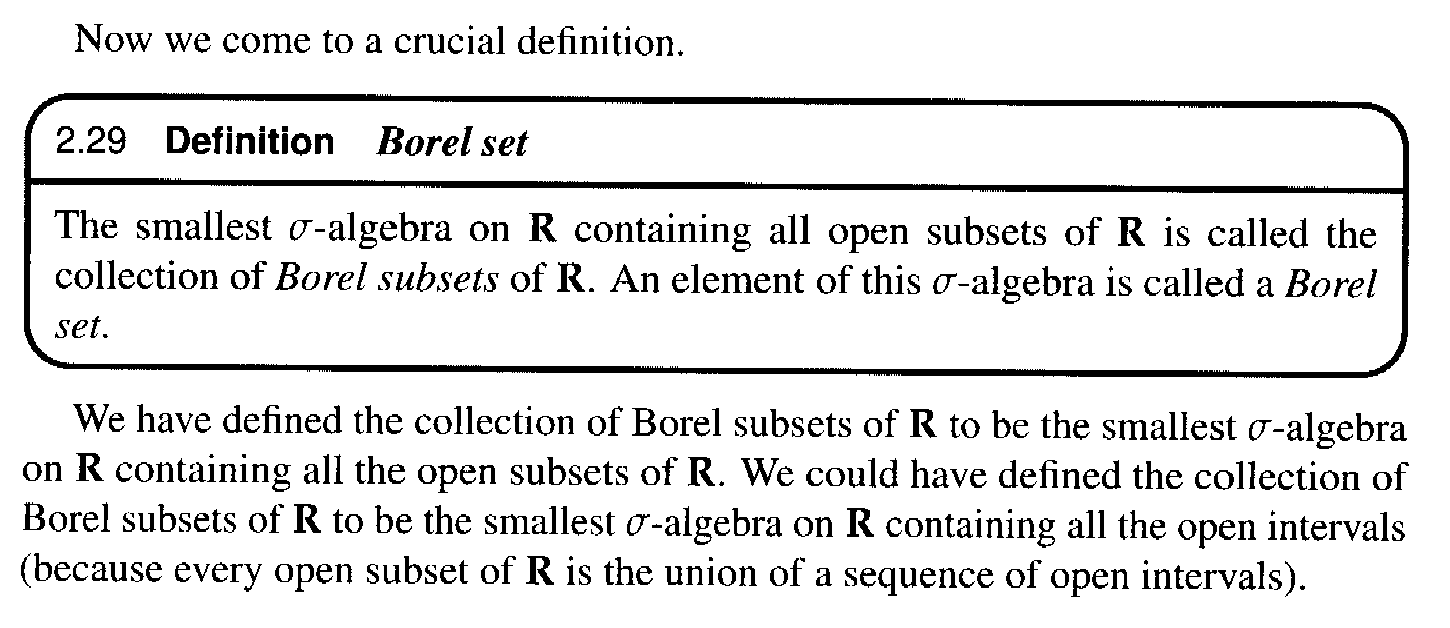
My questions related to the above text are as follows:QUESTION 1

In the above text by Axler we read the following:

" ... ... However, the set of all such intersections is not the set of Borel sets (because it is not closed under countable unions). ... ..."Can someone please explain why exactly that the set of all such intersections is not the set of Borel sets ... ? Why exactly is such a set not closed under countable unions and why is this relevant?
QUESTION 2

In the above text by Axler we read the following:

" ... ... The set of all countable unions of countable intersections of open subsets of $\mathbb{R}$ is also not the set of Borel sets (because it is not closed under countable intersections). ... ... "Can someone please explain why exactly that the set of all countable unions of countable intersections of open subsets of $\mathbb{R}$ is not the set of Borel sets ... ? Why exactly is such a set not closed under countable intersections and why is this relevant?
Help with the above two questions will be much appreciated ...

Peter

 

[Opalg]

WARNING! This question leads into difficult territory.

The Borel sets form a $\sigma$-algebra. So every countable union, and every countable intersection, of Borel sets is a Borel set. You might hope that, starting with the open sets, then adding all countable intersections of open sets, then all countable unions of those sets, then all countable intersections ... , and so on, you might be able to generate all Borel sets. But that is not so. It turns out the there is an infinite hierarchy of larger and larger classes of sets formed in that way.

A countable intersection of open sets is known as a $G_\delta$ set. For example, a set consisting of a single point is a $G_\delta$ set. Since the set of rational numbers $\Bbb{Q}$ is countable, $\Bbb{Q}$ is a countable union of Borel sets and is therefore a Borel set. But $\Bbb{Q}$ is not a $G_\delta$ set (as shown in that Wikipedia link). After that, the examples get rapidly more complicated. The set of all $x$ for which $$\lim_{n\to\infty}\sin(n!\pi x) = 0$$ is a countable union of $G_\delta$ sets but is not itself a $G_\delta$ set (see here). That answers your Question 1.

I do not think that Sheldon Axler is expecting you to construct such examples yourself.

 

[Math Amateur]

WARNING! This question leads into difficult territory.

The Borel sets form a $\sigma$-algebra. So every countable union, and every countable intersection, of Borel sets is a Borel set. You might hope that, starting with the open sets, then adding all countable intersections of open sets, then all countable unions of those sets, then all countable intersections ... , and so on, you might be able to generate all Borel sets. But that is not so. It turns out the there is an infinite hierarchy of larger and larger classes of sets formed in that way.

A countable intersection of open sets is known as a $G_\delta$ set. For example, a set consisting of a single point is a $G_\delta$ set. Since the set of rational numbers $\Bbb{Q}$ is countable, $\Bbb{Q}$ is a countable union of Borel sets and is therefore a Borel set. But $\Bbb{Q}$ is not a $G_\delta$ set (as shown in that Wikipedia link). After that, the examples get rapidly more complicated. The set of all $x$ for which $$\lim_{n\to\infty}\sin(n!\pi x) = 0$$ is a countable union of $G_\delta$ sets but is not itself a $G_\delta$ set (see here). That answers your Question 1.

I do not think that Sheldon Axler is expecting you to construct such examples yourself.

Thanks Opalg ...

Still reflecting on what you have written ...

But ... could you help a bit further ...

You write:

" ... ... A countable intersection of open sets is known as a $G_\delta$ set. For example, a set consisting of a single point is a $G_\delta$ set. ... "

Could you please demonstrate how/why a set consisting of a single point is a $G_\delta$ set. ...EDIT ...

It's OK ... we have

$\{x\}= \bigcap_{n=1}^\infty(x-1/n, x+1/n)$ ...

So $\{x\}$ is a $G_\delta$ set ...

Peter