Theoretical maximum efficiency of a heat engine without Carnot

[KedarMhaswade]

TL;DR Summary

It seems possible to find out the theoretical maximum efficiency of a closed-circuit heat engine without invoking the Carnot cycle and its ideal adiabatic processes. Is it?

Through an intriguing fictitious dialog between Sadi Carnot and Robert Sterling, Prof. Israel Urieli of the Ohio University shows that it is not required to invoke entropy, the second law of thermodynamics, and the Carnot cycle with the [ideal] adiabatic processes in order to find out the maximum theoretical efficiency of a heat engine: https://www.ohio.edu/mechanical/thermo/Intro/Chapt.1_6/Carnot_Stirling/index.html

For the sake of completeness, I reproduce it here:

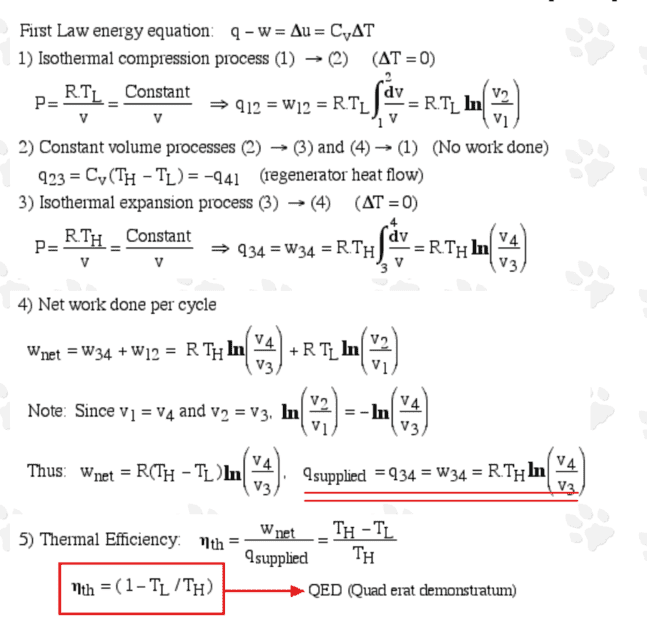
I think the analysis is plausible; the interesting thing is about the heat exchanger (regenerator) which, under ideal conditions, provides a perfect heat transfer between the gas and the regenerator equipment (including the coolant). The only slight confusion is whether $q_{supplied} = q_{34}$ is the only energy transferred into the engine by heat during the isothermal expansion?

Does anyone disagree with this analysis? Please elucidate either way. Note that apparently the professor won a prize for his http://www.centrostirling.com/isec2014/index-isec2014.html.

 

[nbryan5]

Sadi Carnot: Robert, I believe that the maximum efficiency of a heat engine is determined by the temperature difference between its hot and cold reservoirs.Robert Sterling: But Sadi, how do we determine the maximum efficiency without invoking entropy and the second law of thermodynamics?Sadi Carnot: We don't need to invoke entropy or the second law of thermodynamics to find out the maximum theoretical efficiency of a heat engine. We can use an ideal adiabatic process and simply calculate the energy balance between the energy supplied and the energy extracted from the heat engine. The energy supplied is the energy transferred into the engine by heat during the isothermal expansion. The energy extracted is the energy transferred out of the engine by work during the isothermal compression. The maximum efficiency is then the ratio of the energy extracted to the energy supplied.