Thermodynamics problem relating to Chemical Potentials

[JohnJ]

Homework Statement

A 1.05 L bottle of a carbonated soft drink contains 1.0 L of drink as is pressurized at 5 atm with
CO2 gas. At this pressure, the CO2 concentration in the drink is given by Henry’s law,
C = 0.031 P_g , where C is the concentration in moles/L and P_g is the partial pressure of the gas
(5 atm).
(a) What is the chemical potential of the CO2 in this system relative to the gas at atmospheric
pressure?
(b) The cap is briefly loosened, so that the gas comes fully into equilibrium with the atmosphere,
but the dissolved CO2 remains in solution. The bottle is then sealed.
What are the chemical potentials of the CO2 in the drink and the gas above?
(c) Finally, the bottle is shaken, such that the contents come into equilibrium. Describe what
happens, and calculate what is the pressure inside the bottle now?
Approximately how many times can this process be repeated before the drink goes flat?

Relevant Equations

Raoult's Law, Dalton's Law, Equations relating to chemical potentials

For part (a), I used this formula

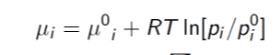
where where the i's represent the substance being used and mu_i^0 represents some reference potential. However, to my knowledge this simply calculates the change in chemical potential from one state to another which is not of much help in finding the relative chemical potential of the gas and the liquid. So I think my answer to (a) is wrong.
For (b) I'm completely lost. I'm not sure if now we take it that the gas is in equilibrium with the atmosphere but is now not in equilibrium with the drink. So then the liquid in the drink and gas in the drink are different temperatures. I'm not sure what new information I can extract from the fact that the gas has come into thermal equilibrium with the atmosphere.
Thanks,

 

[mjc123]

So I think my answer to (a) is wrong.

As you haven't told us your answer to (a), how can we tell?

However, to my knowledge this simply calculates the change in chemical potential from one state to another which is not of much help in finding the relative chemical potential of the gas and the liquid.

If the gas and the liquid are in equilibrium , what does that tell you about the chemical potentials in the gas and the liquid?

In part (b), you can certainly take it that the gas is no longer in equilibrium with the liquid, and a new equilibrium has to be established. What I'm not sure of is whether it means simply that the gas pressure is reduced to 1 atm, or whether it implies full mixing with the atmosphere, so that the partial pressure of CO₂ is the same as that in the atmosphere (which you are not told, but would need to answer part (b)). The latter is how I would understand "fully in equilibrium", but seems inconsistent with a brief loosening of the cap and the liquid not re-equilibrating. It would take time.

 

[JohnJ]

As you haven't told us your answer to (a), how can we tell?

If the gas and the liquid are in equilibrium , what does that tell you about the chemical potentials in the gas and the liquid?

In part (b), you can certainly take it that the gas is no longer in equilibrium with the liquid, and a new equilibrium has to be established. What I'm not sure of is whether it means simply that the gas pressure is reduced to 1 atm, or whether it implies full mixing with the atmosphere, so that the partial pressure of CO₂ is the same as that in the atmosphere (which you are not told, but would need to answer part (b)). The latter is how I would understand "fully in equilibrium", but seems inconsistent with a brief loosening of the cap and the liquid not re-equilibrating. It would take time.

Thanks for your reply.
For part (a) are you suggesting that the answer is then zero, as the chemical potentials are the same due to equilibrium? I can't say that this is incorrect but it's not something that I've heard before.
For (b) I still don't know how I would handle given the information. If we say that the partial pressure of the CO2 is now 1 atm what formula would then be useable to calculate the chemical potential?

 

[mjc123]

(a) Yes. At equilibrium the chemical potentials of CO₂ in the gas and solution are the same. Now you can answer the question. (You had the right equation, just do the calculation.)
(b) The same formula. (I don't think you can, or are expected to, calculate absolute chemical potentials - calculate them, as in (a), relative to CO₂(g) at 1 atm.)