- #1
Tomas Vencl
- 66
- 13
- TL;DR Summary
- Time dilation and length contraction graphically
The thread "twin paradox without math" inspired me try to find fully graphical solution of length contraction.
Here is the result:
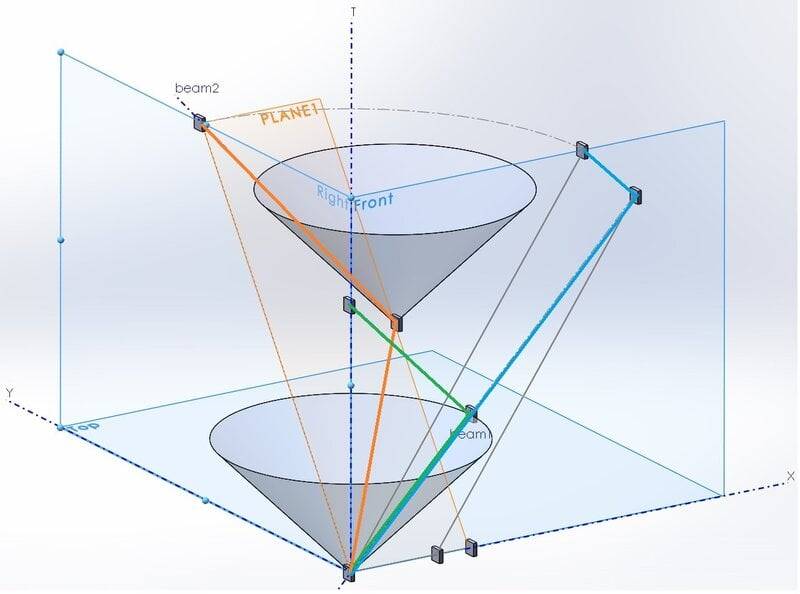
Graph is 3D as I think that is ilustrative to make all in one graph, but I am sure that it is possible to do it in 2 or 3 2D slices.
X and Y are spatial axes, T is time (static observer), light cone is 45 degree, so this is Minkowski spacetime.
There are 3 sets of light clocks at the picture.
The green one is standard static (in front plane) light clock (one tick) and defines scales of the axes (for example, if mirors are 1light second distant, the tick last 2 seconds).
The orange light beam is 2 second tick of moving (along Y axe, in plane 1) clock. The orange beam is intersection of plane 1 and lightcone surface.
The blue light beam is 2 second tick of clock moving along X axe. Because all clocks shoud show the same time independently on their orientation (at given speed), the 2 second tick of blue clocks should have the same duration as orange clocks. From this condition you can derive length contaction. Simply draw 45 degree blue lines from origin and final position of first mirror of blue clocks and their intersection defines necessary event of reflecting beam at second mirror of blue light clocks. This also define length contaction of blue light clocks.
Here is the result:
Graph is 3D as I think that is ilustrative to make all in one graph, but I am sure that it is possible to do it in 2 or 3 2D slices.
X and Y are spatial axes, T is time (static observer), light cone is 45 degree, so this is Minkowski spacetime.
There are 3 sets of light clocks at the picture.
The green one is standard static (in front plane) light clock (one tick) and defines scales of the axes (for example, if mirors are 1light second distant, the tick last 2 seconds).
The orange light beam is 2 second tick of moving (along Y axe, in plane 1) clock. The orange beam is intersection of plane 1 and lightcone surface.
The blue light beam is 2 second tick of clock moving along X axe. Because all clocks shoud show the same time independently on their orientation (at given speed), the 2 second tick of blue clocks should have the same duration as orange clocks. From this condition you can derive length contaction. Simply draw 45 degree blue lines from origin and final position of first mirror of blue clocks and their intersection defines necessary event of reflecting beam at second mirror of blue light clocks. This also define length contaction of blue light clocks.