Time dilation for a simple problem — Light propagating inside a moving bus

[name]

Homework Statement

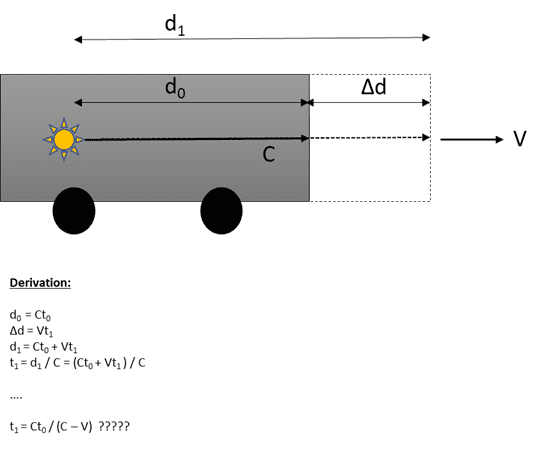
Calculate the time it takes for the light to travel a straight line distance d1 in a bus moving at a constant velocity V as observed by an external observer.

Relevant Equations

N/A derived below.

So I drew the problem and tried to derive t1 for an external observer by making the following assumptions.

1. Inside observer sees light travel a distance of d0 meters in t0 seconds at a speed of c m/s.
2. Bus moved Δd meters in t1 seconds at V m/s.
3. Outside observer sees light travel a distance of d1 meters in t1 seconds at a speed of c m/s.

I know this is incorrect but I don't see where the error is?

Thank You

 

[TSny]

The distance $d_0$ is the distance between the light source and the right end of the bus according to the observer inside the bus. What is this distance according to the external observer?

 

[Chestermiller]

A simple way of solving a problem like this is by direct application of the Lorentz Transformation.

Event 1: t' = t = x' =x =0

Event 2: $x'=d_1$, $t'=\frac{d_1}{c}$, $\ x = ?$, $t = ?$

 

[name]

The distance $d_0$ is the distance between the light source and the right end of the bus according to the observer inside the bus. What is this distance according to the external observer?

I have it as $d_1$ as per my assumptions which is the sum of $d_0$ and $Δd$. Is that incorrect?

A simple way of solving a problem like this is by direct application of the Lorentz Transformation.

Event 1: t' = t = x' =x =0

Event 2: $x'=d_1$, $t'=\frac{d_1}{c}$, $\ x = ?$, $t = ?$

If $x$ the distance according to the external observer, then that would be $d_1 = ct_0 + Vt_1$, no?

 

[Chestermiller]

What does the Lorentz transformation give for this?

 

[TSny]

I have it as $d_1$ as per my assumptions which is the sum of $d_0$ and $Δd$. Is that incorrect?

This is not quite correct. You defined $d_0$ as the distance between the light source and the front of the bus as measured by someone inside the bus. However, $d_1$ is a distance measured by the external observer. At any instant of time according to the external observer, the distance between the light source and the front of the bus is not $d_0$.

Imagine that there is a stick inside of the bus that moves with the bus. The stick extends between the light source and the front of the bus. For observers inside the bus, the stick is at rest and is measured to have a length $d_0$. For the external observer, the stick is in motion. What is the length of the stick according to the external observer?

 

[Chestermiller]

$$t=\gamma(t'+V\frac{x'}{c^2})$$
$$x=\gamma(x'+Vt')$$

 

[Chestermiller]

Imagine that there is a stick inside of the bus that moves with the bus. The stick extends between the light source and the front of the bus. For observers inside the bus, the stick is at rest and is measured to have a length $d_0$. For the external observer, the stick is in motion. What is the length of the stick according to the external observer?

I don't think that this "stick" approach is going to work, because one event occurs at x' = 0 at t' = 0, and the second event occurs at x' = $d_1$ at time t' = $d_1/c$.

 

[TSny]

I don't think that this "stick" approach is going to work, because one event occurs at x' = 0 at t' = 0, and the second event occurs at x' = $d_1$ at time t' = $d_1/c$.

I find that both approaches yield the same answer. Using the Lorentz transformation equations makes it easy. I wanted to show the OP where they made their mistake.

 

[Chestermiller]

I find that both approaches yield the same answer. Using the Lorentz transformation equations makes it easy. I wanted to show the OP where they made their mistake.

I'd be interested in seeing your answer. Private communication?

 

[name]

Thank you guys, I really appreciate the time both of you have taken to respond.

Just a bit of background on this question and why I was confused. I started by trying to derive time dilation equation by drawing the typical example of a bus moving at a constant speed V with the light source starting from the bottom and propagating vertically instead of horizontally as my initial example in this thread.

Using simple trig, I was able to derive the time an external observer would observe (see attached)
$$t = \gamma * t_0$$.

While I was aware of the Lorenz Transformation, no where in my derivation did I really have to "think" about it.

I thought I could do the same with the light source propagating in the x direction this time. It's obviously trickier than I thought as it seems like I was also neglecting length contraction, so my assumption of total distance being equal to d0 + Δd was completely wrong.

 

[PeroK]

Here's a question. If there is length contraction in the direction of motion, how can you rule out length contraction in a direction at right-angles to the motion?

 

[TSny]

I thought I could do the same with the light source propagating in the x direction this time. It's obviously trickier than I thought as it seems like I was also neglecting length contraction, so my assumption of total distance being equal to d0 + Δd was completely wrong.

The only mistake you made when you set up $d_1 = d_0 + \Delta d$ was that you didn't take into account that the distance $d_0$ is Lorentz contracted to $d_0/\gamma$ in the ground frame. So, you should of written $$d_1 = \frac{d_0}{\gamma} + \Delta d$$ Then make your substitutions $d_1 = ct_1$, $d_0 = ct_0$, and $\Delta d = vt_1$. What do you get when you solve for $t_1$ in terms of $t_0$?