- #1
Barnak
- 63
- 0
I'm having some interpretation problems with a rotating dipolar magnetic field, so I need your opinion (I apologise for my bad English).
Let there be a magnetic moment "mu" tilted relative to the rotation axis (the "z" axis), with an inclination angle "alpha". The magnetic moment is steadily rotating with the angular velocity "w". It then generates a time dependant magnetic field with radiation (see Jackson, section 9.3). I already have all the magnetic field components and was able to draw the field lines, at t = 0 (see the picture below, for the case alpha = 90°). The yellow curves are the "last closed" magnetic field lines, inside the "light cylinder" defined by R_L = c/w. The blue curves are some radiation lines. All the curves shown represents the total magnetic field (including near, intermediate and far fields). The red circle is just a reference equator. The total magnetic field has the following mathematical form (the vectorial notation is implied) :
B(t, x, alpha) = F(x) sin(alpha) cos(wt) + G(x) sin(alpha) sin(wt) + H(x) cos(alpha),
with F, G and H three complicated vectors dependant of the space location only (and "w/c", of course). Apparently, the field is simply LOCALLY rotating, but it isn't clear that this should also imply that the field lines are ALL GLOBALLY rotating without any deformation. The numerical evaluation, using Mathematica, seems to indicate that they do rotate like a rigid body, despite the presence of EM radiation flowing out ! This is very surprising to me, and I was expecting some complicated time evolution with lines deformations, a bit like a fluid or an organic shape. So is it possible that, in the special case of a rotating magnetic dipole, the total magnetic field lines are simply rotating like a rigid body, even with the presence of EM waves flowing out ?
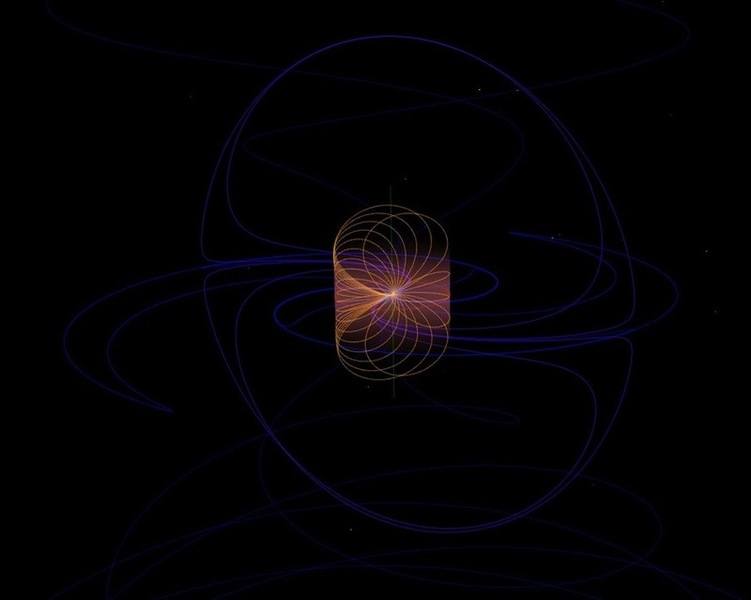
Let there be a magnetic moment "mu" tilted relative to the rotation axis (the "z" axis), with an inclination angle "alpha". The magnetic moment is steadily rotating with the angular velocity "w". It then generates a time dependant magnetic field with radiation (see Jackson, section 9.3). I already have all the magnetic field components and was able to draw the field lines, at t = 0 (see the picture below, for the case alpha = 90°). The yellow curves are the "last closed" magnetic field lines, inside the "light cylinder" defined by R_L = c/w. The blue curves are some radiation lines. All the curves shown represents the total magnetic field (including near, intermediate and far fields). The red circle is just a reference equator. The total magnetic field has the following mathematical form (the vectorial notation is implied) :
B(t, x, alpha) = F(x) sin(alpha) cos(wt) + G(x) sin(alpha) sin(wt) + H(x) cos(alpha),
with F, G and H three complicated vectors dependant of the space location only (and "w/c", of course). Apparently, the field is simply LOCALLY rotating, but it isn't clear that this should also imply that the field lines are ALL GLOBALLY rotating without any deformation. The numerical evaluation, using Mathematica, seems to indicate that they do rotate like a rigid body, despite the presence of EM radiation flowing out ! This is very surprising to me, and I was expecting some complicated time evolution with lines deformations, a bit like a fluid or an organic shape. So is it possible that, in the special case of a rotating magnetic dipole, the total magnetic field lines are simply rotating like a rigid body, even with the presence of EM waves flowing out ?
Last edited: