- #1
brojas7
- 20
- 0
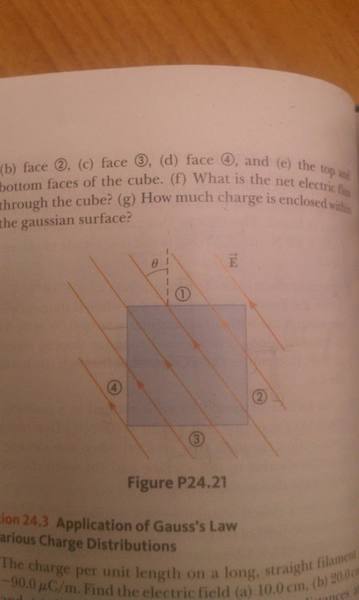
The field makes an angle θ with side 1 and the area of each face is A. In symbolic form, find the electric flux through (a) face 1, (b) face 2, (c) face 3, (d) face 4 and (e) top and bottom.
My professor got:
a=EAcosθ
b=-EAsinθ
c=-EAcosθ
d=EAsinθ
e= 0
I understant why e=0 but for the other sides, for some reason I am not understanding why she got for example a=EAcosθ and not sinθ. Which part of the triangle am I trying to find out? Would it be the adjacent for each or the opposite side for each?
I hope my question makes sense.
My professor got:
a=EAcosθ
b=-EAsinθ
c=-EAcosθ
d=EAsinθ
e= 0
I understant why e=0 but for the other sides, for some reason I am not understanding why she got for example a=EAcosθ and not sinθ. Which part of the triangle am I trying to find out? Would it be the adjacent for each or the opposite side for each?
I hope my question makes sense.