Torque calculation based on distributive load

[arihantsinghi]

Homework Statement

Calculate the minimum torque needed to stop the rod from moving, if the entire BC portion of the rod touches the ground. static coefficient of friction is (mu).

Relevant Equations

T = F*l and trigonometric equations

The following images contain the question as well as my solution. I am not sure whether my solution is correct or not. Please help.

 

[haruspex]

Your image is much too blurry. I cannot even figure out the diagram.
Please add a verbal description of the set up, provide a clearer diagram and type in your working, preferably in LaTeX, per forum rules.

 

[arihantsinghi]

Sorry for the blurry image. I have attached the scanned Pdf. I hope this is clearer. If you still need a Latex file please inform me. Thanks

 

[jbriggs444]

Sorry for the blurry image. I have attached the scanned Pdf. I hope this is clearer. If you still need a Latex file please inform me. Thanks

Please put the words into your message, not scribbled within your scanned image.

The problem had been posted in another sub-forum here previously. So some inside information is available.

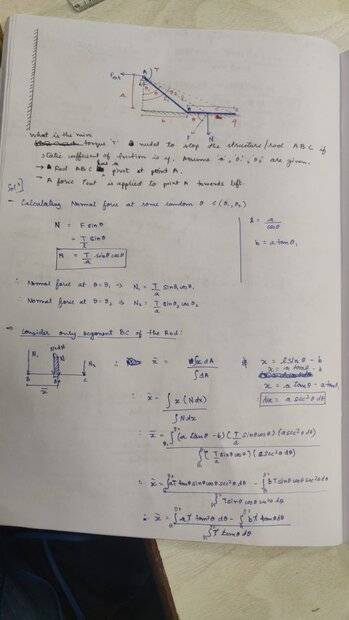
The situation as I understand starts with an elevated hinge. The anchored side of the hinge can be thought of as attached to a cart that is free to slide horizontally and frictionlessly to the right and left. The cart is anchored vertically and rotationally. A leftward external force $F_\text{ext}$ is applied to the cart.

There is mechanism within the hinge that exerts a torque $T$ on the free side of the hinge. The hinge is otherwise frictionless.

Attached to the free side of the hinge is a rigid and massless rod that extends to the right and down. There is an upward bend somewhere in the middle of the rod. The rod continues with a straight section extending further to the right.

The bend is at exactly the right angle so that the straight section of the rod sits level and flat on the ground.

The question is about the lowest value of $T$ that can produce enough static friction to prevent the rod+hinge+cart assembly from being pulled to the left by the external force $F_\text{ext}$ on the cart.

I would suggest that the system is statically indeterminate. The answer hinges on determining a center of pressure of ground on rod. But if all components are assumed to be perfectly rigid then the position of that center cannot be determined from the given information.

Still, an answer can be obtained by treating the center of pressure as falling within a permissible range of positions and looking for an extreme case.

It is also possible that this is a question about real world materials, stresses and resulting deflections. In that case, more information is required before an answer can be obtained. We would need to know about the stiffness of the various materials. How do they deform under load?

 

[Lnewqban]

@arihantsinghi ,
Consider that friction force does not depend on area of contact between the dragging portion of the bar (BC) and the ground.
Only the normal force and the coeficient of static friction are important for what it is a braking mechanism.

Since your torque will not induce a constant normal force along BC, only the greatest value of a punctual normal force should be interesting to us, rather than an average value of that distributed force.

In order to make that BC bar an effective “parking brake” surface, it should have a central pivot that allows natural accomodation to changes of level and angle of the road respect to that horizontally sliding top pivot.

 

[haruspex]

In order to make that BC bar an effective “parking brake” surface, it should have a central pivot that allows natural accomodation to changes of level and angle of the road respect to that horizontally sliding top pivot.

Not sure what you are saying there. As @jbriggs showed, you would minimise the required torque by dispensing with BC altogether.