Baluncore said:
The code box for general code was broken, so I used a .pdf file.
I think you should focus on page 3 of Con_Rod_2.pdf
Are you happy with vectors as complex numbers ?
How are you getting on with understanding the modeling of the linkage ?
Thanks for coming back to me
@Baluncore, I appreciate it. I have to admit, I can't follow that code, unfortunately. The focus for me is at the friction and the other suggestions for the mechanical design. It is getting a bit confused in my head. I think we are almost there, but still missing something.
Mech_LS24 said:
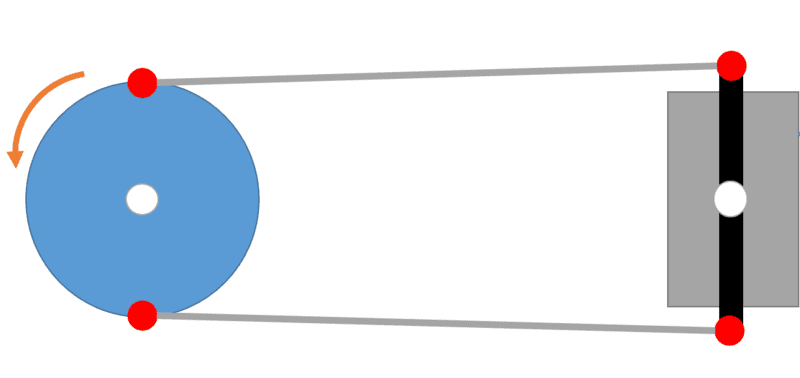
I doesn't need two solid rods, but I though this will help decreasing the amount of force on the rods (as shown below). What are piano wire links/strings? Google searches brings me to real piano's

I can't find the piano wire links meaning, as mentioned in post#29, do you have documentation or so about these?
Mech_LS24 said:
Thanks for the explanation @Baluncore. Unfortunately it is difficult for me to get a feeling for friction. If I am working on concepts, I try to argument what consideration should be taken into account. With a concept like this, I could say as a disadvantage the amount of friction in the rods. Therefore maybe a system with belt/pulley would be the better choice. But always the question remains, what is that amount of friction? A general, simple calculation would help here. But as I understand, friction is a very difficult subject?
Last, I am wondering how that friction should be calculated. I think we should first focus on a mechanism with the servo, disk and
one rod. That should mean the system has two rod ends and the one 7200 bearing. The amount of weight for the mechanism is been determined in post#15. With my current knowledge, this should be the 'input' for calculating the amount of friction right? When the friction is known, I can add the torque for rotating the disk and decide what amount of torque that servo needs to supply.
Mech_LS24 said:
Thus, I can take the 81.4 N as force and the radius to the path of the ball centre as arm? So:
M = F*arm length
M = 81.4(N)*10(mm) = 814 Nmm = 0.81 Nm
Now I can filter motor with an amount of torque of at least 0.81(Nm) + 1.13 (Nm) = 1.95 Nm. If I add a safety factor of 1.5 makes it, 2.92 Nm.
Correct?
This is the calculation I did in post#19, it is supported with a sketch as well in that post. Could you please verify it? The outcome of those numbers feels 'good' to me, but I doesn't get the feeling of friction in my fingers yet, haha..
Hopefully, a 'push' in the right direction would bring me to solve this :)