Total angular momentum of a translating and rotating pancake

[Rikudo]

I have read Classical Mechanics book by David Morin, and there are some parts that I do not understand from its derivation.

Note :
$V$ and $v$ is respectively the velocity of CM and a particle of the body relative to the fixed origin , while $v'$ is velocity of the particle relative to CM
From this, we can get : $$ v = V + v'$$

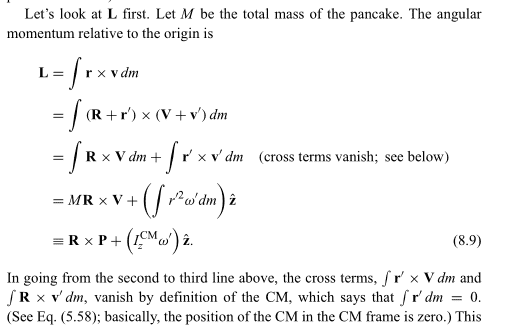
In the 3rd step, It is written that $\int r' \times V\, dm$ vanised because the position of CM in CM frame is zero.
But, isn't the position of CM should be calculated relative to the origin? (Since we are calculating the angular momentum relative to the origin, not CM)

 

[vanhees71]

Well, by definition if you are in the CM frame of reference the CM is chosen as the origin of your reference frame. Then also the reference point of the angular momentum is the CM.

 

[wrobel]

and is respectively the velocity of CM and a particle of the body relative to the fixed origin , while is velocity of the particle relative to CM

it is a very bad phrase
there can not be a velocity of one point relative other point
there can be velocity of a point relative a frame

 

[Rikudo]

it is a very bad phrase
there can not be a velocity of one point relative other point
there can be velocity of a point relative a frame

Ah...I see.Thanks for telling me!

Well, by definition if you are in the CM frame of reference the CM is chosen as the origin of your reference frame. Then also the reference point of the angular momentum is the CM.

Alright. So, $\int V \times r' \,dm$ use CM as its origin point, i suppose?

 

[Delta2]

Figure 8.4 says it all imho. $r'$ is the position vector in the frame of reference of CM, so when you calculate those terms (the ones that vanish) it is like you are in the frame of reference of CM, regardless if the whole calculation is done in the frame of reference of another point that is taken as origin , different than CM.
so in a nutshell it is
$$\int \vec{r'} dm=\vec{0}$$
$$\int \vec{r} dm=M\vec{R}$$

 

[Delta2]

Ok I might not have explained it very well but here is a better (i think) explanation.

From figure 8.4 it is $\vec{r'}=\vec{r}-\vec{R}$ hence integrating both sides we get $$\int\vec{r'}dm=\int\vec{r}dm-\int\vec{R}dm=M\vec{R}-\vec{R}M=0$$

 

[Rikudo]

It's nice to meet you again!

Ok I might not have explained it very well but here is a better (i think) explanation.

From figure 8.4 it is $\vec{r'}=\vec{r}-\vec{R}$ hence integrating both sides we get $$\int\vec{r'}dm=\int\vec{r}dm-\int\vec{R}dm=M\vec{R}-\vec{R}M=0$$

But, isn't $\int V \times r' \,dm = V\int r'\, sin\alpha$ since it is a cross product? (With $\alpha$ is the angle between vector$V$and vector $r'$).
Then, to find $\int r'\, sin\alpha$, we need to multiple both sides by $sin \alpha$ before integrating it.
$$\int\vec{r'} sin (\alpha)dm=\int\vec{r}sin (\alpha) dm-\int\vec{R}sin (\alpha) dm$$

 

[Delta2]

No , you confuse $$\int\vec{V}\times\vec{r'} dm=\vec{V}\times\int\vec{r'}dm$$ with $$\int|\vec{V}\times\vec{r'}|dm=|\vec{V}|\int|\vec{r'}|\sin a dm$$, the first above gives a vector as result(where in the integration opposite vectors cancel out), while the second gives a number greater than zero (where in the integration opposite vectors don't cancel out, because we take their magnitude).

 

[Rikudo]

No , you confuse $$\int\vec{V}\times\vec{r'} dm=\vec{V}\times\int\vec{r'}dm$$ with $$\int|\vec{V}\times\vec{r'}|dm=|\vec{V}|\int|\vec{r'}|\sin a dm$$, the first above gives a vector as result(where in the integration opposite vectors cancel out), while the second gives a number greater than zero (where in the integration opposite vectors don't cancel out, because we take their magnitude).

OK

 

[Rikudo]

it is like you are in the frame of reference of CM, regardless if the whole calculation is done in the frame of reference of another point that is taken as origin

So, we use the origin and CM frame of reference. but, how is it possible to use two different frames at the same time?