Translating reciprocating motion into a 45 degree rotation

[DefinitelyAnEnjinear]

TL;DR Summary

stuck on a problem of designing a mechanism translating linear to rotary motion; mechanisms like a crank are unsuitable for my purposes

I'm trying to design a mechanism to translate reciprocating motion into a 45 degree rotation.
Here's the idea:

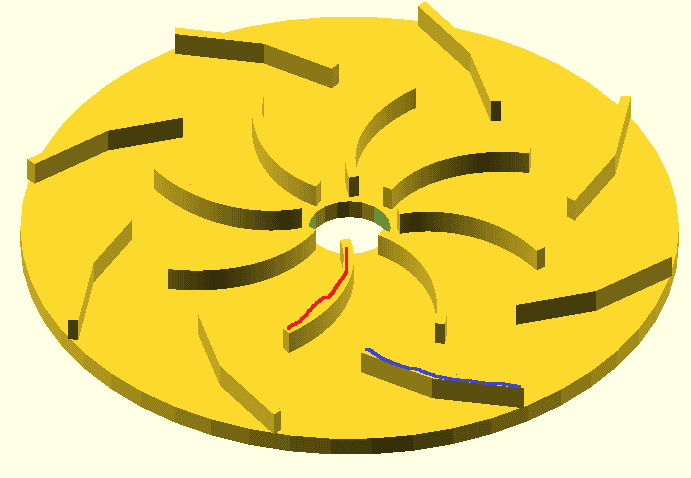
A pin will push against the part marked in red, causing part of the desired rotation. Then, when the pin is pulled back, its interaction with the blue part will complete a 45 degree rotation. since the object is rotationally symmetric, another back-and-forth motion will yield the same result

The problem with this particular design is that I don't know it actually leads to a 45 degree rotation. I designed and printed this just to make sure the idea will work with no idea how to optimally design something like this.

I am now slightly wiser thanks to this forum, but not wise enough.

I'm guessing the optimal design would use two segments of a logarithmic spiral (for the part marked in red and the part marked in blue), one with k=1 (to create a constant 45 degree angle on the forward motion) and one with k=-1 (for a 45 degree angle on the backward motion of the pin).

My main problem is... how do I position the second spiral segment (corresponding to the one marked in blue)?

I can think of a few constraints:

1. have a line from the center of the circle, going through both the end of the red segment (the part closest to the center) and intersecting the blue segment - to guarantee that the pin will interact with the blue segment on the backward motion
2. Have a line from the center of the circle, going through the end of the blue segment and the beginning of the next copy of the red segment - to guarantee that when the forward motion happens, we engage again

I can't think of a way to figure out the placement of the second segment while guaranteeing the result is a 45 degree rotation (ignoring the possibility that momentum from the rotation will lead to extra, undesired movement)

Why not use an existing mechanism, like a crankshaft with an 8:1 gear reduction?
Well, I have yet to come across a suitable mechanism that satisfies the condition that the part driving it be able to disengage from it (in the case of my design, the pin would leave the area of the disc once the 45 degree rotation is complete)

 

[Baluncore]

Maybe an escapement with 8 teeth.
Connect the reciprocating motion to the bottom of the pendulum or one side of the escapement.
https://en.wikipedia.org/wiki/Escapement

 

[DefinitelyAnEnjinear]

Maybe an escapement with 8 teeth.
Connect the reciprocating motion to the bottom of the pendulum or one side of the escapement.
https://en.wikipedia.org/wiki/Escapement

Interesting idea, but a pendulum would be a problem. I can push it, but I'd have to have a way to stop it so it would only complete 1 swing... I'd like to have control over when the movement occurs

 

[berkeman]

I'm trying to design a mechanism to translate reciprocating motion into a 45 degree rotation.

Can you say more about the source and characteristics of this linear displacement mechanism? Is an equal amount of force available in each direction? Is the amount of force available constant with linear displacement? How quickly and how often with this linear displacement cycle occur?

If the rotational motion is constrained to be in one direction (with a ratchet), then it should be pretty simple to make the linear rod push one of 8 pins on the wheel circumference to turn it 45 degrees before retracting and making contact with the next 45 degree pin on the circumference of the wheel to be ready for the next stroke.

 

[Baluncore]

Interesting idea, but a pendulum would be a problem. I can push it, but I'd have to have a way to stop it so it would only complete 1 swing... I'd like to have control over when the movement occurs

I do not suggest the pendulum be present, but what was the pendulum arm, would be the input. Movement would be the reverse of normal, the arm would be arranged to step the wheel. It becomes a simple double ratchet walking mechanism.

 

[DefinitelyAnEnjinear]

Can you say more about the source and characteristics of this linear displacement mechanism? Is an equal amount of force available in each direction? Is the amount of force available constant with linear displacement? How quickly and how often with this linear displacement cycle occur?

If the rotational motion is constrained to be in one direction (with a ratchet), then it should be pretty simple to make the linear rod push one of 8 pins on the wheel circumference to turn it 45 degrees before retracting and making contact with the next 45 degree pin on the circumference of the wheel to be ready for the next stroke.

I'd be using a mangle rack to create a linear motion, so I'm guessing the amount of force would be constant and equal in both direction. The speed doesn't really matter - if it needs to be slow, I can make it slow. as for how often, that would be dependent on the user.

I think I get what you and @Baluncore are talking about now. Seems like a good, simple idea. Thank you