Translation Invariance of Outer Measure .... Axler, Result 2.7 ....

[Math Amateur]

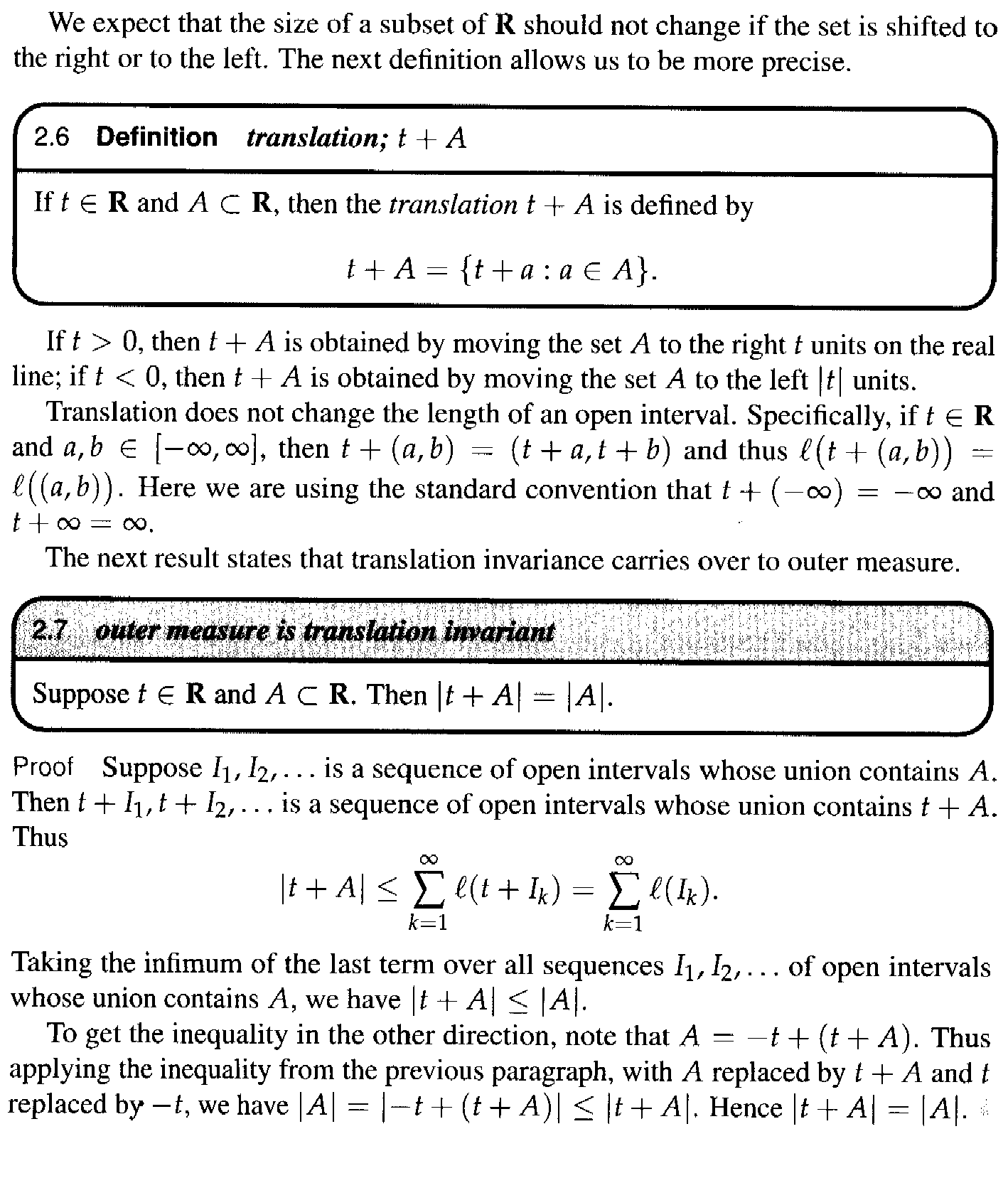
I am reading Sheldon Axler's book: Measure, Integration & Real Analysis ... and I am focused on Chapter 2: Measures ...

I need help with the proof of Result 2.7 ...

Result 2.7 and its proof read as follows:

In the above proof by Axler we read the following:

" ... ... Thus

... $\mid t + A \mid \leq \sum_{ k = 1 }^{ \infty } l ( t + I_k ) = \sum_{ k = 1 }^{ \infty } l ( I_k )$

Taking the infimum of the last term over all sequences $I_1, I_2, ... $ of open intervals whose union contains $A$, we have $\mid t + A \mid \leq \mid A \mid$. ... ..."Can someone please explain exactly how/why taking the infimum of the last term over all sequences $I_1, I_2, ... $ of open intervals whose union contains $A$, we have $\mid t + A \mid \leq \mid A \mid$ ... ?... Peter

 

[Opalg]

The inequality $|t+A| \leqslant \sum_{k=1}^\infty l(I_k)$ shows that $|t+A|$ is a lower bound for the set of sums of the form $\sum_{k=1}^\infty l(I_k)$. The inf of that set is by definition the greatest lower bound of the set. So any other lower bound, in particular $|t+A|$, is less than or equal to that inf.

Peter, it seems to me that most of your recent questions have been, in one form or another, instances of the general principle that "weak inequalities are preserved by limits": if every member of a set satisfies a weak inequality then the limit (or as in this case the sup or inf) of the set satisfies the inequality.

 

[Math Amateur]

Thanks for a most helpful reply, Opalg ...

I think you are correct ...but then I am not used to thinking of sup and inf in terms of limits ...

Peter