Transmission Line Model (TLM) End Resistance (Re)

[Azaxa]

Hi guys.

I'm looking into the modelling of a transmission line model (TLM) and feel that I'm understanding it fairly well. Although, one parameter keeps popping up with very little explanation as to what it actually is.

It seems that to characterize contact resistance for a e.g. GaAs TLM with AuGe ohmic contacts, that the contact resistance cannot just be characterized by the sheet resistance beneath the contact (Rsk) and the semiconductor resistance (Rsh) when these two are not equal. Many resources direct me towards Re in this case.

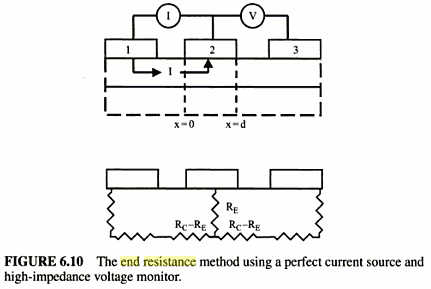
Now, the measurement of Re appears simple, with current flowing between two contacts the voltage is measured from the end contact and a neighbouring unloaded contact, contacts 2 and 3, and this value is divided by the current between contacts 1 and 2 (fig. 6.10).

)

It can be characterized as:

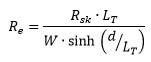
where
Rsk = Sheet resistance beneath ohmic contact,
W = width of the contact,
d = length of the contact,
Lt = transfer length (the length from the front of the contact to where the current is 1/e*io beneath the contact).

My question is:
-Is Re the resistance from Lt to the end of the contact?
-Is Re the resistance blocking current from dispersing from the contact to the next (unloaded) contact?

P.S. the reason I believe this is because if this is the case then and increase in Lt would reduce the area from Lt to end of the contact (x=d) whereas the beginning of the contact (x=0) will have a larger area, contributing to a decrease in contact resistance (Rc) but the "leftover" current after x=Lt will be more dense due to the decrease in area at the contact end. Of course, with greater with this will reduce the resistance of both Rc and Re and finally 1/sinh(d/Lt) or sech(d/Lt) shows that there's an exponential decrease in resistance due to the ratio that current density is shared (Rc/Re = cosh(d/Lt)).
I hope this helps with explanations.

Thanks, in advance.

 

[eljose79]

Hi there,

Thank you for your post and for sharing your thoughts on the measurement of contact resistance in a transmission line model. I can understand why this parameter may be causing some confusion for you.

To answer your questions, Re is not the resistance from Lt to the end of the contact. Instead, it is the resistance that blocks current from dispersing from the contact to the next (unloaded) contact. This resistance is also known as the transfer resistance.

The transfer length (Lt) is the length from the front of the contact to where the current is 1/e*io beneath the contact. It represents the distance over which the current spreads out from the contact and is affected by both the width and length of the contact.

As you mentioned, an increase in Lt would reduce the area from Lt to the end of the contact (x=d). This results in a decrease in contact resistance (Rc) because the current density is more concentrated in this smaller area. However, the decrease in area at the contact end also leads to an increase in transfer resistance (Re) because the current has a longer distance to travel before reaching the next contact.

The ratio of Rc/Re is important because it shows how the current density is distributed between the contact resistance and transfer resistance. As you mentioned, the formula 1/sinh(d/Lt) or sech(d/Lt) shows that there is an exponential decrease in resistance due to this ratio.

I hope this helps to clarify the concept of contact resistance and transfer resistance in a transmission line model. If you have any further questions, please don't hesitate to ask. Keep up the good work with your research!