Truss optimization and analysis

[rohmand]

We were given a small project to do before a test in my engineering statics class, i understand the goals of the project and understand the steps i need to take (i believe) however i don't understand how to simplify the distributed load into something i can anylize as we haven't seen anything like this in class and there's nothing in the book even comparible to it.

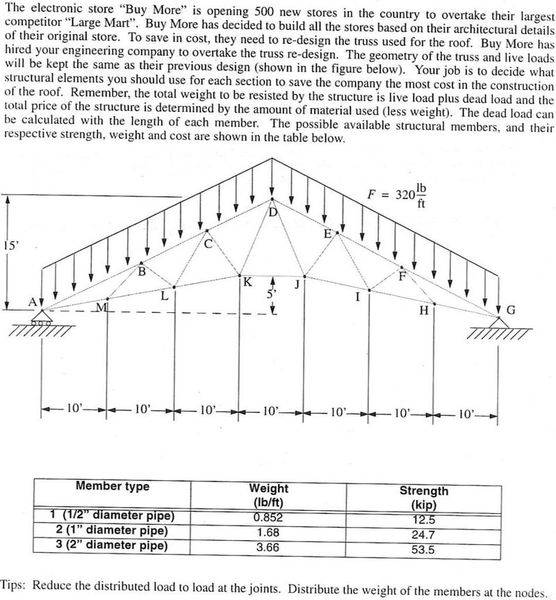
I understand obviously i need to break this down and see what kind of tension and compression i am putting on the truss members so that i can decide which types of tubes i need to design this truss with, what i don't understand is how do i turn this distributed load across the roof into a load i can understand as being put on each node, do i just find the Fr by using the integral and say i have a point load in the center of this truss of whatever that ends up being and if so would i say the per foot is per foot of x-axis or per foot along the roof line (hypotenuse)? the more i look at this thing the more i see more questions that i don't know the answer to or have multiple answers. i really want to understand this as it will help with being prepared for my test and i need to get this thing done so i can study for the rest of the material on my test. please any help pointing me in the right direction would be greatly appreciated. i searched for other posts similar to this but didnt find anything i could relate exactly which may be because i am missing a major step here, i did find this exact problem and actually used his link for the photo which hopefully works but nobody posted on his as well and i see that he came up with some point loads but rather than just use his info i would like to know if and why he is right?

 

[Studiot]

Well rohmand this is a homework question so you are supposed to start with your attempt, right or wrong.

We do not do the problem for you but try to guide you in the right direction.

You have identified a key issue, but think.
If you sum the distributed load and put an equivalent load at its centre will that coincide with any joints?
Why do you think the problem suggests avoiding loading except at joints?

Hints

Have you looked at a real roof and seen how the load is transferred to the trusses?

Look up the term purlin.

 

[rohmand]

well I've noticed that but that's my main problem i just have no idea how to apply the distributed load to each joint, i understand that that's why i need to break it down to then i could do a truss analysis by method of joints or method of sections, however we haven't done any of this stuff in class yet and its a group assignment but nobody in the groups understands what's going on and instead of waiting till the last second I am trying to find a starting point. and i DEFINATLY DO NOT want somebody to just do this and give me an answer i just need to know a starting point in how to apply this load so that i can use what i know about truss analysis.
also this is the only picture we were given so there are no purlins involved however in the real life model i understand that they would connect the trusses and so on, as i work construction and have built the physical model before.
ok so let me try to jump and see if i land anywhere good...
i understan that i want a force at the joint because i can use that in my equations however I am not sure how i would work with a point load put in the middle of a chord member, so with that being said would i integrate the distributed load across the length from joint to joint, ie 320 lb/ft * distAB and that would be my point force at point B then 320 lb/ft *distBC and that would be my force at C then continue to do that to have a point force on my joints then use sigma Fx=0, sigma Fy=0 and sigma Mg=0 to find my forces at G and A then continue to break the truss apart using the method of joints (as i understand it better than method of sections which I am still missing something as it just doesn't click for me yet)

 

[Studiot]

It seems to me that the true purpose (a good one) of this exercise is not to get numerical examples but to foster class discussion and understanding of structural action.

Is the roof symmetrical?
Is the loading symmetrical?

What does this imply?

If DEFG was an inclined plane, and you replaced the roof load with blocks at D, E, F & G what forces would be acting at these points? (not values just labels)

Is the geometrical information shown in your diagram sufficient to uniquely determine all the member lengths?

 

[rohmand]

yes its all symmetrical so the loads at the support reactions will be equal, and the above picture is missing some values i didnt realize that but the copy we were handed in class had them filled it B to C is 10 feet as is C-D and D-E, A-B is 15 foot as is F-G, would DEFG all be the same value? would G be a different value?
this is the part of this problem that has me scratching my head, so now since its an evenly distributed load could i simply sum 35'*320ft/lbs then divide by the number of joints the load is being distributed over? leaving me with 2800 point load per joint?
which as labels would be (distDtoG*F)/#of joints(4)=point force at each joint?
then once i have the point loads correct i can use the method of joints since i need to know all of the compression/tension ratings in the members, to find what force will be applied to each member and which pipe i can use, then i need to find a way to then redo the force problem adding in the weight of the pipes used as to give what the final loading would be and the magnitude of each force on each member

 

[Studiot]

If you take the interjoint distance eg AB and allocate half the distributed load to each joint then you can arrive at joint loadings.

So A gets 15F/2 and B gets 15F/2

However this is not the total at B. Can you complete the allocation?

It would help if you tried to answer the other questions I asked; they are all relevent.

If DEFG was an inclined plane, and you replaced the roof load with blocks at D, E, F & G what forces would be acting at these points? (not values just labels)

Why do you think the problem suggests avoiding loading except at joints?

 

[rohmand]

ah yes, after talking to a class mate today and working the problem in a couple ways knowing i was wrong but just trying to see if i can explain to myself why its wrong and how to correct it, anyway that being said yes i found that the force at B would equal 15F/2 + 10F/2, and i actually broke it down a step further into the length of that 15 foot piece being slanted which ends up being 16.32 feet, which isn't a very significant difference but its a difference all the same, and the 10 foot pieces end up being 10.88 feet
im not sure about your question about the loading at joints vs loading between them, i would think an answer to that would be that loading between joints will cause an odd moment calculation to the solution vs just being able to use cleaner calculations with the loads being applied at the joints

 

[Studiot]

the length of that 15 foot piece being slanted which ends up being 16.32 feet

I'm sorry I don't understand this.

Surely the 15 foot length is 15 feet? Or are you saying that 15 feet is the horizontal length so B is midway between M and L?

You need to specify more precisely, engineering is about attention to detail.

The point about loading only the joints is that your method of sections or joints is only valid if the loads are applied at the joints.
This is because the methods assume that there are no bending moments applied anywhere in the truss, so all the joints act as hinges and all the member forces are axial to their respective members (tension or compression only).
Note this does not say that forces acting don't exert moments, just that the moments everywhere sum to zero.

This allows you to make the assumption (and it is an assumption or model) that the loads are distributed as we described.

I was trying to get you to look at these loads and resolve them parallel to and perpendicular to the slope of the roof, as you would with the inclined plane.
This reduces the calculations since none of the axial forces in the members have any component perpendicular to themselves.

 

[rohmand]

hrmm well i see your point, and I've seen drawings done both ways, i would like to assume that your right and I am overthinking it and that the length of A to B is 15 foot however my TA told us that we would need to use the law of sines and the inverse tan function to find that angle and the real length of A to B, i may need to find my teacher around and ask him as this has become a confusing point in this problem. also i did not say it well but yes i was trying to say something along the lines of what you have said about the loads only being applied to the joints because of a moment force in the middle of the member since this does not work for the equations we have at the time. and yes i understand the moment that will react about the truss itself even with the distributed load changed into a point load about the joints, i started working the problem some more last night and ran into the problem about having no vertical or horizontal members which is making my equations extremely messy and hard to look at, i had not previously thought about how to look at this problem and change this force to be perpendicular to the slope of the roof however i will take some time and try to view this problem as such and get back to you with my ideas and how i think i can manage that and see if you think I am going down the right path or not. thank you for your help this far and sorry i haven't been very clear in my posts, my mind is getting warped by this problem and studying for the test but ill try to compose my words a little more clearly as to get my point across a little better.