Twin paradox, virtual clock on ship with Earth time, discontinuity

[LikenTs]

TL;DR Summary

Twin paradox, special relativity, general relativity, computerized clock in ship showing time on earth.

Conventional Twin Paradox. A ship with speed v = 0.8c makes a round trip from Earth and back. It lasts 6 years and on earth 10 years have passed.

The ship carries its own clock and also a computerized clock that always shows the time on earth at that moment.

It is about knowing the T - T' graph that the computer clock in the ship shows during the trip. The events to follow are (t,x=0), the ticks of the clock in earth frame, which must have coordinates in any other frame.

In the simplified problem, instantaneous accelerations, with SR calculations, the graph could look like this:

This graph represents several problems for me. One is the temporary discontinuity in the middle of the trip. The computerized clock that shows the time on earth would instantly change from marking about 2 years to marking about 8. And also this depends of pilot deciding to return. if he decides to continue flying away from Earth the computerized clock should not jump. All of this seems unphysical.

So I try to think of a more realistic graph, which takes into account the accelerations and following assumptions:

1. Every event, the ticks of the clock on earth, must have coordinates in other frames and its evolution must present continuity.
2. The accelerations (start, stop, return, stop) are symmetrical and must contribute equally to the graph.

And it seems to me that a realistic graph, with physical sense, should be like this.

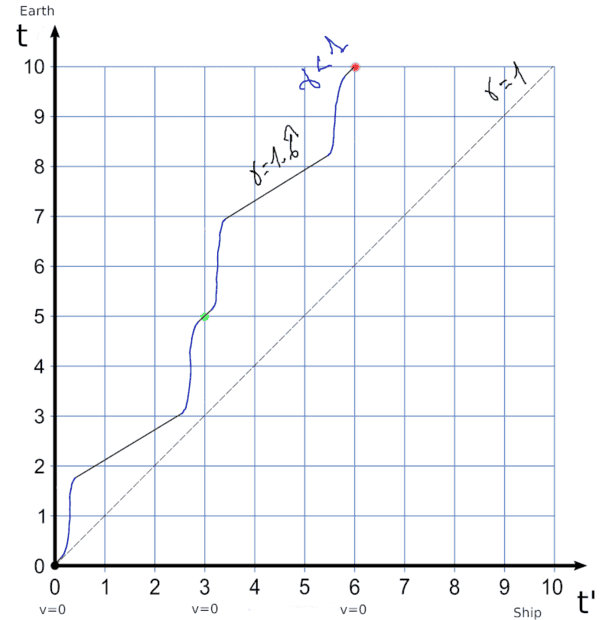
During the accelerations the slope is greater than 1. This indicates that the ship sees in the computerized clock that the time on earth is increasing very fast during the acceleration. This seems to be in accordance with GR equivalence principle. An acceleration, like a gravitational field, objectively slows down clocks.

When the ship is already going at a constant speed, the slope is less than 1. This indicates that it sees dilation in terrestrial time according to the Lorentz transformation.

When it stops halfway, the slope is 1 and the terrestrial clock follows the same rate.

However, according to SR, the acceleration does not influence, only the path and speed, and therefore there can be no slopes greater than 1 in this graph.

So I don't understand which of my assumptions is wrong. I would like to know what would be the right, realistic T-T' graph, the computerized clok in ship, in the twins paradox.

 

[Dale]

The ship carries its own clock and also a computerized clock that always shows the time on earth at that moment.

In which frame is “at that moment” computed?

The computerized clock that shows the time on earth would instantly change from marking about 2 years to marking about 8.

Why? Please post your derivation. Or at least the calculation that the computerized clocks are performing.

However, according to SR, the acceleration does not influence, only the path and speed, and therefore there can be no slopes greater than 1 in this graph.

Why? Where does this limit come from? Again, show your derivation.

 

[Ibix]

All of this seems unphysical.

I recently travelled to Europe from the UK and had to change my watch when I arrived. That's all that's happening at the discontinuity - you changed your convention for zeroing the clock. It's a convention change not a physical one, so "unphysical" doesn't really enter into it .

You can come up with better coordinate systems where the time change is smooth even with a discontinuous speed if you want. Radar coordinates are a good choice. But the fundamental point is that relativity gives you a lot of flexibility to define what you mean by "at the same time" when two events are not in the same place, and patching together two of the standard-issue inertial frame definitions of "at the same time" doesn't do a very good job of it.

 

[PeterDonis]

Conventional Twin Paradox.

Please read this, and also the Usenet Physics FAQ article referenced:

https://www.physicsforums.com/threads/when-discussing-the-twin-paradox-read-this-first.1048697/

 

[Nugatory]

The computerized clock that shows the time on earth would instantly change from marking about 2 years to marking about 8.

Yes.
The computerized clock on the ship is telling you what the earth clock is reading at the same time that we on the ship are looking at the computerized clock. Relativity of simultaneity (if you are not familiar with that concept, stop right now and learn about it - it is essential) means that the definition of "at the same time" is different depending on the speed of the ship relative to the earth. Changes in that relative speed can can produce large changes in the definition of "at the same time" and therefore large swings in the time displayed by the computerized clock on the ship - the vertical line you see in your first graph is the result of the computer clock switching from the "earth receding at .8c" definition to the "earth approaching at .8c" definition instantaneously.

Obviously that line has a slope greater than 1, but all that means is that our notion of "at the same time" changed instantaneously. If we do a more physically realistic version without the instantaneous acceleration we get a line that's not quite vertical, but its slope can still be much greater than 1 (vertical displacement the same 6-odd years, horizontal displacement much less than that). So your second graph is fine; your assumption that the slope cannot exceed 1 is wrong.

Two further thoughts:
1) You are calling the device on the ship a "computerized clock", but it's not measuring time, it's displaying the results of a moderately complicated calculation starting with the regular clock on the ship as input
2) Google for and read about the "Andromeda Paradox", a somewhat more approachable example of how small changes in relative speed can lead to large changes in teh definition of "at the same time" when working with distant objects.

 

[Vanadium 50]

I recently travelled to Europe from the UK and had to change my watch when I arrived.

I think the UK is still part of Europe, at least geologically and geographically, but in any event, they have computerized watches that will do that for you. You land, it figures out what country it is in, and adjusts. I don't think it works in flight, but if it did, it would be the perfect analogy.

 

[FactChecker]

Here is another thing you might want to consider in your initial scenario. Consider the inertial reference frame of the return flight. An observer, O3, in that frame has witnessed the spaceship-twin aging much less than the Earth-twin on his outbound flight because the relative velocity of the spaceship was twice as high. Then the spaceship-twin suddenly turns around and says that the Earth-twin is younger. Why should O3 believe that? He has his own understanding of the reality of the Earth-twin's age.
Using a non-inertial reference frame to calculate ages in other frames is more complicated.

 

[obtronix]

And it seems to me that a realistic graph, with physical sense, should be like
However, according to SR, the acceleration does not influence, only the path and speed, and therefore there can be no slopes greater than 1 in this graph.

So I don't understand which of my assumptions is wrong. I would like to know what would be the right, realistic T-T' graph, the computerized clok in ship, in the twins paradox.

Your graphs both look correct, in special relativity a change in the ship's inertial frames will cause a time jump in "earth time", the fact that acceleration caused it is kind of irrelevant it's the change in inertial frame that matters. It results in a path change and a slope greater than 1.

This is caused by the ship's hyperplane of simultaneity abruptly changing from earth's past to earth's future.

Now realistically a slower acceleration will cause a continuous change in inertial frames, and the Earth clock will speed up (from the ship's perspective) instead of jumping. But the end result is identical.

 

[FactChecker]

the fact that acceleration caused it is kind of irrelevant it's the change in inertial frame that matters.

One might argue that a change in inertial frames is equivalent to acceleration.

 

[Ibix]

One might argue that a change in inertial frames is equivalent to acceleration.

Not really. You can choose to change frames without accelerating, and you can use a single inertial frame even though you accelerate.

We do both all the time: Einstein's "what time does Oxford stop at this train" is a strict use of his rest frame and sounds wrong because in that particular context we routinely use the Earth rest frame even when we are changing speed in that frame. And in a train you might observe that you are doing 60mph and also that your coffee cup is stationary on your table, using two different inertial frames in one sentence with no acceleration.

 

[Ibix]

Incidentally, there's more fun to be had with clocks on the ship that are "synchronised" in this way with clocks at rest in the Earth frame but further from Earth than the turnaround point. They will jump backwards at turnaround. Even with an arbitrarily slow smooth turnaround you can find clocks to synchronise with such that your shipboard clock will tick backwards during the turnaround.

That's a really big clue that this is all convention changes and nothing that affects anything.

 

[Ibix]

Your graphs both look correct,

I don't think the second one is correct at all, unless he's doing something a lot more complex than I think he is. His tick rate initially increases, which isn't consistent with his previous graph at all. And the turnaround ought to produce a much larger shift in the remote clock time than the initial acceleration and final deceleration do, because it produces the same "angle" change in the plane of simultaneity but is much further from Earth than those accelerations. And with a finite acceleration phase he'd have to cruise at above 0.8c to achieve the same overall 6:10 aging ratio.

That's assuming he's doing something like using momentarily comoving inertial frame simultaneity (which additionally has the backwards in time issue I mentioned above).

@LikenTs: a more useful thing to note is that there is no unique answer to "what time is it on Earth". For your original instantaneous acceleration case, see the graph below. Literally any curve that connects (0,0) to (6,10), stays within the blue bounded region, never goes left, and never goes down, is as good as any other. All such curves have the property that, looking through a telescope, Earth will never see the clock "synchronised" like that to be ahead of their own clocks, and nor will the ship ever see the Earth's clocks ahead of their "synchronised" clocks. And all agree on the initial and final times which are the only actual invariants.

(Apologies for lack of labelling - the scales are as per your first graph, with ship time horizontally and time on Earth "now" vertically.)

 

[FactChecker]

Not really. You can choose to change frames without accelerating, and you can use a single inertial frame even though you accelerate.

Good point. I should have been much more precise in my statement. Can you be stationary in one inertial frame and change to remain stationary in a different inertial frame that is moving wrt the first without accelerating?

 

[Ibix]

Good point. I should have been much more precise in my statement. Can you be stationary in one inertial frame and change to remain stationary in a different inertial frame that is moving wrt the first without accelerating?

Not in flat spacetime, no, but that is not the point here. You can always make those time skips (both forward and backward) happen by deciding to use a different frame and programming your "synchronised" clock accordingly, whether you accelerate or not. If the traveller does that and keeps track of the changes correctly they will still end up with the ten year elapsed time. All the acceleration does in this scenario is provide the traveller with a natural reason to use a new inertial frame, rather than doing it for the mental exercise.

 

[Sagittarius A-Star]

View attachment 330776
However, according to SR, the acceleration does not influence, only the path and speed, and therefore there can be no slopes greater than 1 in this graph.
So I don't understand which of my assumptions is wrong.

You must distinguish between proper acceleration of a twin and acceleration of a reference-coordinate system.

• The proper acceleration of a twin does not influence directly the age difference when both twins meet again.
• The proper time of the earth twin can also tick faster than the coordinate-time of the accelerated reference frame (rest frame of the space ship). Slopes can be greater than 1 for a non-inertial reference frame.

2. The accelerations (start, stop, return, stop) are symmetrical and must contribute equally to the graph.

No. The second graph is wrong with regards to this.

• The pseudo-gravitational time dilation of the earth-twin in the rest frame of the space ship leads to a slope smaller than 1 at start and the second stop, because in both cases the space ship's rest frame is accelerating away from earth. Additionally, in both cases the pseudo-gravitational time dilation is insignificant because of the small distance between earth and ship.
• The pseudo-gravitational time dilation of the earth-twin in the rest frame of the space ship leads to a slope greater than 1 at the first stop and return, because in those two cases the ship frame is accelerating towards earth. In those cases, the earth is "above" the ship in the pseudo-gravitational potential.

 

[LikenTs]

Thanks for the responses and sorry for the delay. I have been studying accelerated frames in SR, to understand the key factor that indicated @Sagittarius A-Star, the importance of the sense of accelerations and dependence on distance.

So, this would be roughly the earth display clock on the ship, with non-instantaneous accelerations. The accelerations that matter are the far ones.

Graph 1

If the ship also has a clock display for star 2 time, the destination at 4ly, synchronized previously with Earth, it would be.

Graph 2

In this case, it can be seen that the accelerations that matter, the ones that involve jumps, are the starting ones that are far away. Green point is arriving to star 2, red point arriving to Earth.

So, for me the conclusion would be.

Corollary 1: If you accelerate towards some destination in space, you see that its time jumps, or rapidly increases to, according to the formula DT = L v/c. (Being DT time jump in years, L the distance to the destination in light years and v the speed delta. )

If for example you accelerate to almost the speed of light towards a destination at 4ly, its time (its display clock, according to you) would jump to 4 years, which seems logical. Because you would arrive almost instantly at your destination and you should not arrive at an earlier time than that.

But now comes the most interesting, which @Ibix commented. If we have another third star aligned with Earth and the destination star, but much further away, which has its time synchronized with the earth- and star 2, and the ship also has a clock display to know the time on the star 3, its graph would be

Earth-Star 1 --- 4ly --- Star 2 -- 396 ly -- Star 3

Graph 3

That is, during the turn around back to Earth on star 2, the clock display in the ship for the star 3 goes back in time. This is like aborting the trip to the star 3.

So

Corollary 2: If you abort the trip to a destination in space, its time jumps back according to the same DT = L v/c relationship.

Finally, if Earth (and star 2 and 3) have a clock display for the time in the Ship, it would be

Graph 4.

That is, for "stationary" observers (inertial at rest) in space, they see a time dilation of travelers continuously, where accelerations do not matter. However, for the traveler, acceleration is essential.

My interpretation, physical or philosophical, is that even with a speed limit to communications, Nature allows fast travel in proper time, but the traveler must synchronize with universal time during accelerations, although "stationary" observers see a continuous Lorentz Time dilation. With stationary and universal time I mean objects at low velocity with respect to the microwave background, and away from intense gravitational fields.

 

[Dale]

@LikenTs all of your graphs seem to be random squiggles with no reason, justification, or explanation. Please answer my previous questions before proceeding.

In which frame is “at that moment” computed?

Why? Please post your derivation. Or at least the calculation that the computerized clocks are performing.

Why? Where does this limit come from? Again, show your derivation.

 

[PeroK]

So, for me the conclusion would be.

Corollary 1: If you accelerate towards some destination in space, you see that its time jumps, or rapidly increases to, according to the formula DT = L v/c. (Being DT time jump in years, L the distance to the destination in light years and v the speed delta. )

More simply, this is a change in your standard (Einstein) synchronisation when you change your inertial reference frame.

You don't have to physically accelerate to adopt a new inertial reference frame.

If for example you accelerate to almost the speed of light towards a destination at 4ly, its time (its display clock, according to you) would jump to 4 years, which seems logical. Because you would arrive almost instantly at your destination and you should not arrive at an earlier time than that.

Okay.

But now comes the most interesting, which @Ibix commented. If we have another third star aligned with Earth and the destination star, but much further away, which has its time synchronized with the earth- and star 2, and the ship also has a clock display to know the time on the star 3, its graph would be

Earth-Start 1 --- 4ly --- Star 2 -- 396 ly -- Star 3

Graph 3

View attachment 331075
That is, during the turn around back to Earth on star 2, the clock display in the ship for the star 3 goes back in time. This is like aborting the trip to the star 3.

So

Corollary 2: If you abort the trip to a destination in space, its time jumps back according to the same DT = L v/c relationship.

Again this is simply a change of synchronization associated with a change in inertial reference frame.

Finally, if Earth (and star 2 and 3) have a clock display for the time in the Ship, it would be

Graph 4.

View attachment 331081That is, for "stationary" observers (inertial at rest) in space, they see a time dilation of travelers continuously, where accelerations do not matter. However, for the traveler, acceleration is essential.

I'm not sure what you mean by this.

My interpretation, physical or philosophical, is that even with a speed limit to communications, Nature allows fast travel in proper time, but the traveler must synchronize with universal time during accelerations, although "stationary" observers see a continuous Lorentz Time dilation. With stationary and universal time I mean objects at low velocity with respect to the microwave background, and away from intense gravitational fields.

SR deals with flat spacetime in the absence of gravity. The Lorentz transformation is not dependent on choosing as a baseline the universal reference frame in which the CMBR is isotropic. Gravity and the CMBR are essentially irrelevant to SR.

IMO, you are focusing on the wrong aspects here. SR is a theory of flat spacetime and the transformations between inertial reference frames.

 

[LikenTs]

In which frame is “at that moment” computed?

In Ship frame, Graphs 1, 2, 3, in Earth Frame Graph 4.

Why? Please post your derivation. Or at least the calculation that the computerized clocks are performing.

Let's assume a simple case, in which a Lorentz boost can be applied.

Each tick of the clock on earth is an event of coordinates (t,0) in the frame of the Earth. Applying the Lorentz boost this corresponds to an event (t',x'). Then the t-t' graph is plotted. The time on Earth according to the frame of the ship at that moment in the ship.

Only in this case we cannot apply directly a Lorentz boost because we are following movements with accelerations.

Why? Please post your derivation. Or at least the calculation that the computerized clocks are performing.

Almost based on these calculations:

https://arxiv.org/pdf/1807.02148.pdf
https://guyleckenby.weebly.com/uploads/5/6/2/9/56292217/final_draft.pdf (Especially the formula on page 4, to calculate time jumps)

 

[LikenTs]

Again this is simply a change of synchronization associated with a change in inertial reference frame.

I'm not sure what you mean by this.

SR deals with flat spacetime in the absence of gravity. The Lorentz transformation is not dependent on choosing as a baseline the universal reference frame in which the CMBR is isotropic. Gravity and the CMBR are essentially irrelevant to SR.

IMO, you are focusing on the wrong aspects here. SR is a theory of flat spacetime and the transformations between inertial reference frames.

I understand that from a mathematical point of view it can be considered that way. Also @Ibix compared it with the time change when coming to Europe (continental). Conventions, frame changes, ... but the fact is that he does not return to the UK younger than his peers. SR, physically, is more than reference frames or conventions. It implies that a traveler returns younger. And what he has made differential is to accelerate, something absolute regardless of the frame you choose, and equations indicate that in proper frame time adjustments occur during accelerations. And right, SR, the mathematical model, has nothing to do with the CMBR.

 

[Ibix]

SR, physically, is more than reference frames or conventions.

Indeed. But "how old is the home twin now according to the travelling twin" is inescapably about reference frames (or at least the simultaneity conventions thereof).

And what he has made differential is to accelerate, something absolute regardless of the frame you choose,

True.

and equations indicate that in proper frame time adjustments occur during accelerations.

False, unless you pick a frame that makes it so.

 

[PeterDonis]

It implies that a traveler returns younger. And what he has made differential is to accelerate

Please read the link I gave in post #4. In particular pay attention to the spacetime geometry explanation.

 

[PeterDonis]

equations indicate that in proper frame time adjustments occur during accelerations

More precisely, frame-dependent equations indicate that "time adjustments" occur during accelerations.

The key point you are missing is that there is a difference between the statement "the traveler returns younger", which is indeed an invariant as the traveler and his stay at home twin can stand right next to each other and compare their respective ages, and "time adjustments occur" while the twins are not right next to each other but spatially separated, perhaps by many light years. The latter "adjustments" are frame dependent and indeed have no physical meaning; they are just calculations based on abstract assumptions about coordinates.

Another part of what I linked to in post #4 that you might want to seriously consider is the Doppler Shift explanation in the Usenet Physics FAQ article. That explanation relies only on direct observables--the directly observed Doppler shift of light signals sent between the twins--and it does not require any "time adjustments" at all. It focuses on what each twin actually sees with their eyes (or telescope) the other twin's clock doing during the trip.

 

[PeroK]

I understand that from a mathematical point of view it can be considered that way. Also @Ibix compared it with the time change when coming to Europe (continental). Conventions, frame changes, ... but the fact is that he does not return to the UK younger than his peers. SR, physically, is more than reference frames or conventions. It implies that a traveler returns younger. And what he has made differential is to accelerate, something absolute regardless of the frame you choose, and equations indicate that in proper frame time adjustments occur during accelerations.

We have this discussion frequently. The belief that "when you accelerate something weird happens to time" is false. The twin paradox is fundamentally about spacetime geometry. Acceleration is a side issue.

You can have the equivalent of the twin paradox without acceleration. Simply by measuring different inertial paths through spacetime. It's actually the Minkowski equivalent of the triangle inequality in Euclidean geometry.

 

[LikenTs]

We have this discussion frequently. The belief that "when you accelerate something weird happens to time" is false. The twin paradox is fundamentally about spacetime geometry. Acceleration is a side issue.

You can have the equivalent of the twin paradox without acceleration. Simply by measuring different inertial paths through spacetime. It's actually the Minkowski equivalent of the triangle inequality in Euclidean geometry.

OK. It was not my intention to enter into trite debates, although from the references that I put above I think that I am not alone in the interpretacion.

My main question, summarized, was the following. We have built the ship. The crew asks for a clock display to always know the time on Earth (According to their simultaneity criteria)

Then the following questions arise.

1. Physicists Academy: That is possible and the function is perfectly defined. Or, it is not possible, it does not make sense, you can choose any function that in the end matches.

2. If 1 affirmative. Is it possible to program this clock display only with a local clock and an accelerometer?, and regardless of the trips that the ship finally makes before returning to Earth and both clocks coincide?. Would graphs 1,2,3 approximately correct?.

Another part of what I linked to in post #4 that you might want to seriously consider is the Doppler Shift explanation in the Usenet Physics FAQ article. That explanation relies only on direct observables--the directly observed Doppler shift of light signals sent between the twins--and it does not require any "time adjustments" at all. It focuses on what each twin actually sees with their eyes (or telescope) the other twin's clock doing during the trip.

Thank you. I was reading several previous questions on the subject as well, and I started a new thread because I think, maybe I'm wrong, that the T-T' graphs approach was novel. I think the graphs above are compatible with Doppler analysis, once the speed of light is discounted.

 

[Ibix]

That is possible and the function is perfectly defined

If you specify a simultaneity convention then yes it is tautologically possible, although the result may include positive and negative jumps depending on how sensible the criterion is. There are infinite choices of valid simultaneity criterion, most of which will give different answers.

Is it possible to program this clock display only with a local clock and an accelerometer?

No. You need an accelerometer and at least a memory of all past accelerations (the velocity in the Earth frame before the most recent acceleration will do if we're restricting this to instantaneous accelerations) in order to determine the current velocity and position of Earth in the chosen coordinate system. Acceleration alone won't do.

Suppose you use Einstein synchronization, and the current rate of your synchronised clock is $1/\gamma(v)$. Then you accelerate such that your speed has changed by $v$. Is the new clock rate $1$ or $1/\gamma(v')$ where $v'=2v/(1+v^2/c^2)$? Without knowing the sign of $v$ you can't know if you increased speed or decreased relative to Earth. That information is not held in the clock rate. And I haven't even mentioned the time skip - for that you need to know $x$ as well.

 

[PeroK]

You can programme a clock to do anything. The key point is that simultaneity is not a physical thing. It's a coordinate convention.

One of the issues with the acceleration solution is that you get essentially the same answer with two or four acceleration phases.

In the first case you accelerate away from Earth, travel for four years ship time; decelerate and accelerate back towards Earth; then decelerate when you approach Earth. That's four phases.

In the second case, you get up to full speed, then synchronise your clock with Earth as you fly past. The turnaround accelerations are then the same as the first case, but at the end you compare clocks with Earth as you fly past without decelerating. That's only two phases.

The two scenarios give approximately the same result. The only difference is that in the first case the relative speed was less at the start and end.

If acceleration was really the prime cause of differential aging, you would expect these two cases to be significantly different.

That they are not suggests that acceleration is not the critical factor that some believe.

 

[PeterDonis]

It was not my intention to enter into trite debates

This attitude is not constructive. You have multiple experts who are trying to help you. If you don't want to listen to us, or if you consider our points "trite", then we might as well just close this thread as it is wasting everyone's time.

 

[PeterDonis]

The crew asks for a clock display to always know the time on Earth (According to their simultaneity criteria)

And the proper answer to this question is, first, why do they care? And second, "their simultaneity criteria" is not well-defined--and they'd better be really careful trying to make it well-defined, because the obvious ways of doing it (which I suspect you are implicitly using) don't work--you end up having "the time on Earth" go backwards during some portions of the trip (basically whenever you accelerate hard enough away from Earth).

I was reading several previous questions on the subject as well

Which ones?

I started a new thread because I think, maybe I'm wrong, that the T-T' graphs approach was novel.

It's not--lots of people come up with a similar idea. And then run up against all of the reasons why it isn't really a good idea.

I think the graphs above are compatible with Doppler analysis, once the speed of light is discounted.

Any graphs made with valid coordinate charts will be "compatible" with the Doppler analysis. (If the graph is made using an inertial frame, you can just draw in the 45 degree lines corresponding to the light signals, as is done in the Usenet Physics FAQ article I referred to. In non-inertial frames you have to be a lot more careful because the worldlines of light rays are no longer always 45 degree lines.)

The question is why you would bother making all these graphs when the Doppler analysis shows you, directly, in terms of what you actually see with your eyes (or through your telescope) of the other twin's clock, why the final relative ages come out the way they do. Since you don't need all these extra graphs to get and explain the right answer, what are they for?

 

[Peter Strohmayer]

You can have the equivalent of the twin paradox without acceleration.

I am not sure what is meant by "equivalent".

A twin can never return younger unless he has been accelerated.

The age difference is due to
- the timespans of uniform relative speeds
and
- the accelerations (kinked world lines have no physical reality).

The acceleration is of fundamental importance for me, because a "paradox" can only occur with accelerated motion, but not only with uniform motion.

 

[PeterDonis]

I am not sure what is meant by "equivalent".

He means having the twins age differently when they come back together.

A twin can never return younger unless he has been accelerated.

This is only true under a very restricted set of circumstances: spacetime must be flat, and you must insist that there are only two twins (i.e., scenarios like an outgoing traveler synchronizing his clock with an incoming traveler at the "turnaround" point are rule out) and that the stay at home twin is inertial the whole time. The rule does not generalize to scenarios where those conditions are not met.

The spacetime geometry rule, OTOH, which @PeroK has mentioned and which is discussed in more detail in the article I linked to in post #4 and its references, always works, no matter whether spacetime is flat or curved, no matter how many travelers there are, and no matter who is inertial and who is not.

Given these facts, @PeroK is perfectly correct that focusing on the acceleration is misplaced. The correct focus is on spacetime geometry and arc lengths along timelike curves in that geometry.

 

[Ibix]

This is only true under a very restricted set of circumstances: spacetime must be flat

And topologically trivial (just to add a further restriction). You can have a twin paradox with two eternally inertial twins in a cylindrical universe.

 

[Ibix]

But even in curved space-time it seems to be true that uniform motion does not cause a "paradox"?

In curved spacetime two inertial observers may meet more than once. Their elapsed times between meetings may be different.

 

[PeroK]

PS note that the maximum differential aging (for a given velocity) is achieved in the non-acceleration scenario. That is to say, the proper time in the purely inertial scenario (at some speed $v$) where the sum of two clock readings is taken will be less than the scenarios where there are two or four acceleration phases (up to the cruising speed $v$). In the latter scenarios, the clock travels at a lower relative speed for some of the journey. Although, as above, the difference can be made arbitrarily small.

This illustrates that the need for proper acceleration in the case where a single clock is used actually reduces the total differential aging, below a theoretical maximum - which can be achieved using two clocks.

This also illustrates that acceleration is actually a physical constraint of working with a single clock on both legs of the journey. And not a fundamental aspect of proper time measurements along different spacetime paths.

 

[Dale]

It took me a while to respond to this because I wanted to go through the paper you cited.

In Ship frame, Graphs 1, 2, 3, in Earth Frame Graph 4.

Let's assume a simple case, in which a Lorentz boost can be applied.

You can never use a Lorentz boost either to or from the ship frame. The ship frame is non-inertial and a Lorentz boost is a transform between two inertial frames. This is exactly what I was concerned that you were doing from your initial post.

You can use a Lorentz boost between the earth frame and any of the momentarily co-moving inertial frames of the ship. But none of the momentarily co-moving inertial frames are the ship's frame since the ship is not inertial and does not remain at rest in any of them.

Almost based on these calculations:

https://arxiv.org/pdf/1807.02148.pdf
https://guyleckenby.weebly.com/uploads/5/6/2/9/56292217/final_draft.pdf (Especially the formula on page 4, to calculate time jumps)

One thing to be aware of is that neither of these papers appear to be in the professional scientific literature. The first one appears to have been stored in arxiv for 5 years but still not accepted in any journal. It has some problems that makes me suspect that it will not be accepted. The second one appears to be a student's term paper for a class, so I don't think there was even an attempt to publish it. You should always be skeptical of such references. They are only valid if they are consistent with the rest of the scientific literature, which is difficult to know at the beginning.

Both papers have the same problem. Specifically, neither one actually defines the ship's frame. They both use some Lorentz transforms and some Rindler transforms and they try to hodgepodge together something that they claim represents the home time in the ship's frame, without actually constructing the ship's frame. The problem is that this hodgepodge produces a mapping that fails to be smooth and invertible. As such, it is not a valid reference frame and thus has no claim to represent anyone's perspective including the ship's perspective.

The problem is that although the requirement that any reference frame must be smooth and invertible does eliminate the hodgepodge approach, that requirement does not lead to a unique reference frame. In particular, there are an infinite number of different reference frames that disagree on simultaneity but would all be valid reference frames for the ship. All of those equally can claim to represent the ship's perspective, but they would give different plots, even for the same acceleration profile.