- #1
aheight
- 321
- 109
Hi,
I've computed 512 terms of a power series numerically. Below are the first 20 terms.
$$
\begin{align*}
w(z)&=0.182456 -0.00505418 z+0.323581 z^2-0.708205 z^3-0.861668 z^4+0.83326 z^5+0.994182 z^6 \\ &-1.18398 z^7-0.849919 z^8+2.58123 z^9-0.487307 z^{10}-7.57713 z^{11}+3.91376 z^{12}\\
&+19.6052 z^{13}-12.717 z^{14}-40.7957 z^{15}+40.3343 z^{16}+66.9766 z^{17}-133.148 z^{18}\\
&-78.9037 z^{19}+\cdots
\end{align*}
$$
And wish to show the radius of convergence is approx ##0.574342##. The Ratio test fails as successive ratios are finite but do not tend to a limit as shown in the plot below and I cannot use the Alternating Series test either or integral test or other convergence test I am aware of.
I was wondering if someone could suggest another method I could use to suggest experimentally that this series is appearing to converge (conditionally) in the indicated domain?
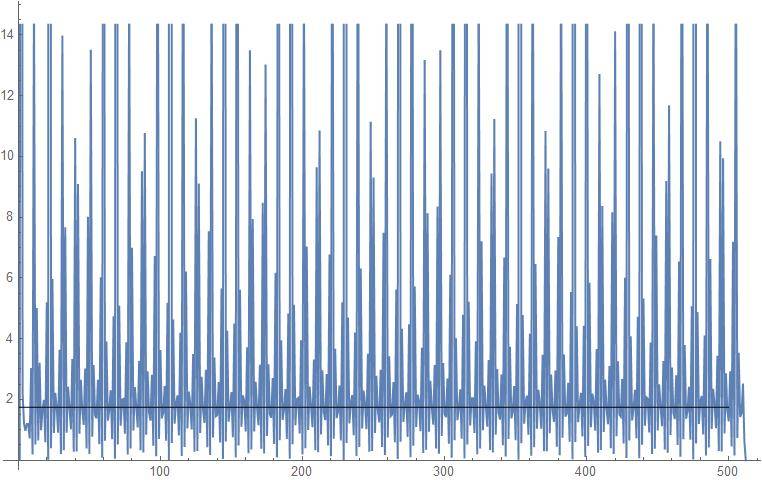
I've computed 512 terms of a power series numerically. Below are the first 20 terms.
$$
\begin{align*}
w(z)&=0.182456 -0.00505418 z+0.323581 z^2-0.708205 z^3-0.861668 z^4+0.83326 z^5+0.994182 z^6 \\ &-1.18398 z^7-0.849919 z^8+2.58123 z^9-0.487307 z^{10}-7.57713 z^{11}+3.91376 z^{12}\\
&+19.6052 z^{13}-12.717 z^{14}-40.7957 z^{15}+40.3343 z^{16}+66.9766 z^{17}-133.148 z^{18}\\
&-78.9037 z^{19}+\cdots
\end{align*}
$$
And wish to show the radius of convergence is approx ##0.574342##. The Ratio test fails as successive ratios are finite but do not tend to a limit as shown in the plot below and I cannot use the Alternating Series test either or integral test or other convergence test I am aware of.
I was wondering if someone could suggest another method I could use to suggest experimentally that this series is appearing to converge (conditionally) in the indicated domain?
Attachments
Last edited:



