- #1
amenhotep
- 29
- 1
Hello,
I'm having problems understanding what exactly is meant by a conservative vector field. So, allow me to explain how I currently understand it.
Let [itex]\overrightarrow{F}[/itex] be some sort of a vector field that is conservative. The stream plot of [itex]\overrightarrow{F}[/itex] is shown below.
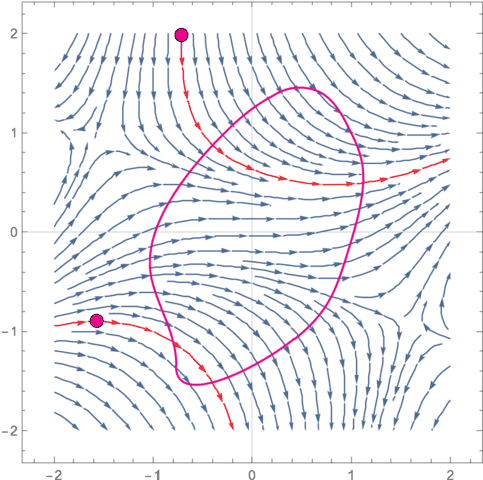
From my understanding, for a particle that interacts with field, the streamlines show the direction of flow of the particle. I have colored two streamlines in red to illustrate that. So, any particle placed at any location in the vector field will move along one of the streamlines by itself. In other words the work done on the particle at it moves along the streamline by the vector field is [itex]\int_C{\overrightarrow{F}\cdot d\overrightarrow{r}}[/itex]. This makes sense.
So, now let's draw any closed curve (shown in pink) inside the domain where [itex]\overrightarrow{F}[/itex] is defined. According to the calculus, since [itex]\overrightarrow{F}[/itex] is conservative, it turns out that [itex]\oint{\overrightarrow{F}\cdot d\overrightarrow{r}}=0[/itex]. I really don't understand two things.
1. How do we get a particle to move along the closed curve we drew even when we know that by itself the particle wants to move along one of the streamlines. Let's say [itex]\overrightarrow{F}[/itex] is some electric field and the particle is a charged particle. How can we make it move along that closed curve. In most calculus books, they just say, "if the particle moves along the closed curve..." But how when it should move along a streamline?
2. Let's assume that somehow the particle decides to move along the closed curve ? How can the work done by the field be 0 ? What caused the particle to move then ?
Please help clarify.
I'm having problems understanding what exactly is meant by a conservative vector field. So, allow me to explain how I currently understand it.
Let [itex]\overrightarrow{F}[/itex] be some sort of a vector field that is conservative. The stream plot of [itex]\overrightarrow{F}[/itex] is shown below.
From my understanding, for a particle that interacts with field, the streamlines show the direction of flow of the particle. I have colored two streamlines in red to illustrate that. So, any particle placed at any location in the vector field will move along one of the streamlines by itself. In other words the work done on the particle at it moves along the streamline by the vector field is [itex]\int_C{\overrightarrow{F}\cdot d\overrightarrow{r}}[/itex]. This makes sense.
So, now let's draw any closed curve (shown in pink) inside the domain where [itex]\overrightarrow{F}[/itex] is defined. According to the calculus, since [itex]\overrightarrow{F}[/itex] is conservative, it turns out that [itex]\oint{\overrightarrow{F}\cdot d\overrightarrow{r}}=0[/itex]. I really don't understand two things.
1. How do we get a particle to move along the closed curve we drew even when we know that by itself the particle wants to move along one of the streamlines. Let's say [itex]\overrightarrow{F}[/itex] is some electric field and the particle is a charged particle. How can we make it move along that closed curve. In most calculus books, they just say, "if the particle moves along the closed curve..." But how when it should move along a streamline?
2. Let's assume that somehow the particle decides to move along the closed curve ? How can the work done by the field be 0 ? What caused the particle to move then ?
Please help clarify.