- #1
fab13
- 318
- 6
Hello,
I try to apprehend the notion of covariant derivative. In order to undertsand better, here is a figure on which we are searching for express the difference [itex] \vec{V} = \vec{V}(M') - \vec{V}(M)[/itex] :
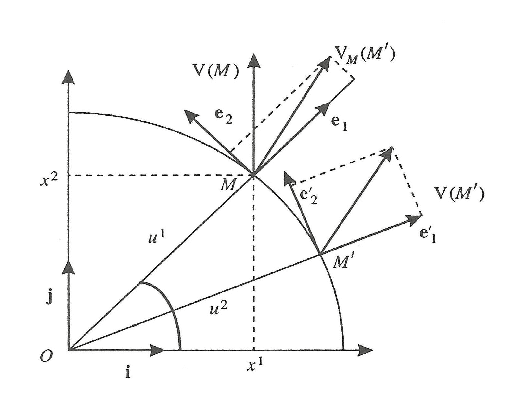
In order to evaluate this difference, we do a parallel transport of [itex]\vec{V}(M')[/itex] at point [itex]M[/itex].
When one writes what we call "the absolute differential" [itex]\text{D}\,v_{k}[/itex] :
[itex]\text{D}\,v_{k} = (\partial_{j}\,v_{k} - v_{i}\Gamma_{jk}^{i})\,dy^{j}[/itex]
does [itex]\text{D}\,v_{k}[/itex] represent the difference that we could get if the 2 vectors [itex]\vec{V}(M')[/itex] and [itex]\vec{V}(M)[/itex] were expressed in the Cartesian referential ?
So that we could write also from the above figure (with [itex]\theta=\theta(M)[/itex] :
[itex]\text{D}\,v_{x}=(\vec{V}_{\rho}(M')-\vec{V}_{\rho}(M))cos(\theta)[/itex]
and
[itex]\text{D}\,v_{y}=(\vec{V}_{\rho}(M')-\vec{V}_{\rho}(M))sin(\theta)[/itex]
??
Then, if this covariant derivative is zero, one can write :
[itex]\text{d}\,v_{k} = v_{j}\,\Gamma_{ik}^{j}\,dy^{i}[/itex]
does it significate curvilinear coordinates changes in the way that they are equal to [itex]v_{j}\,\Gamma_{ik}^{j}\,dy^{i}[/itex] ?
In the case of a parallel transport of vector [itex]\vec{V}(M')[/itex] from [itex]M'[/itex] point to [itex]M[/itex] point, why absolute differential between the
two vectors is equal to zero whereas the two vectors are not equal in the Cartesian coordinates system (O,x,y) ?
And if I take the same curvilinear coordinates for the two vectors, I get for the absolute differential :
[itex]\text{D}\,v_{k} = v_{j}\,\Gamma_{ik}^{j}\,dy^{i}[/itex]
What does this case represent on the above figure ?
If anyone could help me to clarify this notion of covariant derivative and parallel transport.
Thanks.
I try to apprehend the notion of covariant derivative. In order to undertsand better, here is a figure on which we are searching for express the difference [itex] \vec{V} = \vec{V}(M') - \vec{V}(M)[/itex] :
In order to evaluate this difference, we do a parallel transport of [itex]\vec{V}(M')[/itex] at point [itex]M[/itex].
When one writes what we call "the absolute differential" [itex]\text{D}\,v_{k}[/itex] :
[itex]\text{D}\,v_{k} = (\partial_{j}\,v_{k} - v_{i}\Gamma_{jk}^{i})\,dy^{j}[/itex]
does [itex]\text{D}\,v_{k}[/itex] represent the difference that we could get if the 2 vectors [itex]\vec{V}(M')[/itex] and [itex]\vec{V}(M)[/itex] were expressed in the Cartesian referential ?
So that we could write also from the above figure (with [itex]\theta=\theta(M)[/itex] :
[itex]\text{D}\,v_{x}=(\vec{V}_{\rho}(M')-\vec{V}_{\rho}(M))cos(\theta)[/itex]
and
[itex]\text{D}\,v_{y}=(\vec{V}_{\rho}(M')-\vec{V}_{\rho}(M))sin(\theta)[/itex]
??
Then, if this covariant derivative is zero, one can write :
[itex]\text{d}\,v_{k} = v_{j}\,\Gamma_{ik}^{j}\,dy^{i}[/itex]
does it significate curvilinear coordinates changes in the way that they are equal to [itex]v_{j}\,\Gamma_{ik}^{j}\,dy^{i}[/itex] ?
In the case of a parallel transport of vector [itex]\vec{V}(M')[/itex] from [itex]M'[/itex] point to [itex]M[/itex] point, why absolute differential between the
two vectors is equal to zero whereas the two vectors are not equal in the Cartesian coordinates system (O,x,y) ?
And if I take the same curvilinear coordinates for the two vectors, I get for the absolute differential :
[itex]\text{D}\,v_{k} = v_{j}\,\Gamma_{ik}^{j}\,dy^{i}[/itex]
What does this case represent on the above figure ?
If anyone could help me to clarify this notion of covariant derivative and parallel transport.
Thanks.