Understanding how to derive the stress-energy tensor formula

[JD_PM]

TL;DR Summary

Exercise statement: Prove that the stress-energy tensor is given by the functional derivative of the action with respect to $\delta g^{\mu \nu}$



$$T_{\mu \nu} = \frac{-2}{\sqrt{-g}} \frac{\delta S}{\delta g^{\mu \nu}}$$

Tong proposes the following exercise in this lecture (around 25:30, section b)):



Exercise statement: Prove that the stress-energy tensor is given by the functional derivative of the action with respect to $\delta g^{\mu \nu}$

$$T_{\mu \nu} = \frac{-2}{\sqrt{-g}} \frac{\delta S}{\delta g^{\mu \nu}}$$

I've been trying to solve it based on Tong's suggestion: using what he calls 'another cute trick' (I attached the PDF, owned by the University of Cambridge, UK).

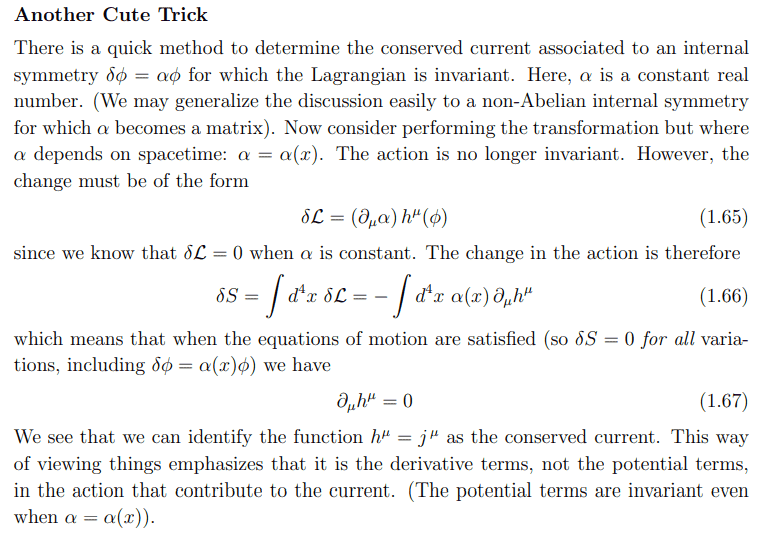
After being stuck in it for a while I found a method in Carroll's book, which is as follows:

He takes the action for the Klein-Gordon scalar field $\phi$ (EQ 4.52 in Carroll's book)

$$S_{\phi} = \int \Big( -\frac 1 2 g^{\mu \nu} (\nabla_{\mu} \phi)(\nabla_{\nu} \phi)-V(\phi) \Big) \sqrt{-g} d^n x$$

At this point, instead of varying the action with respect to $\phi$ we do so with respect to $g^{\mu \nu}$. We get (the following EQs are 4.77 and 4.78 in Carroll's book):

$$\delta S_{\phi}= \int d^n x\Big[ \sqrt{-g} \Big( - \frac 1 2 \delta g^{\mu \nu} \nabla_{\mu} \phi \nabla_{\nu} \phi \Big) + \delta \sqrt{-g} \Big( -\frac 1 2 g^{\mu \nu} \nabla_{\mu} \phi \nabla_{\nu} \phi -V(\phi) \Big) \Big]$$

At this point we do some relabelling and we apply $\delta \sqrt{-g}=-\frac 1 2 \sqrt{-g} g_{\mu \nu} \delta g^{\mu \nu}$ (please let me skip the proof of such a formula; it is explained in Carroll's book, page 163) to get

$$\delta S_{\phi}=\int d^n x \sqrt{-g} \delta g^{\mu \nu} \Big[ -\frac 1 2 \nabla_{\mu} \phi \nabla_{\nu} \phi + \Big(-\frac 1 2 g_{\mu \nu} \Big) \Big( -\frac 1 2 g^{\rho \sigma} \nabla_{\rho} \phi \nabla_{\sigma} \phi -V(\phi) \Big) \Big]$$

From which we obtain the desired formula.

I have some questions:

1) Is this way of deriving the stress-energy tensor formula analogous to applying the 'another cute trick'?

2) It looks to me that everything boils down to seeing that the trick is varying the action with respect to the inverse metric instead of doing so with respect to $\phi$. This doesn't look intuitive to me. Is there a reason one could come up with the idea or it is simply a matter of trying and trying till one gets it?

3) Is there any more illuminating method out there?

Any help is appreciated.

Thank you

 

[Orodruin]

I would say that is the definition of the stress-energy tensor.

If you are wondering why it appears in Einstein’s field equations: The EFEs are the result of varying the full action (Einstein-Hilbert part plus any matter part) with respect to the metric. As such, they are the equations of motion for the metric, not for the field $\phi$.

If you vary the action wrt the field $\phi$ you would get the EoMs for $\phi$, but this is not what the EFEs are.

Compare to electromagnetism where you have an action containing both the EM vacuum action, the free part of the charged matter contents, and the interaction terms. Varying with respect to the motion of the charges, you would obtain Lorentz’ force law, which has an expression containing the EM fields as the source. If you vary the action wrt the EM 4-potential, you get Maxwell’s equations with the source term depending on the charges.

The GR situation is completely analogous. The stress-energy tensor is the source term of the EFEs, which are analogous to Maxwell’s equations, and therefore result from variation wrt the metric.

 

[JD_PM]

Let me take your electromagnetism analogy.

Let's deal with an action describing a photon in a four-dimensional Minkowski spacetime. The dynamics of this photon depend on a vector field $A_{\mu}$, which is on the Minkowski spacetime. The Lagrangian of the action is as follows

$$\mathcal{L}= -\frac 1 4 F_{\mu \nu} F^{\mu \nu} + 4 \pi A_{\mu} J^{\mu}$$

Where $F_{\mu \nu}$ is the field strength (antisymmetric) tensor defined as $F_{\mu \nu} := \partial_{\mu} A_{\nu} - \partial_{\nu} A_{\mu}$ and $J^{\mu}$ is a background field (what is meant with background field is this: we keep in mind that we fix the values of $J^{\mu}$ at every point in our spacetime) that interacts with $A_{\mu}$.

Varying with respect to the motion of the charges, you would obtain Lorentz’ force law, which has an expression containing the EM fields as the source.

Mmm I am afraid I do not understand what you mean here. What do you mean when you say 'varying with respect to the motion of the charges'?

I know how to get two Maxwell's equations out of the Bianchi's identity

$$\partial_{\mu} F_{\nu \rho} + \partial_{\nu} F_{\rho \mu} + \partial_{\rho} F_{\mu \nu}=0$$

These are:

$$\vec \nabla \cdot \vec B=0 \ \ \ \ (1)$$

$$\vec \nabla \times \vec E + \partial_t \vec E = 0 \ \ \ \ (2)$$

I guess what you meant is that if we vary the action wrt a quantity (i.e. 'the motion of the charges') we will get $(1)$ and $(2)$. But what is this quantity mathematically speaking?

My apologies if my guess is wrong and this is not what you meant

If you vary the action wrt the EM 4-potential, you get Maxwell’s equations with the source term depending on the charges.

Let me see if I understand your comment.

If we decide to vary the action wrt the vector field $A_{\mu}$ we are going to get the other 2 Maxwell's equations:

$$\partial_{\mu} F^{\mu \nu} = -4 \pi J^{\nu}$$

Or in a more familiar way

$$\vec \nabla \cdot \vec E = 4 \pi \rho \ \ \ \ (3)$$

$$\vec \nabla \times \vec B - \partial_t \vec E = 4 \pi \vec J \ \ \ \ (4)$$

Is this what you meant?

Thank you.

 

[vanhees71]

Energy is the conserved quantity due to time-translation invariance and momentum is the conserved quantity due to space-translation invariance. In relativistic theories it's convenient to treat both symmetries of the Minkowski space at once, using space-time-translation, i.e., $x^{\mu} \mapsto x^{\prime \mu}=x^{\mu}-\delta a^{\mu}$ with $\delta a^{\mu}=\text{const}$.

In the case of the em. field there's some subtlety with gauge invariance. Since the energy-momentum tensor is only determined up to a total divergence you can choose it such to give a gauge invariant (symmetric) energy momentum tensor. An elegant way to achieve this is to take into account that the four-potential is only determined modulo a gauge transformation. Thus the general realization of translation invariance is
$$A_{\mu}'(x')=A_{\mu}(x)-\delta a^{\nu} \partial_{\mu} \chi_{\mu}(x).$$
Then you use this in Noether's theory and adjust $\chi_{\mu}$ such as to get a gauge-invariant symmetric energy-momentum tensor.

An alternative is to rely on GR and take your Lagrangian as the "matter part" of the Einstein-Hilbert Lagrangian. Varying with respect to the metric then leads to the symmetric energy-momentum tensor of the corresponding "matter fields" (or here the electromagnetic field).

 

[Orodruin]

Let me take your electromagnetism analogy.

Let's deal with an action describing a photon in a four-dimensional Minkowski spacetime. The dynamics of this photon depend on a vector field $A_{\mu}$, which is on the Minkowski spacetime. The Lagrangian of the action is as follows

$$\mathcal{L}= -\frac 1 4 F_{\mu \nu} F^{\mu \nu} + 4 \pi A_{\mu} J^{\mu}$$

Where $F_{\mu \nu}$ is the field strength (antisymmetric) tensor defined as $F_{\mu \nu} := \partial_{\mu} A_{\nu} - \partial_{\nu} A_{\mu}$ and $J^{\mu}$ is a background field (what is meant with background field is this: we keep in mind that we fix the values of $J^{\mu}$ at every point in our spacetime) that interacts with $A_{\mu}$.
Mmm I am afraid I do not understand what you mean here. What do you mean when you say 'varying with respect to the motion of the charges'?

I know how to get two Maxwell's equations out of the Bianchi's identity

$$\partial_{\mu} F_{\nu \rho} + \partial_{\nu} F_{\rho \mu} + \partial_{\rho} F_{\mu \nu}=0$$

These are:

$$\vec \nabla \cdot \vec B=0 \ \ \ \ (1)$$

$$\vec \nabla \times \vec E + \partial_t \vec E = 0 \ \ \ \ (2)$$

I guess what you meant is that if we vary the action wrt a quantity (i.e. 'the motion of the charges') we will get $(1)$ and $(2)$. But what is this quantity mathematically speaking?

My apologies if my guess is wrong and this is not what you meant

No. I mean that you have charges whose motion you vary. This can occur either through a charged field (then you vary with respect to that field) or through an assumed point charge (vary with respect to the world-line of the point charge). You should get the Lorentz force law out. Not Maxwell's equations.

The Bianchi identity follows directly from the definition of the field strength tensor in terms of the 4-potential.

So, consider a point charge moving along the world line $x^\mu(s)$. Express the 4-current in terms of this world line, and vary the full lagrangian with respect to the $x^\mu$. This gives you the Lorentz force law.

Let me see if I understand your comment.

If we decide to vary the action wrt the vector field $A_{\mu}$ we are going to get the other 2 Maxwell's equations:

$$\partial_{\mu} F^{\mu \nu} = -4 \pi J^{\nu}$$

Or in a more familiar way

$$\vec \nabla \cdot \vec E = 4 \pi \rho \ \ \ \ (3)$$

$$\vec \nabla \times \vec B - \partial_t \vec E = 4 \pi \vec J \ \ \ \ (4)$$

Is this what you meant?

Yes, varying with respect to the 4-potential gives you the part of Maxwell's equations that do not follow from the definition of the field strength tensor.

 

[JD_PM]

Hi vanhees71

In the case of the em. field there's some subtlety with gauge invariance. Since the energy-momentum tensor is only determined up to a total divergence you can choose it such to give a gauge invariant (symmetric) energy momentum tensor. An elegant way to achieve this is to take into account that the four-potential is only determined modulo a gauge transformation. Thus the general realization of translation invariance is
$$A_{\mu}'(x')=A_{\mu}(x)-\delta a^{\nu} \partial_{\mu} \chi_{\mu}(x).$$
Then you use this in Noether's theory and adjust $\chi_{\mu}$ such as to get a gauge-invariant symmetric energy-momentum tensor.

Mmm let me check if I understand your point.

Bianchi's identity and $\partial_{\nu} F^{\mu \nu} = s^{\mu}$ can be derived from the following Lagrangian density (in natural units):

$$\mathcal{L}=-\frac 1 4 F_{\mu \nu} F^{\mu \nu} - s_{\mu} A^{\mu}$$

Where $s_{\mu}$ is the charge-current density; $s_{\mu}=(\rho, \vec j)$

Then your point is that such a Lagrangian is invariant under Lorentz-Gauge transformations, which have the general form $A_{\mu}(x) \rightarrow A_{\mu'}(x) = A_{\mu}(x) + \partial_{\mu} f(x)$.

Applying the transformation to the Lagrangian we get

$$\mathcal{L} \rightarrow \mathcal{L} - s_{\mu}(x) \partial^{\mu} f(x)=\mathcal{L}-\partial^{\mu}\Big( s_{\mu}(x)f(x) \Big)$$

Then one could naively think that the Lagrangian is not invariant. However, adding derivatives of arbitrary functions of the vector field $A_{\mu}$ does not alter the field equations, which means that the Lagrangian is Lorentz-Gauge invariant.

Source: Quantum Field Theory by Mandl and Shaw, chapter 5

 

[JD_PM]

So, consider a point charge moving along the world line $x^\mu(s)$. Express the 4-current in terms of this world line, and vary the full lagrangian with respect to the $x^\mu$. This gives you the Lorentz force law.

Oh I think I see your point

Alright, let us have the Lagrangian I posted at #6

$$\mathcal{L}=-\frac 1 4 F_{\mu \nu} F^{\mu \nu} - s_{\mu} A^{\mu}=-\frac 1 4 F_{\mu \nu} F^{\mu \nu} - \rho A^{0} - \vec j (x^{i})A_i $$

Where $i=1,2,3$.

So do you mean that if we vary the action with Lagrangian $\mathcal{L}=-\frac 1 4 F_{\mu \nu} F^{\mu \nu}- \rho A^{0} - \vec j (x^{i})A_i$ wrt $\vec j (x^{i})$ we are going to get the Lorentz force law?

 

[Orodruin]

So do you mean that if we vary the action with Lagrangian $\mathcal{L}=-\frac 1 4 F_{\mu \nu} F^{\mu \nu}- \rho A^{0} - \vec j (x^{i})A_i$ wrt $\vec j (x^{i})$ we are going to get the Lorentz force law?

The Lagrangian is not as easy as that. You need a model for what charges are placed into the theory (for example, point charges). Adding the appropriate Lagrangian terms is then going to be a matter of constructing $\rho$ and $\vec j$ in terms of the world-line of the point charge (which will include delta distributions). Varying with respect to the world-line would then give you the Lorentz force law.

 

[JD_PM]

The Lagrangian is not as easy as that. You need a model for what charges are placed into the theory (for example, point charges). Adding the appropriate Lagrangian terms is then going to be a matter of constructing $\rho$ and $\vec j$ in terms of the world-line of the point charge (which will include delta distributions). Varying with respect to the world-line would then give you the Lorentz force law.

Oh I see. I was stuck in how to get such a Lagrangian and I did some googling. I found two candidates

1)

$$\mathcal{L_p}=[e^{2 a \phi} \eta_{\mu \nu} \dot x^{\mu} \dot x^{\nu} + \sigma e^{2b \phi} (\dot x^4 + A_{\mu} \dot x^{\mu})^2]^{\frac 1 2}$$

Which comes from the Kaluza-Klein theory.

Source: https://arxiv.org/pdf/1705.03362.pdf, equation 7

2)

$$\mathcal{L}=-m \int d\tau \delta(x-w(\tau) ) + q \int d\tau \frac{dw^\mu}{d\tau} A_\mu \delta(x - w(\tau))$$

Source: https://www.physicsforums.com/threads/lorentz-force-law-from-action.758709/

Now you suggest:

constructing $\rho$ and $\vec j$ in terms of the world-line of the point charge (which will include delta distributions).

But I do not really see how to do so in any of the above Lagrangians...

What Lagrangian you'd suggest I should work out? If none of these two meet what you had in mind, could you please give me an example of a Lagrangian meeting your standards?

Thank you

 

[JD_PM]

Besides, I am wondering if the way Carroll derived the stress energy tensor formula is what Tong had in mind when he proposed the exercise in the lecture I attached (please see original post; the exercise is proposed by Tong $\sim$ minute 25:30).