Understanding the Equipartition Theorem for Ideal Gases

[GravityX]

Homework Statement

At what temperature does the degrees of freedom freeze (estimate)

Relevant Equations

none

Hi,

I am unfortunately stuck with the following task

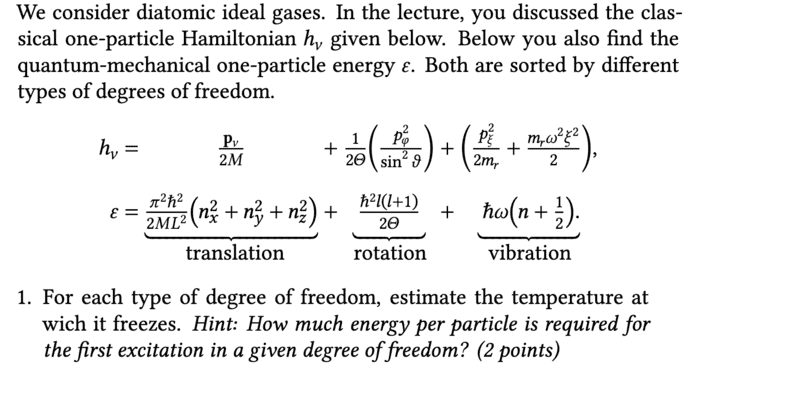
I started once with the hint that at very low temperatures the diatomic ideal gas behaves like monatomic gas and has only three degrees of freedom of translation $f=3$. If you then excite the gas by increasing the temperature, you add two degrees of freedom of rotation, $f=5$ and if you then excite the gas even further, you add two more degrees of freedom of vibration $f=7$.

The Equipartition theorem states that the internal energy is distributed equally among the degrees of freedom. The calculation of the internal energy for the ideal gas is $U=\frac{3}{2}RT$ for the translation, $U=\frac{5}{2}RT$ for the rotation and $U=\frac{7}{2}RT$ for the oscillation.

Unfortunately, I don't know either $U$ or $T$, but I can't think of any other way to estimate the temperature.

 

[vela]

Did you use the provided hint for each type of motion?

 

[GravityX]

Thanks vela for your help

I would now proceed as follows

Translation:$\frac{3}{2}RT=\frac{\pi^2 \hbar^2}{2ML^2}(n_x^2+n_y^2+n_z^2)$

Rotation: $\frac{5}{2}RT=\frac{\pi^2 \hbar^2}{2ML^2}(n_x^2+n_y^2+n_z^2)+\frac{\hbar^2l(l+1)}{2\theta}$

Oscillation: $\frac{7}{2}RT=\frac{\pi^2 \hbar^2}{2ML^2}(n_x^2+n_y^2+n_z^2)+\frac{\hbar^2l(l+1)}{2\theta}+\hbar\omega(n+\frac{1}{2})$

Now I can solve the individual equations according to the temperature with

For translation, $n_x^2,n_y^2,n_z^2=1$
For rotation $n_x^2,n_y^2,n_z^2=2$ and $l=1$
During oscillation $n_x^2,n_y^2,n_z^2=2$ , $l=1$ and $n=1$

 

[vela]

The Hamiltonian is for a single particle, so you want to use the Boltzmann constant, not the gas constant.

 

[GravityX]

Thanks vela for your help

So $\frac{3}{2}k_bT$ instead of $\frac{3}{2}RT$.