- #1
physics_CD
- 6
- 0
- Homework Statement
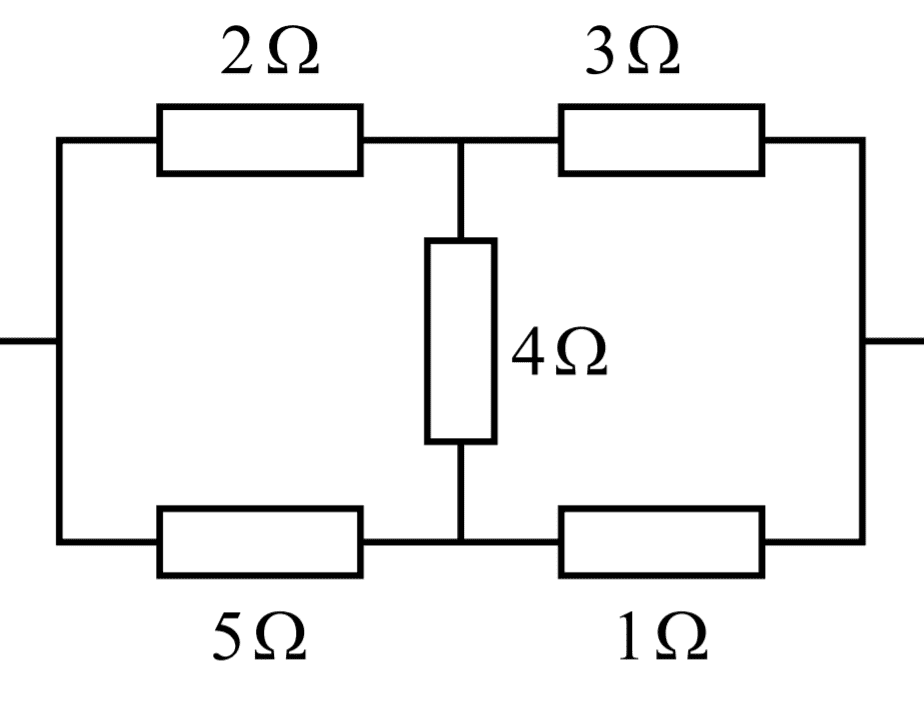
- I have this resistor which is connected to some battery (not very important). I don't understand why the total voltage is equal to the voltage drop over the 3 Ω and 2 Ω resistors. Why is it not dependent on the resistor in the middle with 4 Ω . I do understand it if the 4 Ω were substituted with a wire. But how can it be that there is no difference whether it is 4 Ω or 0 Ω?
- Relevant Equations
- .
I really don't have any clue why the total voltage is equal to the voltage drop over the 3 Ω and 2 Ω resistors and independent of the 4 Ω resistor . Does it have to do with parallel circuits?