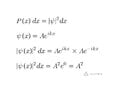jjson775
- 112
- 26
- TL;DR Summary
- Location of a free particle with known momentum
The textbook I am self studying says that the wave function for a free particle with a known momentum, on the x axis, can be given as Asin(kx) and that the particle has an equal probability of being at any point along the x axis. I understand the square of the wave function to be the probability of finding the particle at a particular location. The square of Asin (kx) is not a constant so how can the probability be the same for all values of x? What am I missing?