Unruh & Minkowski Modes: Analytic Extension Explained

[KDPhysics]

TL;DR Summary

Why do the so-called unruh modes which are extensions of Rindler modes share the same vacuum as the Minkowski modes, as explained in Carroll's Spacetime and geometry?

In Carroll "Spacetime and Geometry" I found the following explanation for why the analytically extended rindler modes share the same vacuum state as the Minkowski vacuum state:

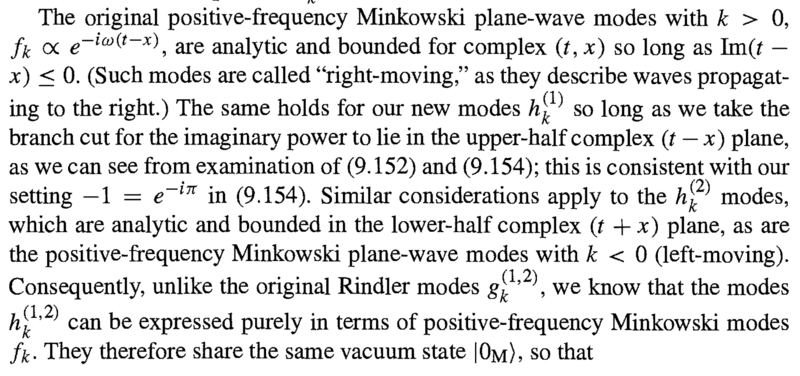
I can't quite understand why the fact that the extended modes [\tex]h_k^{(1),(2)}[\tex] are analytic and bounded on the same region as the Minkowski modes proves that [\tex]h_k^{(1),(2)}[\tex] can be expressed in terms of positive-frequency Minkowski modes only. Why are negative frequency modes out of the picture?

 

[Demystifier]

For simplicity, consider only right-moving modes. (The argument for left-moving ones will be analogous.) Positive frequency Minkowski modes are analytic in one half of the complex plane, while negative frequency Minkowski modes are analytic in the other half. Hence, a function that is analytic in one (and not in the other!) half of the plane must have expansion only in terms of one set of Minkowski modes. Modes which are not analytic in the needed half cannot contribute to a function which is analytic there.

 

[KDPhysics]

But couldn't the left moving negative frequency modes be analytic in that half of the complex plane?

 

[Demystifier]

But couldn't the left moving negative frequency modes be analytic in that half of the complex plane?

Right moving wave has an expansion only in terms of right moving modes. You cannot superimpose both left and right moving modes to get a purely right moving wave.

 

[KDPhysics]

I see, thanks!

 

[KDPhysics]

One last question, the Unruh modes as defined in Sean Carroll's "Spacetime and Geometry" are:
$$h_k^{(1)} = \frac{1}{\sqrt{2\sinh(\pi \omega/a)}}\big(e^{\pi \omega/2a} g_k^{(1)} + e^{-\pi \omega/2a} g_{-k}^{(2)}{}^*\big)$$
On the other hand this paper gives a different definition:
$$h_k^{(1)} = \frac{1}{\sqrt{2\sinh(\pi \omega/a)}}\big(e^{\pi \omega/2a} g_k^{(1)} + e^{-\pi \omega/2a} g_{k}^{(2)}{}^*\big)$$
I can't quite understand how these could be the same.

 

[Demystifier]

The other paper either has a typo or uses a different convention for definition of second modes.