chwala
Gold Member
- 2,827
- 415
- Homework Statement
- See attached
- Relevant Equations
- complex numbers
Is there any particular reason as to why certain texts use ##j## and others ##i## when looking at complex numbers? Maths is a relatively easy subject but at times made difficult with all this mix-up... i tend to use a lot of my time in trying to understand author's language and this is also evident on the convention used on argument, an area that is pretty easy/straightforward to me...this is akin to the mix up/confusion on the standard way of expressing derivatives noting that the two great mathematicians :Sir Isaac Newton and Leibnitz had different notations...
anyway, which is the standard way of expressing complex numbers?
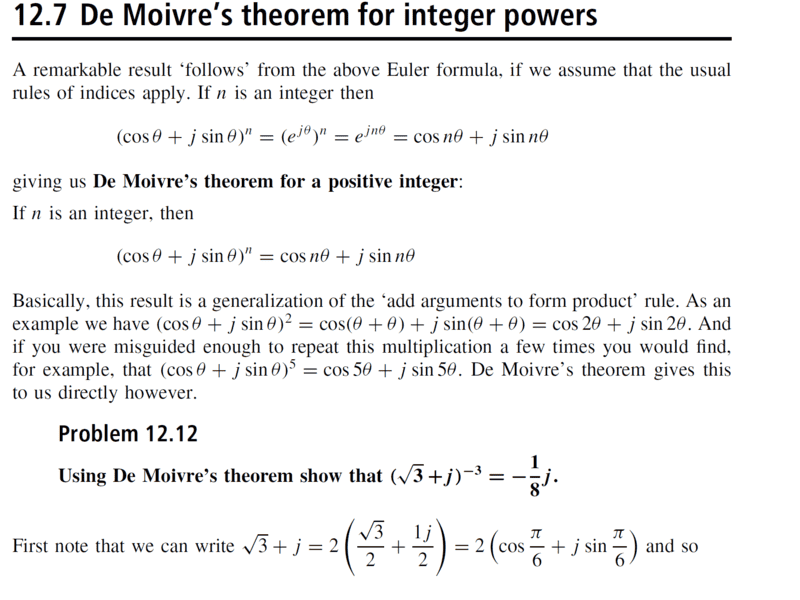
anyway, which is the standard way of expressing complex numbers?
Last edited: