Use the Shifting Theorem to find the Laplace transform

[member 731016]

Homework Statement

Please see below

Relevant Equations

Shifting theorem

For (b),

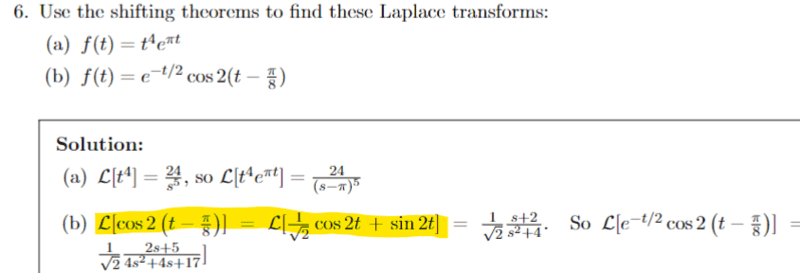
I'm confused on the highlighted step. Does someone please explain to me how they got from the left to the right?

Thanks!

 

[Frabjous]

cos(a-b)=cos(a)cos(b)+sin(a)sin(b) and cos(π/4)=sin(π/4)=1/√2

 

[Orodruin]

Note again the typos in the solution. For example the $1/\sqrt 2$ multiplying only one of the terms. The amount of typos in your problems is really beyond critisism. It is outright embarrassing to whatever institution is giving these out.

 

[WWGD]

Note again the typos in the solution. For example the $1/\sqrt 2$ multiplying only one of the terms. The amount of typos in your problems is really beyond critisism. It is outright embarrassing to whatever institution is giving these out.

Criticism.

 

[Orodruin]

Criticism.

Valid critisism. I have no idea where OP is studying, but I know that in every single problem they have posted the provided solution has been full of typos. Many times those typos have been the source of OP’s confusion.

 

[Orodruin]

To put the criticism into context. There are typos in all of the solutions provided by the teachers in the following recent threads:
https://www.physicsforums.com/threa...heorem-to-find-the-laplace-transform.1062007/
https://www.physicsforums.com/threads/laplace-transform-of-proper-rational-function.1061978/
https://www.physicsforums.com/threads/ode-with-constant-coefficient-b.1061726
These all seem to originate from the same course, a course on ODEs.