sHatDowN
- 52
- 7
- Homework Statement
- Force image and resolve force
- Relevant Equations
- vector
The components of the force of 800 lb F along the non-perpendicular axes Get a, b. Also determine the image F on the axes a, b
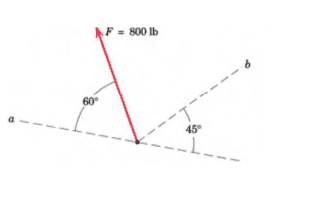
could some one help me how to resolve forces non-perpendicular.And also what is the meaning of image F one the a,b axes
could some one help me how to resolve forces non-perpendicular.And also what is the meaning of image F one the a,b axes