Verifying V Graph and Acceleration Calculations

[Peter G.]

For the V Graph upload: It asks to: The graph shows the motion of a stone thrown vertically upwards. Calculate the maximum height reached by the stone: a) by first finding the average velocity of the stone b) by finding the area under the graph

for a) I did total displacement / total time = ((12 x 12) / 2 ) / 12 = 6 m/s

for b) I did ((12 x 12) / 2 ) = 72 m

Are those right? Because it doesn't make much sense that the ball got up to a height of 72 m

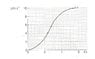
For the Second upload, part A of the question asks us for the greatest acceleration in the graph. I chose the greatest gradient, which I assume is that straight line in the center and calculated 4 m/s ^2. The graph is not very good so you guys probably can't help me out much on that one I think.

Part c of the question asks us to sketch the general shape of the velocity time graph as a acceleration time graph. I never did one with curves so, any thoughts? Did I go completely wrong and I should have another go?

Thanks,
Peter.

 

[JaredJames]

A stone thrown upwards will travel vertically, stop, descend. Your velocity time graph doesn't reflect this motion - I can't see the others because they are too small or not labelled.

Now your velocity time graph only covers the upward part of the journey.

Based on your velocity time graph, you get the deceleration from total velocity / total time.

So, deceleration = (12 / 12) = 1m/s².

So from that, you can see that the speed will only reduce by 1m/s² each second, so it isn't so hard to believe it could go 72m up - after the first 3 seconds it will have gone 12m + 11m + 10m = 33m - so you can see how it adds up.

The problem is, a stone thrown vertically upwards should have a deceleration of g, but we'll overlook that for now.

Just to check your answers:

Using the equations of motion, you know a = 1, v = 0, u = 12 and t = 12. Using these you can get distance (s).
v² = u² + 2as
( v² - u² ) / 2a = s = (0² - 12²) / 2(1) = 72m.

So your value for distance traveled is ok.

72 / 12 = 6m/s

So your value for average velocity is ok.

I can't answer more than that as I can't see the other graphs. For the straight line velocity time graph, the acceleration is constant. The second graph (s-curve) appears to be the downwards part but I can't see it as it's too small. But yes, the steepest part of that graph would be the greatest acceleration.

Your last graph looks wrong.

For the upwards section of the journey, the deceleration is constant so should be a straight horizontal line (acceleration on the y-axis, time on the x-axis). For the downwards section it should be curved.

 

[Peter G.]

Ok, firstly, thanks for the help with the first question.

(I uploaded better versions of the previous graphs)

I've only dealt with very simple acceleration time graphs and their conversions, I guess that's why they ask for the general shape of this one. So, if you can, help me work through it.

So, for part (a) in which I should find the maximum acceleration I used two red dots to show where I thought the steepest gradient was. It was a straight line in that part so I didn't use any tangent. I therefore got: Difference in y / difference in x = (5.6-4) / (2.8-2.4) = 1.6 /0.4 = 4m/s ^2

Now for the second part where I have to draw the graph:
For the first part of the graph, from 0 seconds to 2.2, the velocity is increasing at a non linear rate. So he is accelerating but not in a constant rate. So I should draw a curve for that part of the graph or a straight upward line as I did? For what I recognized as the second part of the graph, which I drew a line with a steeper gradient in the uploaded image I think I made a mistake since I stated a few times already that the line is straight there. I think I have to change that line for a horizontal line, since velocity is increasing constantly. For the third part of the graph, from 3 seconds to 5.6 seconds the velocity is still increasing, but like in the beginning not in a linear rate, so I don't know if I should draw a straight line upward, or a curve. And finally, for the last 0.4 seconds of the trip since the velocity straightens out, there is no acceleration, so I drew a straight line in the x axis.

Thanks again,
Peter