Virtual work of constraint forces in Hamilton’s principle

[Kashmir]

Goldstein 2ed pg 36

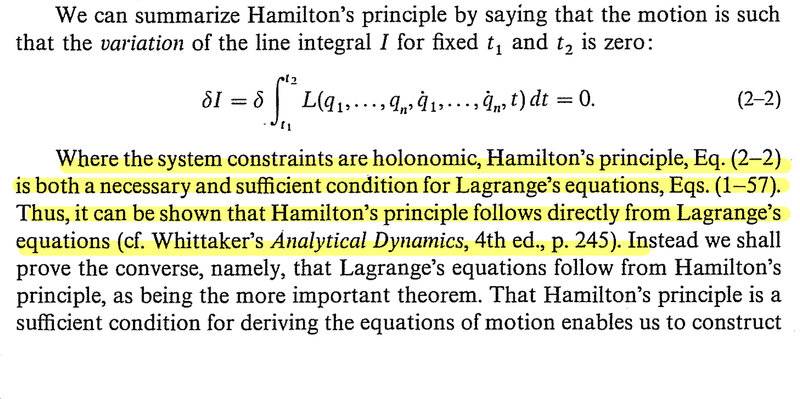
So in the case of holonomic constraints we can move back and forth between Hamiltons principle and Lagrange equations given as $\frac{d}{d t}\left(\frac{\partial L}{\partial \dot{q}_{j}}\right)-\frac{\partial L}{\partial q_{j}}=0$

But the Lagrange equations were derived from DAlembets principal Assuming that the virtual work of constraints is zero. But in the Hamilton‘s principal, we don’t see that the constraints should be workless .

Is it implicitly assumed that the virtual work of constraints is zero in Hamilton‘s principal?
How can we prove it?

 

[vanhees71]

Here the Hamilton principle is treated by working in the holonomous constraints by choosing a set of independent generalized coordinates. This leads to the EL equations of the 2nd kind. D'Alembert's principle is usually formulated in Cartesian coordinates with the constraints worked in using Lagrange multipliers, which leads to the Euler-Lagrange equations of the 1st kind.

Of course you can also use the Hamilton principle with Lagrange multipliers. Then it's entirely equivalent to the d'Alembert principle, leading to the same Euler-Lagrange equations of the 1st kind.

 

[Kashmir]

Here the Hamilton principle is treated by working in the holonomous constraints by choosing a set of independent generalized coordinates. This leads to the EL equations

To arrive at those El equations via DAlembert we begin by assuming workless constraints.

So it makes sense if that assumption is built in the Hamilton principle.

Is that so?

 

[vanhees71]

The Hamilton principle of least action says (for the special case of point-particle mechanics) that the equations of motion can be derived by assuming that the action functional is stationary for the trajectory of the particles,
$$S[q]=\int_{t_1}^{t_2} \mathrm{d} t L(q,\dot{q},t),$$
moving through fixed points at the times $t_1$ and $t_2$. The $q$ are arbitrary coordinates of the configuration space, where the particles are allowed to move.

To find the equations of motion consider the variation of the trajectory around the stationary one,
$$\delta S = \int_{t_1}^{t_2} \mathrm{d} t (\delta q \partial_q L + \delta \dot{q} \partial_{\dot q} L)=0.$$
Since the time is not varied, you have $\delta \dot{q} = \mathrm{d}_t \delta q$. Then you can integrate the 2nd term by parts, using that the initial and final points of the trajectory are fixed, i.e., $\delta q(t_1)=\delta q(t_2)=0$. This implies
$$\delta S=\int_{t_1}^{t_2} \mathrm{d} t \delta q [\partial_q L -\mathrm{d}_t (\partial_{\dot{q}}L) ]=0.$$
This can only hold true when the expression under the bracket vanishes, and this leads to the Euler-Lagrange equations,
$$\mathrm{d}_t (\partial_{\dot{q}} L) = \partial_q L.$$

 

[Kashmir]

The Hamilton principle of least action says (for the special case of point-particle mechanics) that the equations of motion can be derived by assuming that the action functional is stationary for the trajectory of the particles,
$$S[q]=\int_{t_1}^{t_2} \mathrm{d} t L(q,\dot{q},t),$$
moving through fixed points at the times $t_1$ and $t_2$. The $q$ are arbitrary coordinates of the configuration space, where the particles are allowed to move.

To find the equations of motion consider the variation of the trajectory around the stationary one,
$$\delta S = \int_{t_1}^{t_2} \mathrm{d} t (\delta q \partial_q L + \delta \dot{q} \partial_{\dot q} L)=0.$$
Since the time is not varied, you have $\delta \dot{q} = \mathrm{d}_t \delta q$. Then you can integrate the 2nd term by parts, using that the initial and final points of the trajectory are fixed, i.e., $\delta q(t_1)=\delta q(t_2)=0$. This implies
$$\delta S=\int_{t_1}^{t_2} \mathrm{d} t \delta q [\partial_q L -\mathrm{d}_t (\partial_{\dot{q}}L) ]=0.$$
This can only hold true when the expression under the bracket vanishes, and this leads to the Euler-Lagrange equations,
$$\mathrm{d}_t (\partial_{\dot{q}} L) = \partial_q L.$$

Thanks but I think that doesn't answer the question.

I know what Hamilton's principle is and how we can deduce EL equations via it using stationary integral of Lagrangian.

 

[vanhees71]

Then I don't understand the question.

 

[Kashmir]

Then I don't understand the question.

1) we can get EL equations via DAlemberts principle for the case of conservative systems.
However a crucial step while deriving those equations is that we assume that the virtual work of constraints is zero. Such constraints are also known as workless constraints.

2) Hamilton's principle is equivalent to DAlemberts principle for holonomic case. Obviously we get the same EL equations as with DAlemberts principle. Question:
Why doesn't the assumption of workless constraints enter when we use Hamilton's principle for holonomic constraints?

 

[vanhees71]

It's simply because it's another principle. They are equivalent in the sense that they lead to the same equations of motion. There are many more such principles. Hamilton's principle itself already comes in two variations: the one we discussed here in terms of the Lagrangian, where the trajectories are in configuration space, as well as the Hamiltonian version, where the trajectories are in phase space.

These two principles are by far the most important ones, because they turned out to be most easily usable in a wider range of applications. Within classical physics you can also use them to describe classical field theories.

Their main use is the application of the theory of Lie groups to derive conservation laws (Noether's theorems) or in the other direction to derive, how equations of motion must look like for given symmetries. In classical physics these are the symmetries of the underlying spacetime models.

Last but not least it's an important heuristical tool to also derive quantum theories, e.g., through canonical quantization, which assumes that the Poisson brackets of classical Hamiltonian dynamics translate into commutation relations for operators.

The classical Hamilton principle itself can be understood as derived from quantum theory as the classical approximation, making use of Feynman's path-integral formulation.