Viscosity & Temperature - Linearisation and glycerine?

[adityax26]

Hey all, I'm new to the forums and had a quick question:
(please tell me if this is the wrong topic under which I posted, please move it if needed! @mods)

I am doing a lab report investigating the effect of temperature (˚C) on the dynamic viscosity (Pa s) of glycerin. I am timing the time it takes for a small sphere (marble) to travel from point A to point B with terminal velocity and plugging this into the Stoke's Law Equation solving for viscosity. I am testing: 20 ˚C, 30 ˚C, 40 ˚C, 50 ˚C, 60 ˚C, 70 ˚C, and 80 ˚C. I had a few questions I'd like to ask if you guys!

1) How do I know that the marble when dropped will reach terminal velocity when dropping from a certain point to another? I am writing my controlled variables, and this is one of them. Is there an equation I can use with drag or something to find the time/distance required to reach this value? Essentially I tried the experiment with sunflower oil & water, but those were WAY too less viscous and it dropped within a few milliseconds; hard to measure, not sure if it even dropped at terminal velocity...

2) Is glycerin (or glycerol, same thing) a good liquid to use for such experiment? I am thinking of using either 1L or 2L of glycerin. I can let the marble drop for a maximum of 10-15 cm, and by then it MUST HAVE reached terminal velocity; do you think it will do so? Do you reccomend using 2L glycerin or will 1 L do? Will I also have enough time to measure within the two points of measurements (I will measure the time it takes to travel like 20 cm at terminal velocity)? The diameter of my tubes are like 6-7 cm and my marble diameter is like 1.5 cm (with mass of around 15 g)

3) When looking at some existing data, I noticed that temp. and viscosity are are inversely proportional. I took some data from the Internet, but how do I linearise this? In theory I thought that graphing a 1/T graph vs. viscosity would achieve this, but I don't really get a linear graph, do I? My two graphs:

https://imgur.com/uPWAhmG

https://imgur.com/eRBAgpE

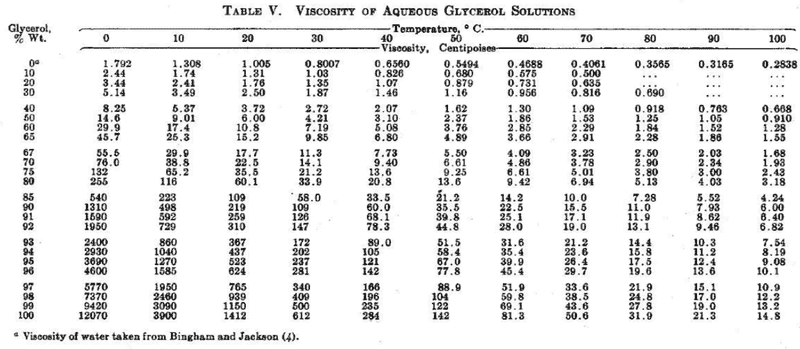
Glycerin & its viscosity at different temps/concs (I am using 100% vegetable glycerine):

Thanks so much! :D

 

[Chestermiller]

I'll be back with additional answers later. But, glycerine and glycerol are the same substance.

Try plotting the viscosity on a log scale as a function of 1/T (using absolute temperature) on an arithmetic scale. It should be close to a straight line.

 

[Chestermiller]

For question 2, what do you think you can do to answer this experimentally?

 

[adityax26]

For question 2, what do you think you can do to answer this experimentally?

I thought about actually conducting the experiment myself at home or quickly at school just to see if it does reach terminal velocity using a slowmotion video capture device and seeing how long it takes to travel 0.2 cm (for instance) by splitting the total distance (20 cm) in 10 parts and seeing if it is the same throughout.

The problem is is that I can only do this on Wednesday, which is the only time I will be able to conduct the experiment. I searched around for any formulas I could use but I am getting all advanced calculus equations that we haven't even been introduced to, so kinda confused here... Any tips? o:

 

[adityax26]

Oh yeah and also, it's linear if I plot 1/T versus log viscosity! But I was wondering, why is this? Since temp and visc. are inversely proportional, wouldn't 1/T vs plain viscosity linearise it? Are there any justifications I can make of why log viscosity vs. 1/T gives me a linear graph? Also, it's downwards sloping linear... could you please explain to me why this is, or what this shows about the relationships between the two variables

 

[Chestermiller]

Oh yeah and also, it's linear if I plot 1/T versus log viscosity! But I was wondering, why is this? Since temp and visc. are inversely proportional, wouldn't 1/T vs plain viscosity linearise it? Are there any justifications I can make of why log viscosity vs. 1/T gives me a linear graph? Also, it's downwards sloping linear... could you please explain to me why this is, or what this shows about the relationships between the two variables

Well, the viscosity decreases with increasing temperature, and, from the theory, what we are dealing with here is a kind of "activation energy" effect, which implies that the log of the viscosity is going to be nearly linear with the 1/T. This is the simplest terms I can explain it to someone at your present level.

 

[Chestermiller]

I thought about actually conducting the experiment myself at home or quickly at school just to see if it does reach terminal velocity using a slowmotion video capture device and seeing how long it takes to travel 0.2 cm (for instance) by splitting the total distance (20 cm) in 10 parts and seeing if it is the same throughout.

The problem is is that I can only do this on Wednesday, which is the only time I will be able to conduct the experiment. I searched around for any formulas I could use but I am getting all advanced calculus equations that we haven't even been introduced to, so kinda confused here... Any tips? o:

Well, you have the right idea. If you had more extensive background, we could set up a force balance on the marble, and predict theoretically its velocity with respect to time, and the time constant for its velocity to approach terminal velocity. But, as you said, you would have to know how to solve differential equations to do this. In lieu of this, the best you can do is experimentally measure the distance vs time over known distances (say increments of 10 cm), and see how close the velocity comes to being constant.

 

[adityax26]

Well, you have the right idea. If you had more extensive background, we could set up a force balance on the marble, and predict theoretically its velocity with respect to time, and the time constant for its velocity to approach terminal velocity. But, as you said, you would have to know how to solve differential equations to do this. In lieu of this, the best you can do is experimentally measure the distance vs time over known distances (say increments of 10 cm), and see how close the velocity comes to being constant.

Thanks! Do you think glycerin is a good viscous liquid to test this with?

 

[Chestermiller]

Thanks! Do you think glycerin is a good viscous liquid to test this with?

It depends on how much the marble weights. Can you use the viscous drag equation for a sphere to calculate the terminal velocity? If you don't know how to do this, Google "Falling Ball Viscometry."

 

[adityax26]

It depends on how much the marble weights. Can you use the viscous drag equation for a sphere to calculate the terminal velocity? If you don't know how to do this, Google "Falling Ball Viscometry."

Thanks again! Btw, one more question. If I plot 1/T vs. log viscosity I get a linear graph. Doing some research I found that they are related in a somewhat Arrhenius type relation, where the slope when graphed = Ea / R. However, what does this 'Ea' mean? In chemistry we learned that it was the energy required for particles to react, but I am confused as to what it means in terms of viscosity/liquid flow. I did some research but can't seem to find much... Would appreciate any tips!

 

[Chestermiller]

Thanks again! Btw, one more question. If I plot 1/T vs. log viscosity I get a linear graph. Doing some research I found that they are related in a somewhat Arrhenius type relation, where the slope when graphed = Ea / R. However, what does this 'Ea' mean? In chemistry we learned that it was the energy required for particles to react, but I am confused as to what it means in terms of viscosity/liquid flow. I did some research but can't seem to find much... Would appreciate any tips!

See chapter 1 of Transport Phenomena by Bird, Stewart, and Lightfoot.