- #1
matt2971
- 2
- 0
Hi, I'm unsure if I'm posting this in the correct place, so apologies in advance if not. I looked at the study board, but the post template doesn't apply to my question.
I'm a layperson who had previously only read popular science books on QM, but has recently been watching some more in-depth lectures on YouTube, in order to get a better idea of the mathematical concepts that model the theory. Specifically I've attempted to get most of my information from "Lecture 1 | Quantum Entanglements, Part 1 (Stanford)" and the follow-up lectures by Leonard Susskind.
On the back of this, I've been attempting to explain some of those concepts in writing (for my blog - nothing professional), concentrating on explaining imaginary and complex numbers, and why the maths and geometry of using the complex plane models the observed phenomena.
I'm only drafting, but am desperately needing a sanity check, to find out whether any of the conclusions I'm drawing are complete misunderstanding on my part, or whether I'm on the right track with some of them, but need amendments to my wording etc..
So, if anyone has time to take a look below and give me any feedback, I'd be very grateful.
One last thing - if I am completely wrong on all or any of these conclusions, please don't laugh too hard! I have almost no math skills, so I'm trying to just understand the concepts at a high level, and then use that to make visualizations. The main reason I'm writing it all down is for my own reference!
I realize that this endeavor may not actually be possible, but need to find out... think of it as an experiment ;)
NOTE: The sections in red are those I am most concerned about. Also, I've skipped the first part where I introduce "i".
----------------------------
The imaginary number i and multiples thereof, are used in a series (i, 2i, 3i etc) to create an extra axis on a graph, in addition to the regular x y and z axes representing the usual three spatial dimensions. In the visualization below, the three usual dimensions are combined into a single real axis:
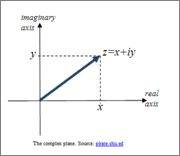
Points plotted against the real and imaginary axes then take the form of one real number (e.g. 2 or -2) and one imaginary number (e.g. 3i or -3i), making a composite that is known as a complex number (e.g. 2+3i or 2-3i or -2+3i or -2-3i).
This full graph on which both real and complex numbers can be plotted is known as the complex plane, and in quantum mechanics, it's not points that are plotted, but quantum state vectors, each representing a potential value for an observable of the system (for example the quantum spin). The quantum state vector in the illustration above is simply the arrow.
Now imagine a new vector that is the mirror image reflection of original vector across the real axis as shown below with the blue examples.
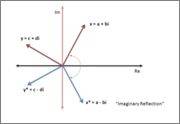
In the maths, these mirrored vectors are the complex conjugates of the original complex numbers, and are are created by reversing the plus or minus symbol of the imaginary part of the complex number. So for example, 2+3i has a complex conjugate of 2-3i. This is symbolized on a variable by adding a star symbol, so if a=2+3i then it's complex conjugate is know as a* and is equal to 2-3i.
Above you can see the original quantum state vectors y and x as the red vectors, and their complex conjugates y* and x* in blue. When the complex conjugate of a complex number is drawn, there is always an angle between them. This can also be drawn as triangle - just imagine a line between x and x*. The interior of this triangle can be thought of as a complex vector space, with vectors therein representing probability values. These probabilities indicate the likelihood of finding the system in a certain state when measured, and corresponds to one of the main features quantum mechanics, namely superposition.
Quantum state vectors can be manipulated using standard mathematical linear operators such as addition and multiplication. The mathematics of complex numbers is such that the results of these operations can be either another complex number, or a real one. One such operation is visualized as a rotation.
Note that if the state vector is entirely on the real axis, then in the maths it's complex conjugate is itself. In the visualization, this means that it has no mirror image, no rotation is necessary to make it real, and it's vector space of probabilities has no size. Correspondingly, in quantum mechanics, the observable of the system is not in superposition, but instead is a measured real quantity.
The specific operator that will rotate a vector from being somewhere in the complex plane to sitting squarely on the real axis as a normal real number is called the Hermitian operator. The application of the Hermitian operator therefore corresponds to the wave function collapse that takes place on measuring an aspect of a system.
Now consider that each quantum state vector (e.g. y and x above) represents just a single aspect of the system's state, and that for each vector there is a unique rotation (the Hermitian operator) that will bring it onto the real axis (this is where eigenvalues and eigenvectors come in, but I won't go into those concepts here).
So for example, if you rotate the entire system to bring x onto the real axis, then y will have rotated as well and will not be real. If you then rotate the system again so that y is real, then x returns to no longer being real. This feature corresponds to the uncertainty principle, where if you measure one observable of a quantum system, then the complimentary observable will be uncertain, or in other words, an unreal complex number.
Similarly, it's the use of i and the complex plane that allows quantum mechanics to model other features quantum mechanics like entanglement. Specifically, on systems containing two or more particles, certain mathematical operations do not commute. In maths with real numbers, that would be the equivalent of saying that 2 x 3 does not equal 3 x 2, but that they yield two different answers. This equates to an an extra state being available over and above those you might expect, and that state corresponds to the entangled systems.
The upshot of all this is that each unintuitive feature of quantum mechanics is modeled by the maths, and therefore if one can see what the maths is doing, then perhaps one can begin to get a better picture of what might be happening physically.
However, there is danger here. This is where science meets philosophy, and interpretation is everything.
Not always in physics do the apparent properties of the maths correspond to how things are physically. The use of complex numbers in classical wave mechanics described above is a good example, but there, at least as I understand it, the use of the extra dimension introduced by i is more like a shortcut to avoid doing harder, more regular maths. In quantum mechanics I don't believe that's the case. The i is a mandatory part of the theory.
Alternatively, sometimes different mathematical models are devised that yield the same results because they are equivalent. In quantum mechanics this already happened, where Matrix Mechanics was used before complex numbers to do exactly the same thing. In this case, what little can be drawn out of looking at the maths in question looks very similar to the complex plane model anyway, but there are other examples in physics where that's not the case. Godel's novel solution to Einstein's field equation is a good example.
I'm a layperson who had previously only read popular science books on QM, but has recently been watching some more in-depth lectures on YouTube, in order to get a better idea of the mathematical concepts that model the theory. Specifically I've attempted to get most of my information from "Lecture 1 | Quantum Entanglements, Part 1 (Stanford)" and the follow-up lectures by Leonard Susskind.
On the back of this, I've been attempting to explain some of those concepts in writing (for my blog - nothing professional), concentrating on explaining imaginary and complex numbers, and why the maths and geometry of using the complex plane models the observed phenomena.
I'm only drafting, but am desperately needing a sanity check, to find out whether any of the conclusions I'm drawing are complete misunderstanding on my part, or whether I'm on the right track with some of them, but need amendments to my wording etc..
So, if anyone has time to take a look below and give me any feedback, I'd be very grateful.
One last thing - if I am completely wrong on all or any of these conclusions, please don't laugh too hard! I have almost no math skills, so I'm trying to just understand the concepts at a high level, and then use that to make visualizations. The main reason I'm writing it all down is for my own reference!
I realize that this endeavor may not actually be possible, but need to find out... think of it as an experiment ;)
NOTE: The sections in red are those I am most concerned about. Also, I've skipped the first part where I introduce "i".
----------------------------
The imaginary number i and multiples thereof, are used in a series (i, 2i, 3i etc) to create an extra axis on a graph, in addition to the regular x y and z axes representing the usual three spatial dimensions. In the visualization below, the three usual dimensions are combined into a single real axis:
Points plotted against the real and imaginary axes then take the form of one real number (e.g. 2 or -2) and one imaginary number (e.g. 3i or -3i), making a composite that is known as a complex number (e.g. 2+3i or 2-3i or -2+3i or -2-3i).
This full graph on which both real and complex numbers can be plotted is known as the complex plane, and in quantum mechanics, it's not points that are plotted, but quantum state vectors, each representing a potential value for an observable of the system (for example the quantum spin). The quantum state vector in the illustration above is simply the arrow.
Now imagine a new vector that is the mirror image reflection of original vector across the real axis as shown below with the blue examples.
In the maths, these mirrored vectors are the complex conjugates of the original complex numbers, and are are created by reversing the plus or minus symbol of the imaginary part of the complex number. So for example, 2+3i has a complex conjugate of 2-3i. This is symbolized on a variable by adding a star symbol, so if a=2+3i then it's complex conjugate is know as a* and is equal to 2-3i.
Above you can see the original quantum state vectors y and x as the red vectors, and their complex conjugates y* and x* in blue. When the complex conjugate of a complex number is drawn, there is always an angle between them. This can also be drawn as triangle - just imagine a line between x and x*. The interior of this triangle can be thought of as a complex vector space, with vectors therein representing probability values. These probabilities indicate the likelihood of finding the system in a certain state when measured, and corresponds to one of the main features quantum mechanics, namely superposition.
Quantum state vectors can be manipulated using standard mathematical linear operators such as addition and multiplication. The mathematics of complex numbers is such that the results of these operations can be either another complex number, or a real one. One such operation is visualized as a rotation.
Note that if the state vector is entirely on the real axis, then in the maths it's complex conjugate is itself. In the visualization, this means that it has no mirror image, no rotation is necessary to make it real, and it's vector space of probabilities has no size. Correspondingly, in quantum mechanics, the observable of the system is not in superposition, but instead is a measured real quantity.
The specific operator that will rotate a vector from being somewhere in the complex plane to sitting squarely on the real axis as a normal real number is called the Hermitian operator. The application of the Hermitian operator therefore corresponds to the wave function collapse that takes place on measuring an aspect of a system.
Now consider that each quantum state vector (e.g. y and x above) represents just a single aspect of the system's state, and that for each vector there is a unique rotation (the Hermitian operator) that will bring it onto the real axis (this is where eigenvalues and eigenvectors come in, but I won't go into those concepts here).
So for example, if you rotate the entire system to bring x onto the real axis, then y will have rotated as well and will not be real. If you then rotate the system again so that y is real, then x returns to no longer being real. This feature corresponds to the uncertainty principle, where if you measure one observable of a quantum system, then the complimentary observable will be uncertain, or in other words, an unreal complex number.
Similarly, it's the use of i and the complex plane that allows quantum mechanics to model other features quantum mechanics like entanglement. Specifically, on systems containing two or more particles, certain mathematical operations do not commute. In maths with real numbers, that would be the equivalent of saying that 2 x 3 does not equal 3 x 2, but that they yield two different answers. This equates to an an extra state being available over and above those you might expect, and that state corresponds to the entangled systems.
The upshot of all this is that each unintuitive feature of quantum mechanics is modeled by the maths, and therefore if one can see what the maths is doing, then perhaps one can begin to get a better picture of what might be happening physically.
However, there is danger here. This is where science meets philosophy, and interpretation is everything.
Not always in physics do the apparent properties of the maths correspond to how things are physically. The use of complex numbers in classical wave mechanics described above is a good example, but there, at least as I understand it, the use of the extra dimension introduced by i is more like a shortcut to avoid doing harder, more regular maths. In quantum mechanics I don't believe that's the case. The i is a mandatory part of the theory.
Alternatively, sometimes different mathematical models are devised that yield the same results because they are equivalent. In quantum mechanics this already happened, where Matrix Mechanics was used before complex numbers to do exactly the same thing. In this case, what little can be drawn out of looking at the maths in question looks very similar to the complex plane model anyway, but there are other examples in physics where that's not the case. Godel's novel solution to Einstein's field equation is a good example.