Visualizing Arbitrary Coordinate System - Example Needed

[BLevine1985]

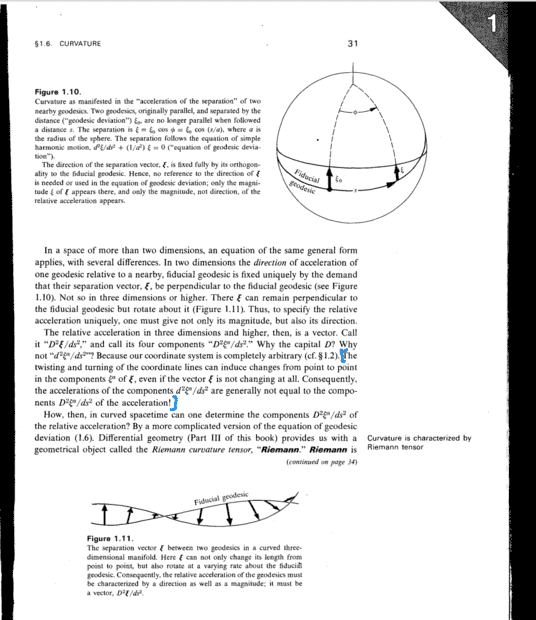
Hi I'm wondering if someone can illustrate with an example what I bracketed in blue? I'm having a hard time visualizing how it is that the accelerations of the components are NOT necessarily equal to the components of the acceleration...Much appreciated!

 

[pasmith]

In plane polars, the position vector is r\mathbf{e}_r(\theta) = r(\cos \theta, \sin \theta). The accelerations of the components are therefore \ddot r and zero. However, the acceleration is <br /> \ddot{\mathbf{r}} = \ddot r\mathbf{e}_r + 2\dot r \dot \theta \mathbf{e}_\theta + r(-\dot \theta^2 \mathbf{e}_r + \ddot \theta \mathbf{e}_\theta) = (\ddot r - r\dot \theta^2)\mathbf{e}_r + (2\dot r \dot \theta + r\ddot \theta)\mathbf{e}_\theta.

 

[BLevine1985]

How did you get the acceleration of the components as r-double-dot and zero?

I understand how you got the general acceleration of r-double dot but not the first part. Sorry it's been like 10 years since I took classical mechanics...