Voltage across a potentiometer?

[gneill]

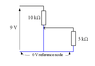
also, wouldn't it work if the slider was at the bottom, which would put the resistors in series, as 5/(5+10) is still a third which is what we want

If the slider is at the bottom of the pot then it will be connected to the 0 V reference node and the output will be 0 V, not the required 3 V. Another way to look at it is that when the slider is at the very bottom of the POT the 5k load resistor will be shorted out.

 

[agreaves]

my working was pretty much the same as the others, just swapping the (1-x)'s and x's around

1/3 = [5*10x/(10x+5)] / [(5*10x/(10x+5) + 10(1-x)]

got this to 1/3 = [50x]/ [5*10x + 10(1-x)(10x+5)]

then to 1/3 = [x]/[-2x²+2x+1] divided through by 50

 

[gneill]

my working was pretty much the same as the others, just swapping the (1-x)'s and x's around

1/3 = [5*10x/(10x+5)] / [(5*10x/(10x+5) + 10(1-x)]

got this to 1/3 = [50x]/ [5*10x + 10(1-x)(10x+5)]

then to 1/3 = [x]/[-2x²+2x+1] divided through by 50

Something went wrong between your first and second lines. While the first line expression is fine and leads to the correct solution for x, the second line does not. So, check your algebra steps in between the two.

 

[agreaves]

yeah, sussed it, 3x - 2x does not equal -x
thanks for the help

 

[Koogle]

Hi, I am also struggling with this one if anyone can point me in the right direction.

This is where I am with it:

3/9 = 10χ5/10χ + 5
-------------------------
10(1-χ) + 10χ5/10χ + 5

I have tried to simplify further:

50χ/10χ+5
------------------------
10χ-10 + 50χ/10χ+5

5/5
--------------
10χ-10 + 5/5

1
-------
10χ-9

I can work out χ but feel that I am not doing this right and would appreciate and help or input.

Thanks for your time

 

[gneill]

Hi, I am also struggling with this one if anyone can point me in the right direction.

This is where I am with it:

3/9 = 10χ5/10χ + 5
-------------------------
10(1-χ) + 10χ5/10χ + 5

I have tried to simplify further:

50χ/10χ+5
------------------------
10χ-10 + 50χ/10χ+5

5/5
--------------
10χ-10 + 5/5

1
-------
10χ-9

I can work out χ but feel that I am not doing this right and would appreciate and help or input.

Thanks for your time

Your problems seem to be with the algebra rather than the physics. For example in your second line, in expanding the 10(1 - x) term in the denominator you wrote 10x - 10. You've negated the signs.

Work through your algebra carefully. Pick a "test" value for x that you can plug in after each "simplification" to check that the overall expression hasn't changed value (not a 100% foolproof test, but it will usually raise the "I did something boneheaded there in that step" flag).

By the way, you may want to investigate the LaTex syntax that's available for rendering math formulas. You can avoid having space compression and proportional fonts mucking up the formatting of your equations by using LaTex. Check out this quick LaTex overview post for more information.

 

[Jayohtwo]

Hi guys,

I have been searching for a way to solve the equation past here and came up short ( no idea why the image is sideways sorry). Is there a rule I'm forgetting about to simplify the last part to determine x?

 

[gneill]

Check your expansions of $10(1 - x) + 5$. There should be a $10 x$ in the result.

There's no secret method, it's just a matter of slogging through the expansions and cancellations, cross multiplications and so forth, to arrive at a quadratic equation in x.

 

[Jayohtwo]

After cross multiplying and simplifying the equation and then using the quadratic formula to get 2 values for x I've ended up with 2 values that use imaginary numbers, clearly this is not correct and I've gone wrong somewhere above. I'm pretty sure I've cross multiplied incorrectly but not obviously not 100% sure at what stage I've messed up. Any help would would be greatly appreciated thanks.