- #1
Albertgauss
Gold Member
- 294
- 37
Good Day
Hi all,
I am doing a project with the standard lab about measuring equipotential surfaces with conductive paper, a voltmeter, and a power supply. I have a couple of questions about this project.
I attach a jpeg of the equations for voltage calculations in the file “Equations.jpeg”. My confusion is centered around making V = 0 as r --> ∞ (as you move away from the charge distributions) for the configurations of this project.
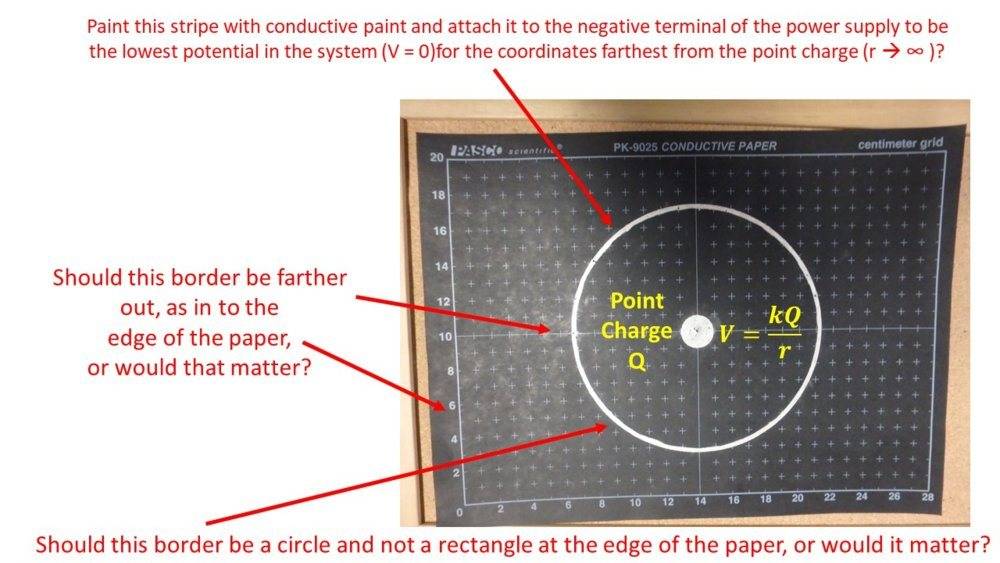
Question 1: In the slides “Dipole.jpeg”, “LineOfCharge.jpeg”, “ParLines.jpeg”, “PointCharge.jpeg”, do I need to paint a conductive stripe (attached to the negative to terminal of the power supply) on the edges of my conductive paper, so that V --> 0 as r --> ∞?
Note that in the jpeg “PointCharge.jpeg”, there is already a silver ring for this purpose. Should I move that ring closer to the edge of the paper? Could I replace the ring with a rectangular border on the edge of the paper? The ring in that paper does not seem far away enough from the point charge for “r --> ∞”.
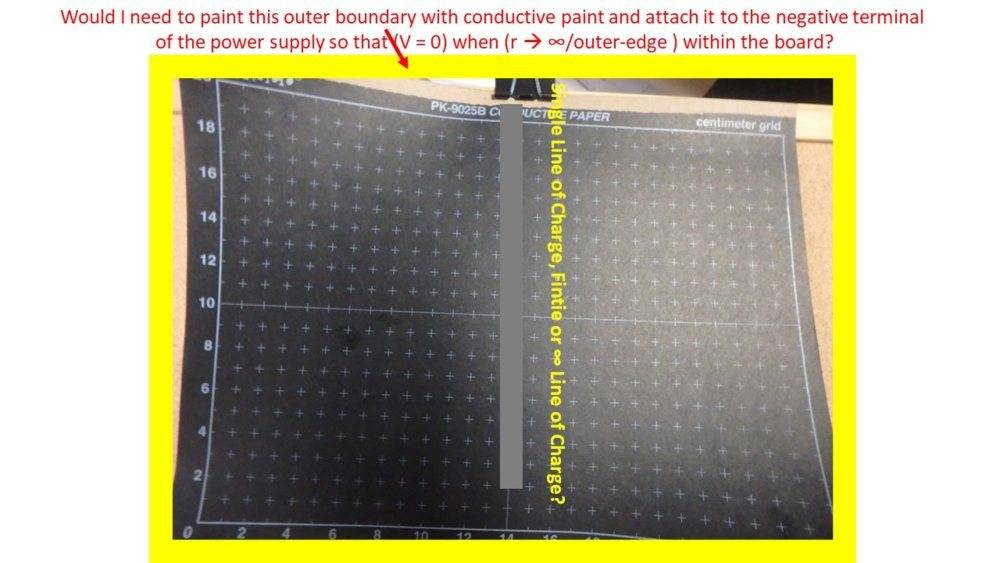
I have not seen any such border on any Parallel lines for this project, yet, though. Usually one line is at the (negative terminal of power supply and COM of voltmeter) and the other line will be at V ~ 20 volts and connected to the positive terminal of the power supply. Many labs just have the bars at opposite ends of the paper and only focus on voltages in between the lines; for those labs with the bars centered in the middle of the paper, no border was ever present.

Question 2. Should I use the expression of the voltage due to a finite wire, even though it is complicated, for a single line of charge (but possibly more realistic)? Or can I use the voltage due to an infinite wire? If the later, do I need a border (yellow) at which the negative terminal of the power supply and COM of the voltmeter would be attached, as in the jpeg “LineOfCharge.jpeg”?
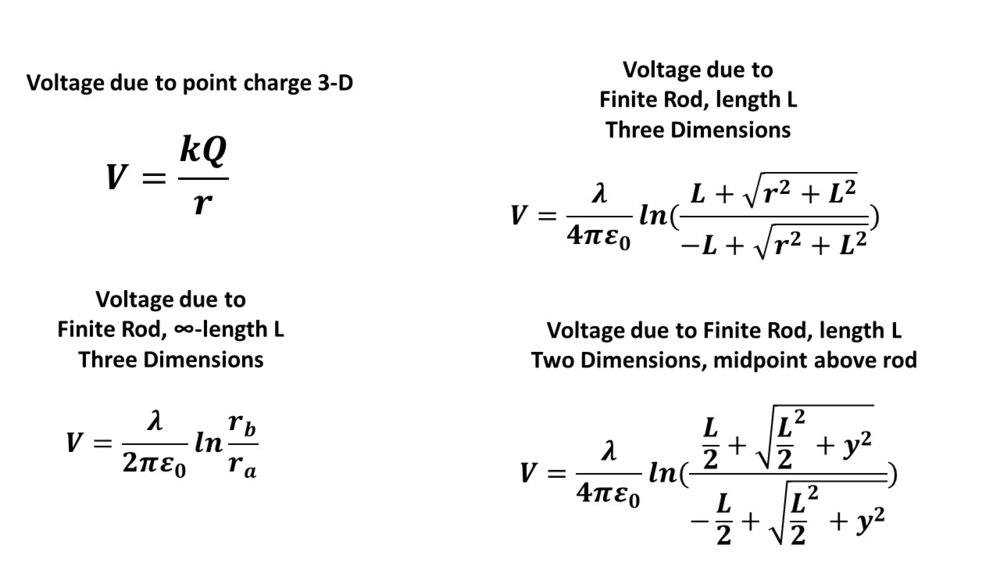
Question 3. I have never seen any calculations for the potential to distinguish between two dimensions or three-dimensions? Does the voltage expression change between two and three dimensions? I have never seen any calculations for the voltage due to these configurations expressly developed for one case or the other but would be glad to know where to find them. My equations above are what I understand about this last question.
Hi all,
I am doing a project with the standard lab about measuring equipotential surfaces with conductive paper, a voltmeter, and a power supply. I have a couple of questions about this project.
I attach a jpeg of the equations for voltage calculations in the file “Equations.jpeg”. My confusion is centered around making V = 0 as r --> ∞ (as you move away from the charge distributions) for the configurations of this project.
Question 1: In the slides “Dipole.jpeg”, “LineOfCharge.jpeg”, “ParLines.jpeg”, “PointCharge.jpeg”, do I need to paint a conductive stripe (attached to the negative to terminal of the power supply) on the edges of my conductive paper, so that V --> 0 as r --> ∞?
Note that in the jpeg “PointCharge.jpeg”, there is already a silver ring for this purpose. Should I move that ring closer to the edge of the paper? Could I replace the ring with a rectangular border on the edge of the paper? The ring in that paper does not seem far away enough from the point charge for “r --> ∞”.
I have not seen any such border on any Parallel lines for this project, yet, though. Usually one line is at the (negative terminal of power supply and COM of voltmeter) and the other line will be at V ~ 20 volts and connected to the positive terminal of the power supply. Many labs just have the bars at opposite ends of the paper and only focus on voltages in between the lines; for those labs with the bars centered in the middle of the paper, no border was ever present.
Question 2. Should I use the expression of the voltage due to a finite wire, even though it is complicated, for a single line of charge (but possibly more realistic)? Or can I use the voltage due to an infinite wire? If the later, do I need a border (yellow) at which the negative terminal of the power supply and COM of the voltmeter would be attached, as in the jpeg “LineOfCharge.jpeg”?
Question 3. I have never seen any calculations for the potential to distinguish between two dimensions or three-dimensions? Does the voltage expression change between two and three dimensions? I have never seen any calculations for the voltage due to these configurations expressly developed for one case or the other but would be glad to know where to find them. My equations above are what I understand about this last question.
