- #1
Korisnik
- 62
- 1
Hello, I don't understand how more loops in the AC generator create bigger voltage. I understand when we talk about magnetic flux how it happens (rate of change of surface that's penetrated by field lines), but there's also the explanation that says "if the magnetic field penetrates the paper, and if the conductor moves to the right, then the force will be upwards", now by force, since it's actually creating a voltage source, (the force acts on positive charges, and the current flows from + to -) is equivalent to conventional current direction.
So the picture is here: http://sciencecity.oupchina.com.hk/npaw/student/glossary/img/slip_rings.jpg
Now if you look at the loops you notice that if the loop that's farthest from the center of rotation actually moves like a tangent to a small circle, so it doesn't rotate, it goes in circles! Now if the loop were in the position that the holes were on the sides and the wires were up and down, then, looking from the north pole side, the lower part of the loop moves upwards, but SO DOES the upper part of the loop (because they aren't in the center of the rotation).
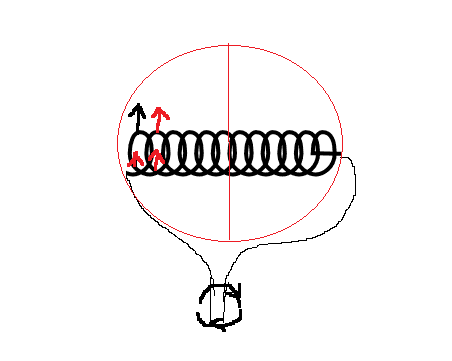
Since both upper and lower parts of the loop move upwards, by the left hand rule I described earlier the current goes in the same direction in upper and lower part of the wire; since the wire of the loop is continuous, the currents (voltages) should interact and annihilate one another. Why isn't this so?
So the picture is here: http://sciencecity.oupchina.com.hk/npaw/student/glossary/img/slip_rings.jpg
Now if you look at the loops you notice that if the loop that's farthest from the center of rotation actually moves like a tangent to a small circle, so it doesn't rotate, it goes in circles! Now if the loop were in the position that the holes were on the sides and the wires were up and down, then, looking from the north pole side, the lower part of the loop moves upwards, but SO DOES the upper part of the loop (because they aren't in the center of the rotation).
Since both upper and lower parts of the loop move upwards, by the left hand rule I described earlier the current goes in the same direction in upper and lower part of the wire; since the wire of the loop is continuous, the currents (voltages) should interact and annihilate one another. Why isn't this so?
Last edited by a moderator: