W0 = 1/RC? Transfer Functions and Bode Plots

[garthenar]

Homework Statement

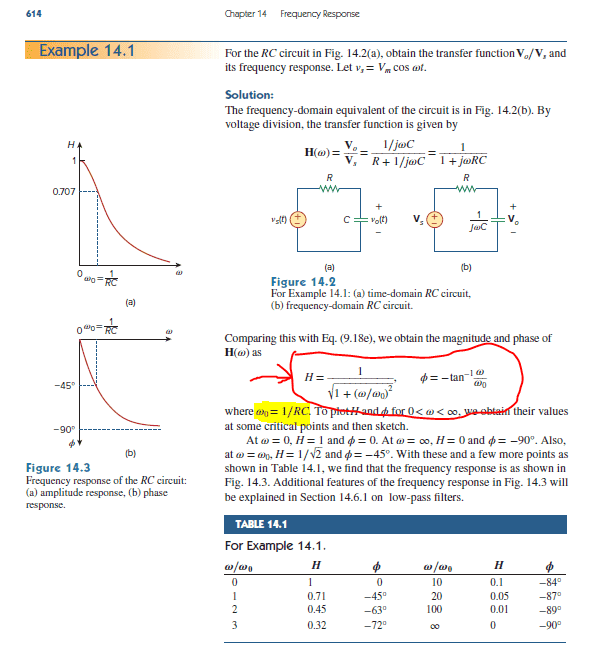
Example 14.1: For the RC circuit in Fig. 14.2(a), obtain the transfer function Vo∕Vs and
its frequency response. Let vs = Vm cos ωt

Relevant Equations

H(w) = Vo / Vin = |H(w)| ∟θ

Algebra/Trig for dealing with the Magnitude and Phase

Here is the example and solution in full. I have circled where I'm at and highlighted the part that's tripping me up.

I managed to get...

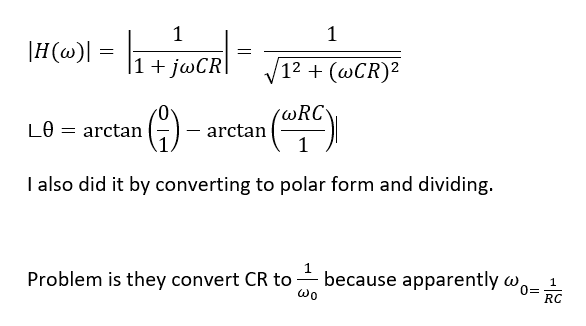
and getting everything in terms of the angular frequency seems to be critical for getting the plots for the Frequency Response.

I checked my notes on RC, LC, and RCL circuits and I can't find this equation. I also checked my book.

I looked on Wikipedia and they seem to be getting this from A function G (gain?) but there's a lot of mention of Filters (Next section) and all the videos on Youtube were talking about Laplace Transforms (Section after next) so I'm having trouble understanding. https://en.wikipedia.org/wiki/RC_circuit#Frequency-domain_considerations

Hope that's not too much. I'm sure it's something simple but It's driving me up a wall.

 

[ammarb32]

In the picture where it says "Comparing this with Eq (9.18e)", could you post a picture showing what Eq (9.18e) is referring to? (screenshot below):

 

[garthenar]

In the picture where it says "Comparing this with Eq (9.18e)", could you post a picture showing what Eq (9.18e) is referring to? (screenshot below):
...

Sure. Here you go.

I've been doing more research and I'm starting to think this has something to do with cutoff frequency. I'm going to look at getting the pols and checking it against that.

 

[Joshy]

Are you confused as to how they got to the $\omega_0 = 1/CR$? You could copy from your own results (hopefully that's your work in the image below the textbook screenshot) by setting $\omega/\omega_0 = \omega CR$ it's from your own solution... solve for $\omega_0$... I think you'll be very surprised at the result ;)

If you're confused about why they chose that form putting with $1+\omega/\omega_0$ in the denominator what happens to the magnitude when $\omega = \omega_0$? The graph in figure 14.3a it gives you a hint particularly at a some numbers along the y-axis it's a very common and special number.

 

[garthenar]

Are you confused as to how they got to the $\omega_0 = 1/CR$?...

That is exactly what is confusing me.

Looking at it, if I set $\omega =$\omega_0 then the magnitude becomes 1/√2 which I just read is called the cutoff? (Brand new to this and LPFs are two sections from now). Did they chose RC = 1/##\omega_0 to achieve this number?

 

[Joshy]

Correct! When you set $\omega = \omega_0$ it becomes $1/\sqrt{2}$. After the frequency $\omega$ passes $\omega_0$ the magnitude of the transfer function $H(\omega)$ starts decreasing a lot approaching a very small number near zero. Notice prior to $\omega_0$ where $\omega << \omega_0$ it's much smaller, then the magnitude is still pretty close to $1/1$.

You could copy from your own results (hopefully that's your work in the image below the textbook screenshot) by setting $\omega/\omega_0 = \omega CR$ it's from your own solution... solve for $\omega_0$... I think you'll be very surprised at the result ;)

So how to find $\omega_0$ for your circuit?

Copy it from your own equation right underneath where you said "I managed to get" and you have some equation for $|H(\omega)|$.

Do you see from the textbook that it has an equation (the one you circled in red) that looks suspiciously like your equation? Here's what they have:
$$\sqrt{ \frac{1}{1 + \left ( \frac{\omega}{\omega_0} \right )^2} }$$
Here's what you have
$$\sqrt{ \frac{1}{1 + (\omega RC)^2} }$$
You could set your equation to equal $1/\sqrt{2}$ too, but... that little difference (on the bottom right of the equations) why don't you go ahead and set that part to be the same in both equations. Set $(\omega/\omega_0)^2 = (\omega RC)^2$ and solve for $\omega_0$.

Are you feeling better about this? The homework section they don't allow us to just give answers...

 

[garthenar]

Are you feeling better about this? The homework section they don't allow us to just give answers...

I'm definitely feeling better about this. I still have a lot to understand but I'm kind of ticked that they used concepts from later in the book in the practice problem. I'm just glad it wasn't something I had already learned.

And thank you. I know they don't allow people to just give answers but getting hints and having more experienced people to tell you when you've found a sound answer helps a lot.

 

[hutchphd]

Perhaps I am misunderstanding the question, but let me add this: The choice of $\omega_0\equiv 1/RC$ is esthetic. It makes the equation pretty and defines a frequency that clearly has some fundamental importance in the algebra. Further analysis shows this to be true, but it is just a computational tool (dictated by the problem) that we choose to use.

 

[LvW]

Perhaps I am misunderstanding the question, but let me add this: The choice of $\omega_0\equiv 1/RC$ is esthetic. It makes the equation pretty and defines a frequency that clearly has some fundamental importance in the algebra. Further analysis shows this to be true, but it is just a computational tool (dictated by the problem) that we choose to use.

I rather think that there are some other (and good) reason to define the cut-off frequency - not only "esthetic" reasons.
Up to now, we spoke only on first-order lowpass filters. In this context it is inportant to know that for filter transfer functions it is quite normal to use the compex frequency notation "s=sigma+jw" instead of "jw".
This allows us to find a good and logical systematic for ALL filters of ALL orders with the help of some new definitions: (1) Pole position in the complex s-plane, (2) pole frequency wp and (3) pole-quality factor Qp (important to discriminate between different second-order responses).
Applying this to the first-order lowpass means:

Transfer function H(s)=1/(1+sRC). (For plotting magnitude and phase we return to s=jw).

Now - setting the denominator (1+sRC)=0 defines the pole at sp=-1/RC. So we have a pole on the negative-real axis at "-1/RC". The so called "pole frequency" is defined as the magnitude of the pointer to this pole: wp=1/RC.

THIS POLE FREQUENCY wp IS DEFINED AS THE END OF THE PASSBAND (CUT-OFF).

And it turns out that the magnitude of the transfer function at w=wp is 1/SQRT(2) and the phase shft is -45 deg. But this is only the CONSEQUENCE of the definition.
Note that this definition (cut-off at the pole frequency) is applicable also to second-order filters with BUTTERWORTH characteristics. For all other characteristcs (Chebyshev, Cauer, Bessel,...) the pole frequency again plays the dominant role for defining the individual cut-off frequencies.

 

[hutchphd]

In no way did I mean this as a derogatory term. The exact definition is somewhat arbitrary but the choice to define it is certainly not capricious. As you detail (and as in fact I described it) it is of fundamental importance to the the analysis using the myriad methods.
But in the flow of analyzing the equation, it is largely an esthetic choice...I believe this was one of the issues worrying the OP.