- #1
Happiness
- 695
- 31
In obtaining (5.362) from (5.359), we first get
##U_{\tau}i\hbar\frac{\partial}{\partial t}\Psi(t) = U_{\tau}H^*\Psi^*(-t)##
In order to obtain the LHS of (5.362), ##U_{\tau}## must commute with ##\frac{\partial}{\partial t}##. But how do we know that they commute?
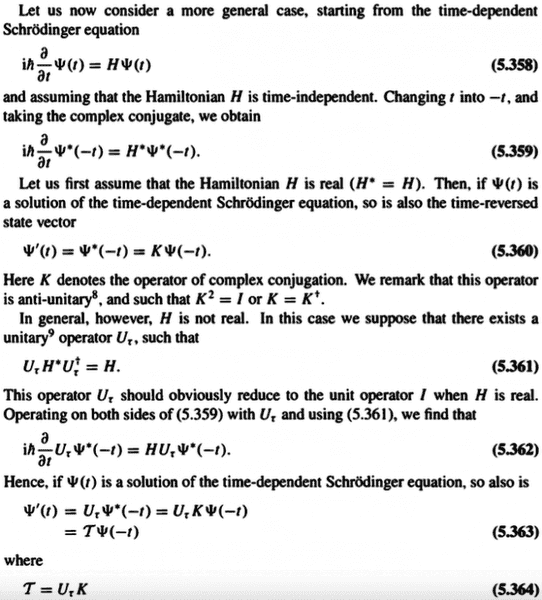
##U_{\tau}i\hbar\frac{\partial}{\partial t}\Psi(t) = U_{\tau}H^*\Psi^*(-t)##
In order to obtain the LHS of (5.362), ##U_{\tau}## must commute with ##\frac{\partial}{\partial t}##. But how do we know that they commute?