- #1
mingyz0403
- 12
- 1
- Homework Statement
- Block A supports a pipe column and rests as shown on wedge B. the coefficient of static friction at all surfaces of contact is 0.25. if p = 0, determine (a) the angle θ for which sliding is impending, (b) the corresponding force exerted on the block by the vertical wall.
- Relevant Equations
- Free body diagram
The correct angle is 28.1 degree.I understand ∅=arctan(m). Isn’t R should be angled ∅ degree away from the normal force.
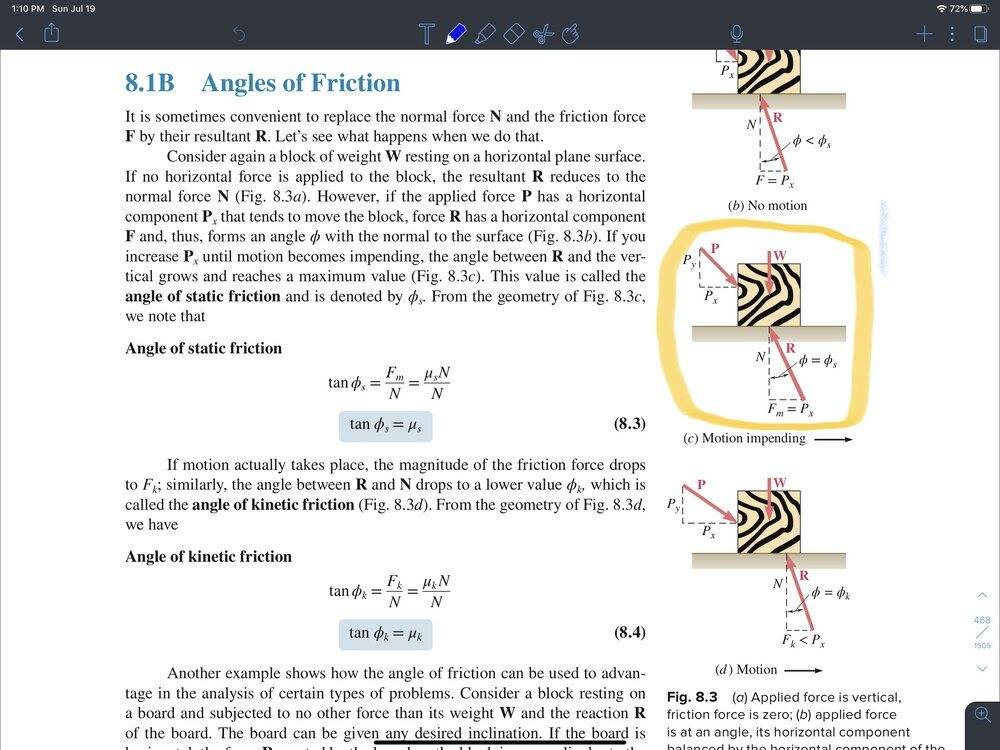
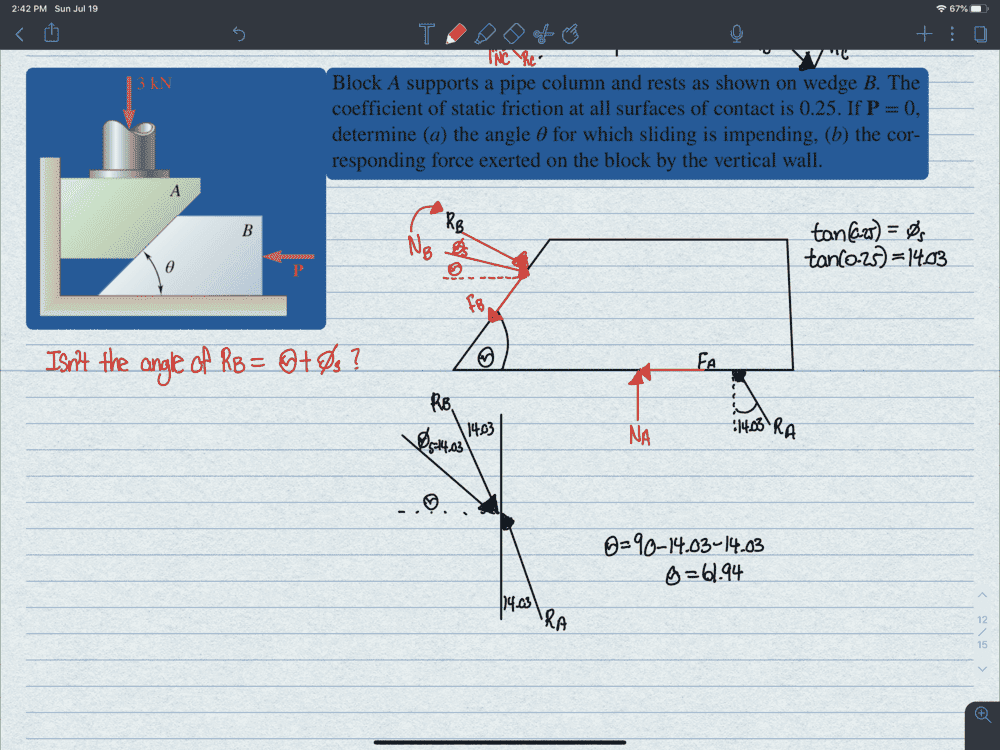
Last edited: